Global Controllability for Quasilinear Non-negative Definite System of ODEs and SDEs

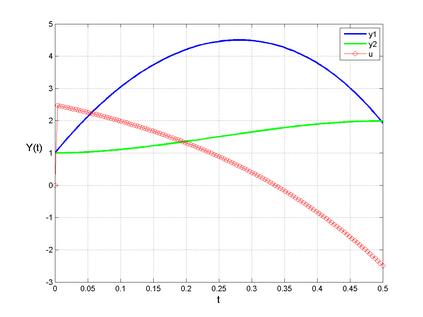
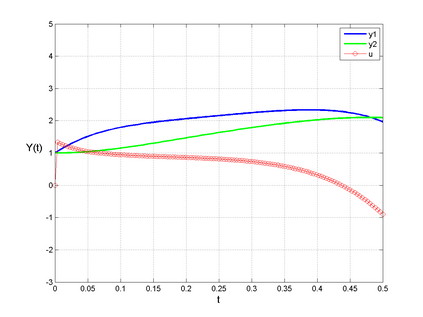
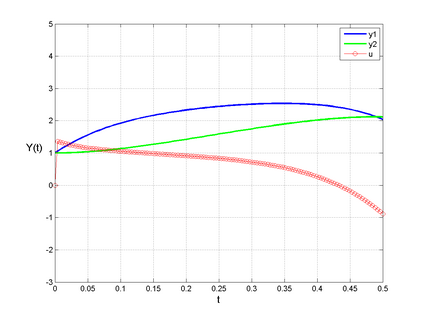
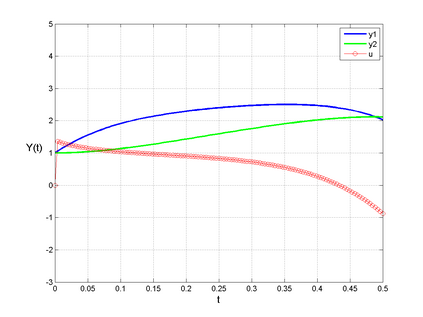
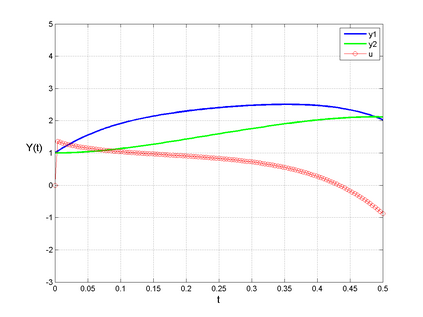
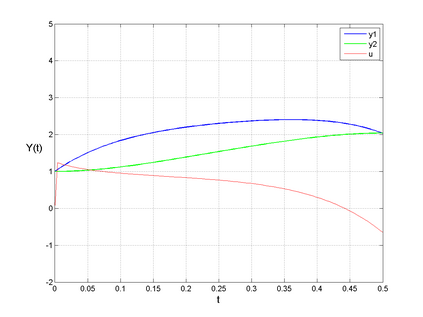
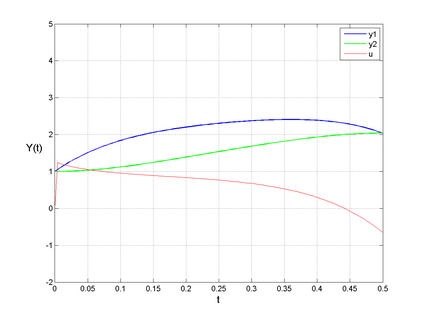
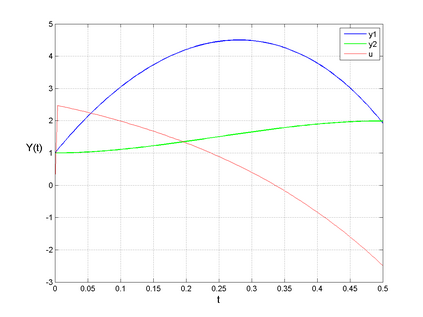
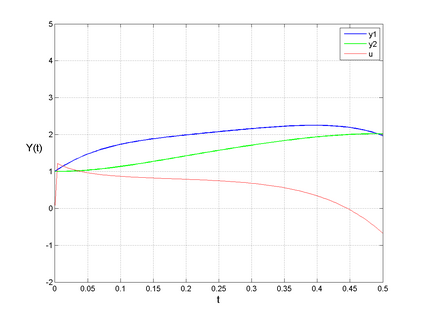
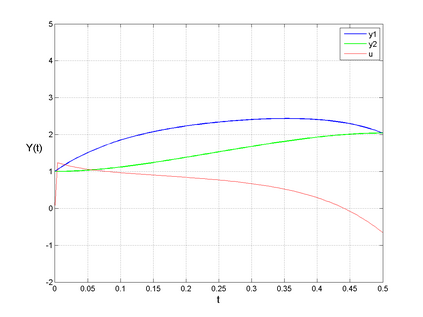
We consider exact and averaged control problem for a system of quasi-linear ODEs and SDEs with a non-negative definite symmetric matrix of the system. The strategy of the proof is the standard linearization of the system by fixing the function appearing in the nonlinear part of the system, and then applying the Leray-Schauder fixed point theorem. We shall also need the continuous induction arguments to prolong the control to the final state which is a novel approach in the field. This enables us to obtain controllability for arbitrarily large initial data (so called global controllability).