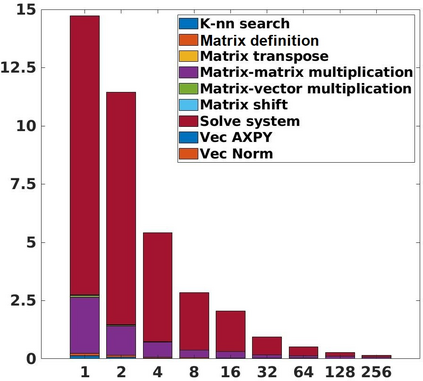
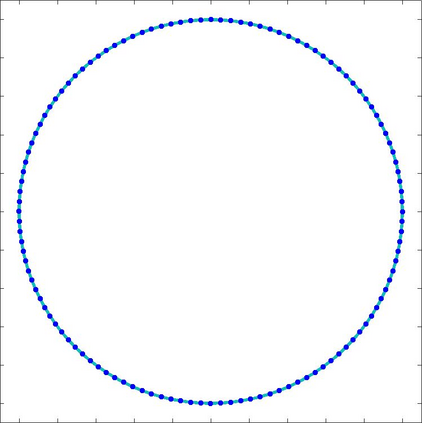
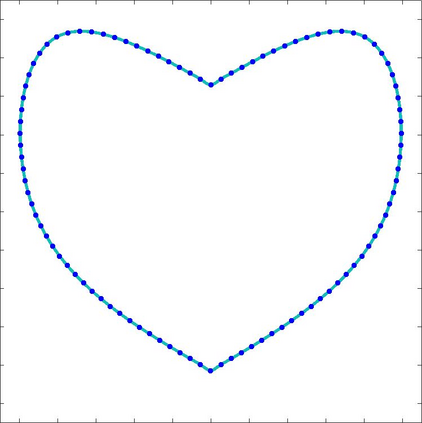
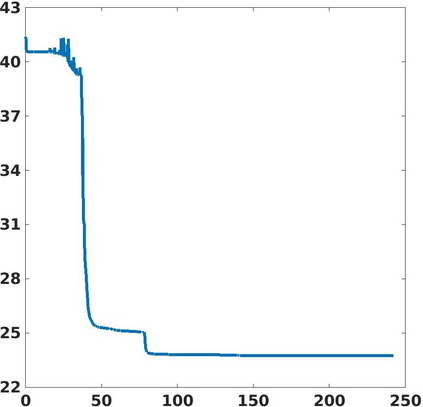
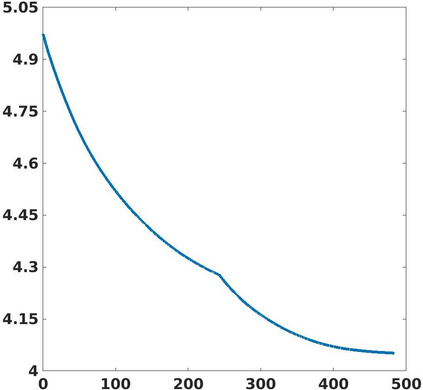
Several physics and engineering applications involve the solution of a minimisation problem to compute an approximation of the input signal. Modern computing hardware and software apply high-performance computing to solve and considerably reduce the execution time. We compare and analyse different minimisation methods in terms of functional computation, convergence, execution time, and scalability properties, for the solution of two minimisation problems (i.e., approximation and denoising) with different constraints that involve computationally expensive operations. These problems are attractive due to their numerical and analytical properties, and our general analysis can be extended to most signal-processing problems. We perform our tests on the Cineca Marconi100 cluster, at the 26th position in the top500 list. Our experimental results show that PRAXIS is the best optimiser in terms of minima computation: the efficiency of the approximation is 38% with 256 processes, while the denoising has 46% with 32 processes.
翻译:暂无翻译