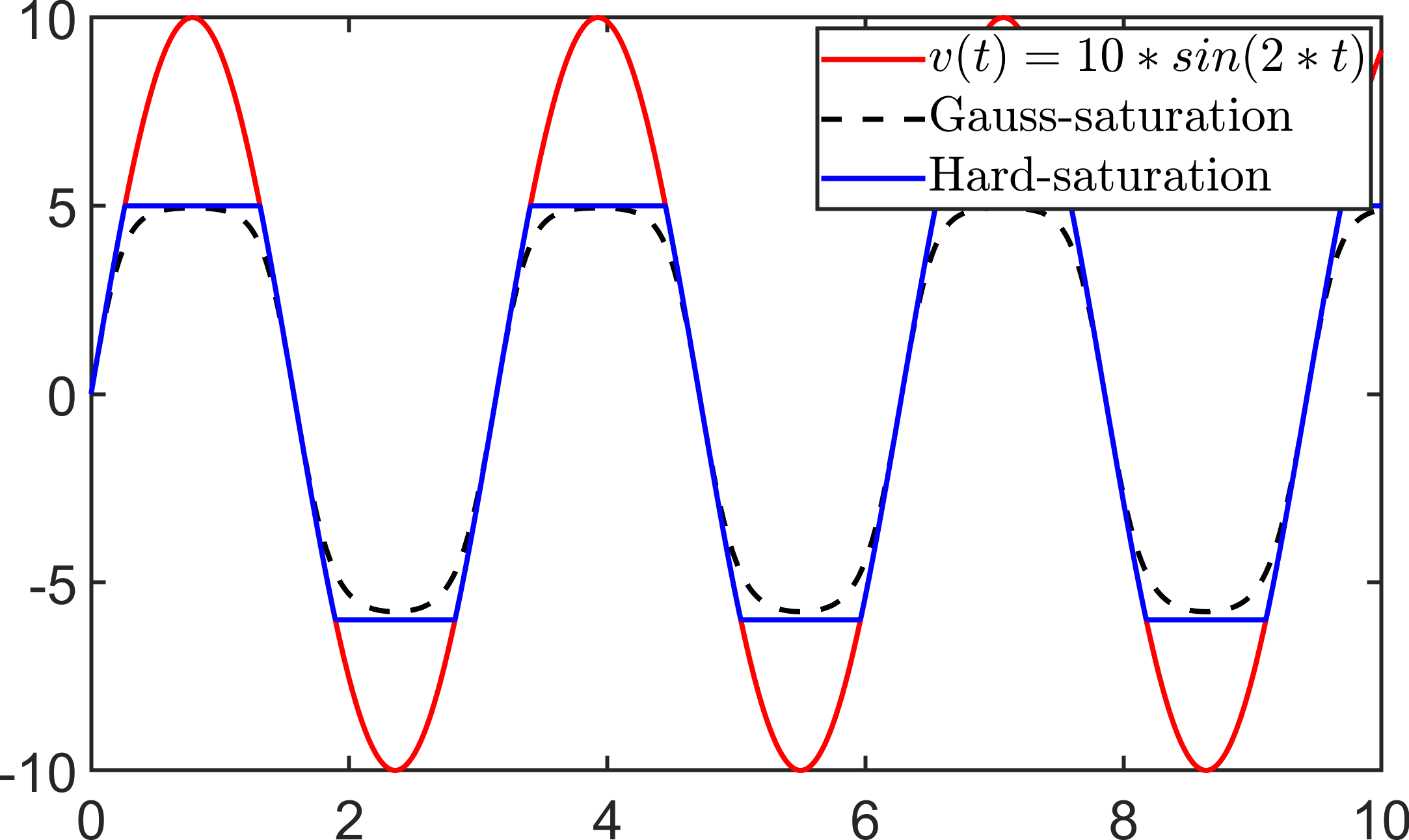
This paper designs a servo control system based on sliding mode control for the shape control of elastic objects. In order to solve the effect of non-smooth and asymmetric control saturation, a Gaussian-based continuous differentiable asymmetric saturation function is used for this goal. The proposed detection approach runs in a highly real-time manner. Meanwhile, this paper uses sliding mode control to prove that the estimation stability of the deformation Jacobian matrix and the system stability of the controller are combined, which verifies the control stability of the closed-loop system including estimation. Besides, an integral sliding mode function is designed to avoid the need for second-order derivatives of variables, which enhances the robustness of the system in actual situations. Finally, the Lyapunov theory is used to prove the consistent final boundedness of all variables of the system.
翻译:暂无翻译