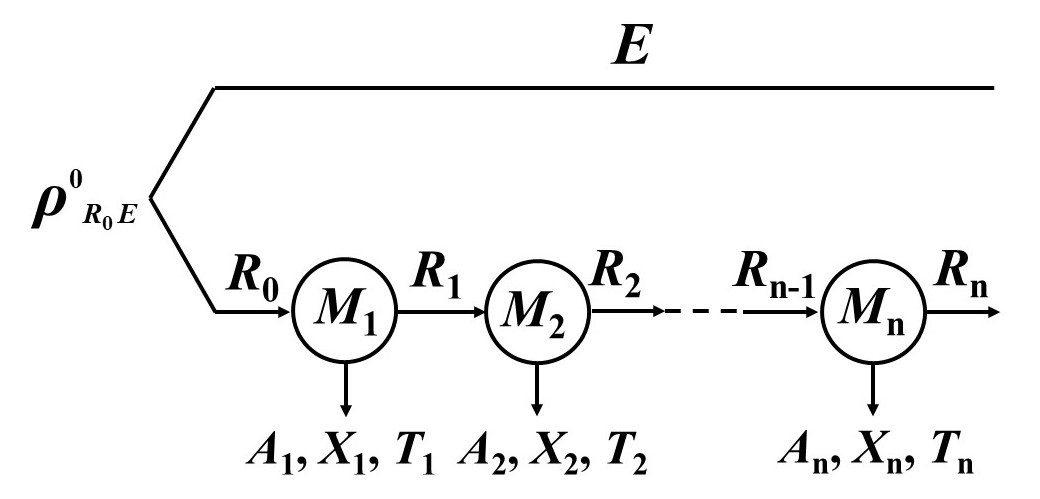
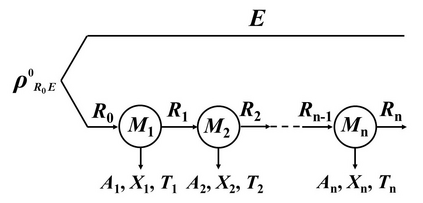
We establish a one-shot strong converse bound for privacy amplification against quantum side information using trace distance as a security criterion. This strong converse bound implies that in the independent and identical scenario, the trace distance exponentially converges to one in every finite blocklength when the rate of the extracted randomness exceeds the quantum conditional entropy. The established one-shot bound has an application to bounding the information leakage of classical-quantum wiretap channel coding and private communication over quantum channels. That is, the trace distance between Alice and Eavesdropper's joint state and its decoupled state vanishes as the rate of randomness used in hashing exceeds the quantum mutual information. On the other hand, the trace distance converges to one when the rate is below the quantum mutual information, resulting in an exponential strong converse. Our result also leads to an exponential strong converse for entropy accumulation, which complements a recent result by Dupuis [arXiv:2105.05342]. Lastly, our result and its applications apply to the moderate deviation regime. Namely, we characterize the asymptotic behaviors of the trace distances when the associated rates approach the fundamental thresholds with speeds slower than $O(1/\sqrt{n})$.
翻译:我们用微距离作为安全标准,针对量子侧信息建立一发强烈的私隐放大线。 这种强烈的反向约束意味着,在独立和相同的假设中,当抽取随机率超过量子有条件英特质时,痕量距离会指数指数化为每个有限区长。 已经建立的一发捆绑可以将古典- 量子窃听器频道编码和私人通信在量子频道上的信息渗漏捆绑起来。 也就是说, Alice 和 Eavesdroper 联合状态之间的微距离及其分解状态会消失, 其分解状态会随着在集散中使用的随机率超过量子相互信息的速度而消失。 另一方面,当提取速率低于量子共同信息时,痕量距离会指数化为一个区段。 我们的结果还导致对矩形积累的指数强烈的反向,这补充了Dupiis [ariv: 2105. 0531/] (最后,我们的结果及其应用适用于中度偏差制度。 也就是说, 当基本距离时,我们将追踪速度与最低速度相比的速度看。