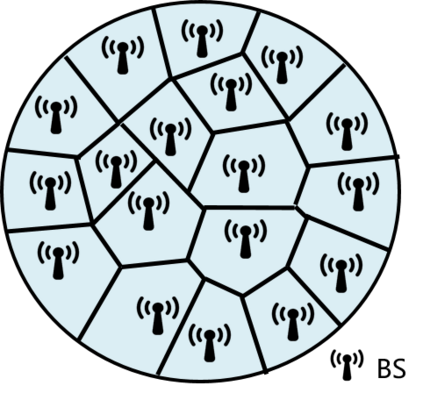
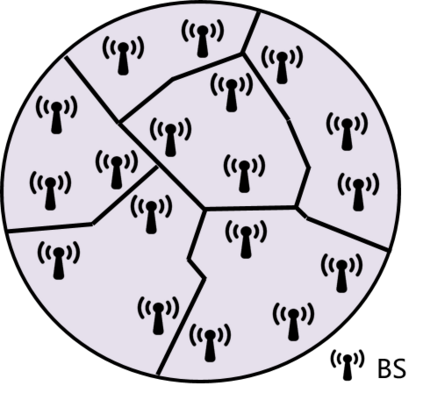
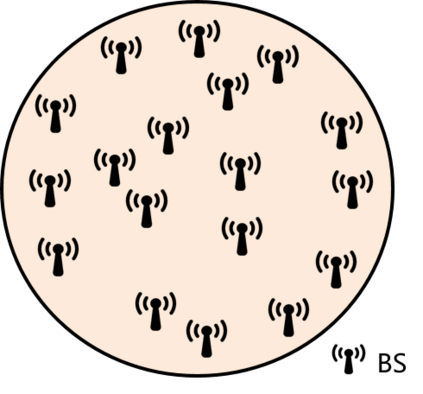
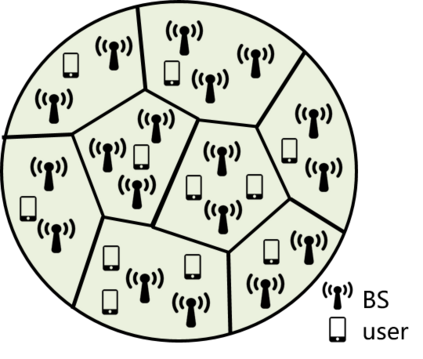
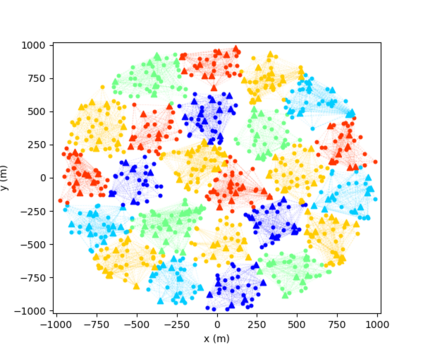
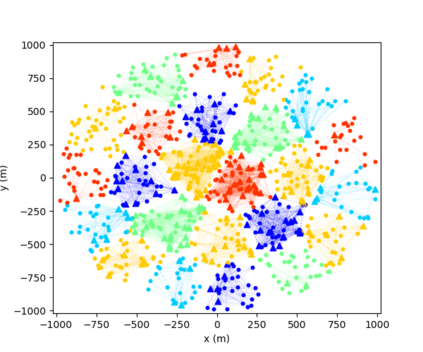
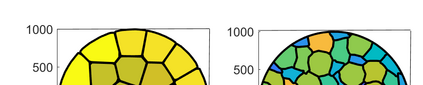
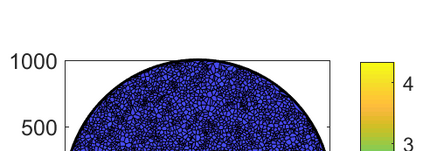
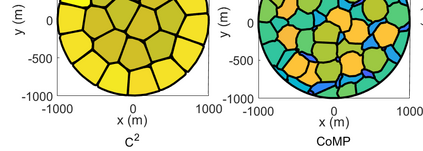
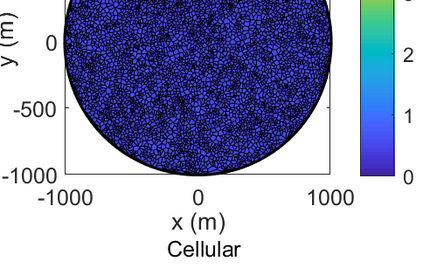
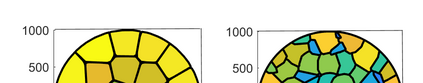
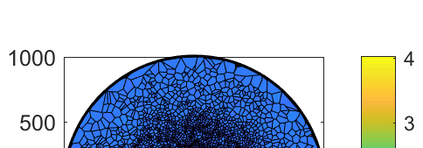
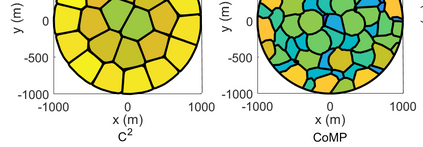
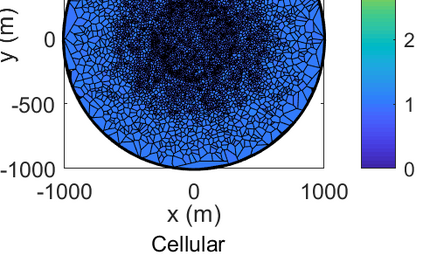
The accelerated convergence of digital and real-world lifestyles has imposed unprecedented demands on today's wireless network architectures, as it is highly desirable for such architectures to support wireless devices everywhere with high capacity and minimal signaling overhead. Conventional architectures, such as cellular architectures, are not able to satisfy these requirements simultaneously, and are thus no longer suitable for the future era. In this paper, we propose a capacity-centric (C$^2$) architecture for future wireless communication networks. It is designed based on the principles of maximizing the number of non-overlapping clusters with the average cluster capacity guaranteed to be higher than a certain threshold, and thus provides a flexible way to balance the capacity requirement against the signaling overhead. Our analytical results reveal that C$^2$ has superior generality, wherein both the cellular and the fully coordinated architectures can be viewed as its extreme cases. Simulation results show that the average capacity of C$^2$ is at least three times higher compared to that of the cellular architecture. More importantly, different from the widely adopted conventional wisdom that base-station distributions dominate architecture designs, we find that the C$^2$ architecture is independent of base-station distributions, and instead user-side information should be the focus in future wireless network architecture designs.
翻译:数字和现实世界生活方式的加速融合对今天的无线网络结构提出了前所未有的要求,因为这些结构非常理想地支持无线装置,其容量高,信号量最小; 细胞结构等常规结构无法同时满足这些要求,因此不再适合未来时代; 在本文件中,我们为未来的无线通信网络提出了一个以能力为中心的(C$2美元)结构; 其设计所依据的原则是最大限度地增加不重叠的集群数量,保证平均集群能力高于某一阈值,从而提供一个灵活的方式,平衡能力需求与信号管理之间的平衡。 我们的分析结果表明,C$2美元结构具有较高的通用性,其中手机和完全协调的结构都可被视为其极端情况。 模拟结果显示,与手机结构相比,C$2美元的平均能力至少高出三倍。 更重要的是,与普遍采用的传统智慧相比,基础站分配控制着建筑设计,我们发现C$2美元结构应该独立于未来网络的用户结构,而不是数据库的用户结构分配重点。