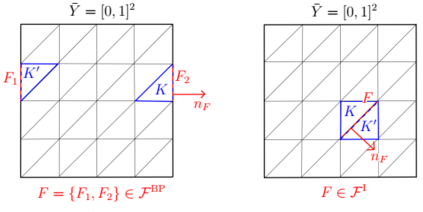
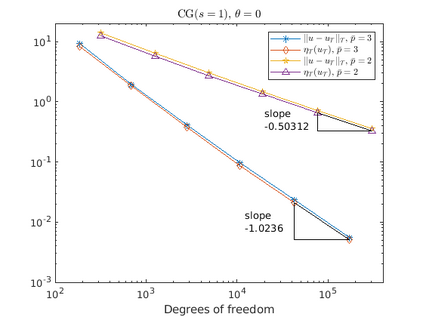
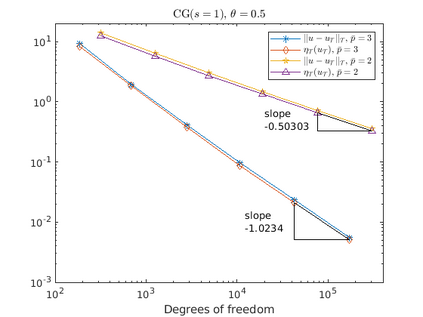
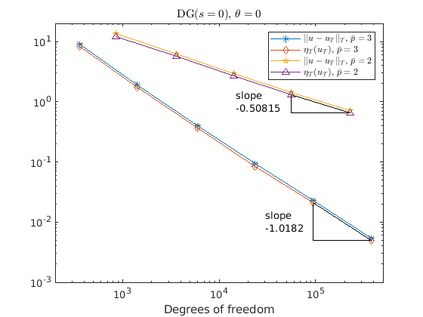
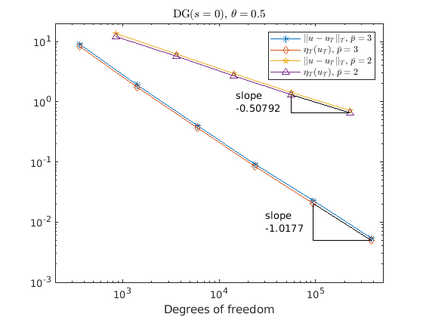
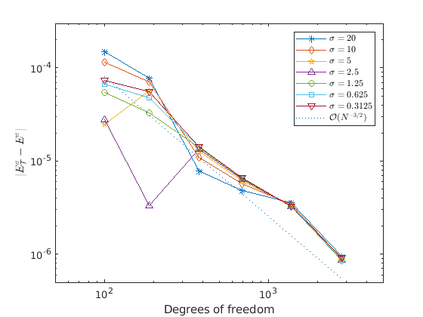
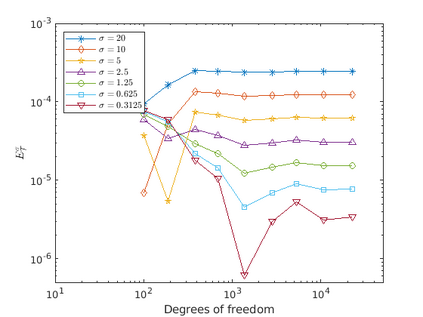
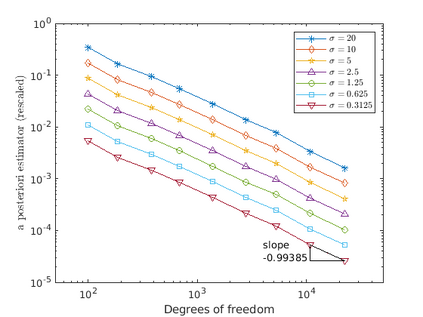
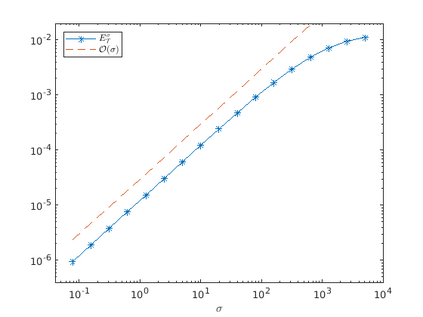
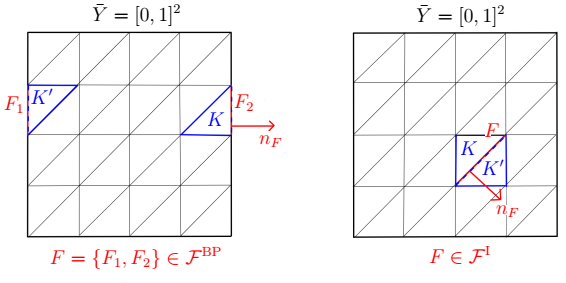
In the first part of the paper, we study the discontinuous Galerkin (DG) and $C^0$ interior penalty ($C^0$-IP) finite element approximation of the periodic strong solution to the fully nonlinear second-order Hamilton--Jacobi--Bellman--Isaacs (HJBI) equation with coefficients satisfying the Cordes condition. We prove well-posedness and perform abstract a posteriori and a priori analyses which apply to a wide family of numerical schemes. These periodic problems arise as the corrector problems in the homogenization of HJBI equations. The second part of the paper focuses on the numerical approximation to the effective Hamiltonian of ergodic HJBI operators via DG/$C^0$-IP finite element approximations to approximate corrector problems. Finally, we provide numerical experiments demonstrating the performance of the numerical schemes.
翻译:在文件第一部分,我们研究了不连续的Galerkin(DG)和$C$0美元内部罚款(C$0美元-IP)的有限要素,这是对完全非线性二级汉密尔顿-Jacobi-Bellman-Isaacs(HJBI)等式及其符合Cordes条件的系数的定期有力解决办法的定期有效近似值,我们证明它具有很好的影响力,并进行了抽象的后生分析,以及适用于一个广大数字体系的先验分析。这些周期性问题是HJBI等式同化的正确性问题。文件第二部分的重点是通过DG/$C$0美元-IP的有限要素近似值,使Ergodic HJBI操作员的汉密尔顿式有效接近值,以近似于正确的问题。最后,我们提供了数字实验,以证明数字体系的性能。