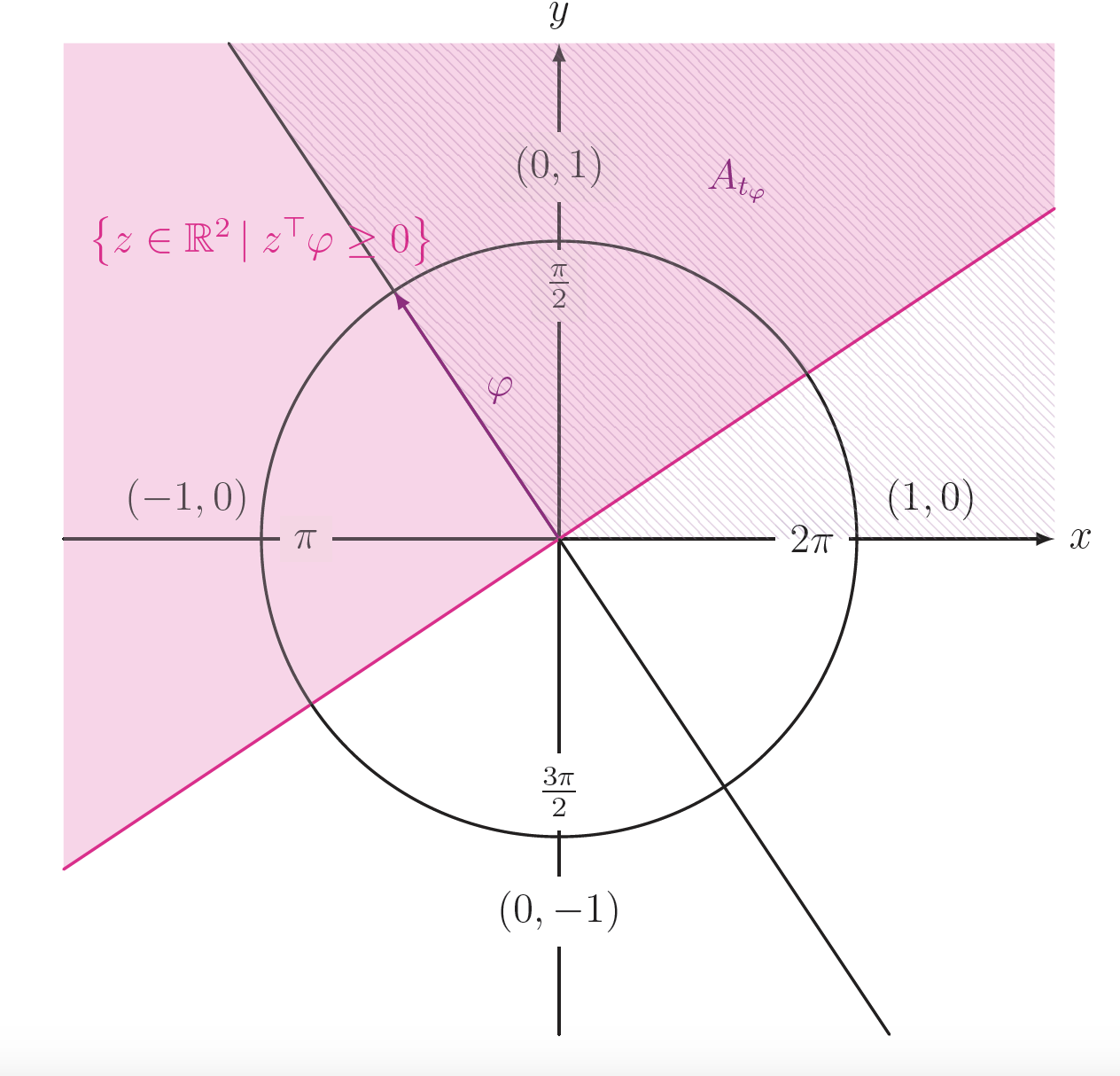
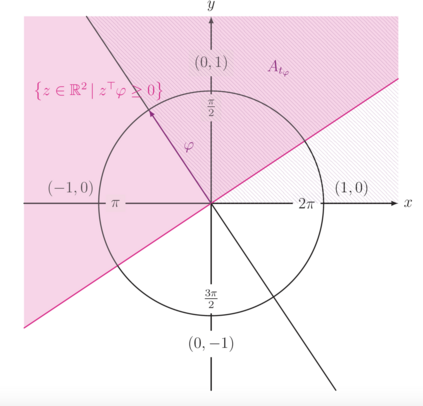
We establish a definition of ordinal patterns for multivariate time series data based on the concept of Tukey's halfspace depth. Given the definition of these \emph{depth patterns}, we are interested in the probabilities of observing specific patterns in a time series. For this, we consider the relative frequency of depth patterns as natural estimators for their occurrence probabilities. Depending on the choice of reference distribution and the relation between reference and data distribution, we distinguish different settings that are considered separately. Within these settings we study statistical properties of ordinal pattern probabilities, establishing consistency and asymptotic normality under the assumption of weakly dependent time series data. Since our concept only depends on ordinal depth information, the resulting values are robust under small perturbations and measurement errors.
翻译:暂无翻译