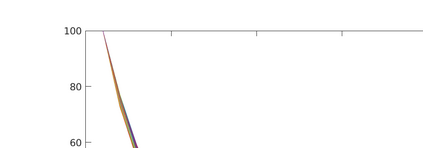
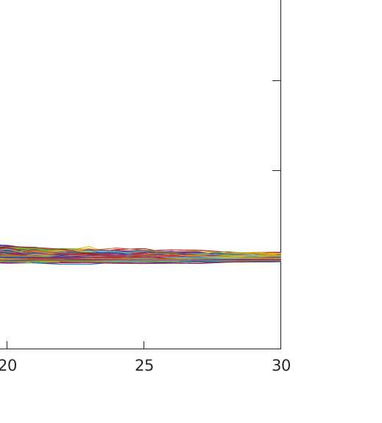
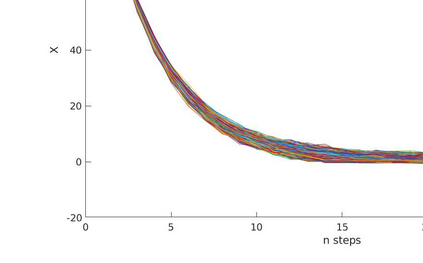
In this paper we investigate explicit numerical approximations for stochastic differential delay equations (SDDEs) under a local Lipschitz condition by employing the adaptive Euler-Maruyama (EM) method. Working in both finite and infinite horizons, we achieve strong convergence results by showing the boundedness of the pth moments of the adaptive EM solution. We also obtain the order of convergence infinite horizon. In addition, we show almost sure exponential stability of the adaptive approximate solution for both SDEs and SDDEs.
翻译:在本文中,我们通过使用适应性欧拉-马鲁山(EM)方法,调查当地利普西茨条件下的随机差分延迟方程式(SDDEs)的明显数字近似值。在有限和无限的视野中,我们通过显示适应性EM解决方案的微秒的界限,取得了强烈的趋同结果。我们还获得了趋同无限的地平线。此外,我们几乎可以肯定地显示SDEs和SDDEs的适应性近似解决方案的指数稳定性。
相关内容
专知会员服务
77+阅读 · 2020年2月8日
专知会员服务
36+阅读 · 2019年10月17日