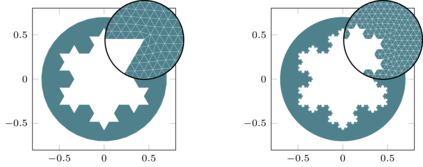
This paper is concerned with the numerical computation of scattering resonances of the Laplacian for Dirichlet obstacles with rough boundary. We prove that under mild geometric assumptions on the obstacle there exists an algorithm whose output is guaranteed to converge to the set of resonances of the problem. The result is formulated using the framework of Solvability Complexity Indices. The proof is constructive and provides an efficient numerical method. The algorithm is based on a combination of a Glazman decomposition, a polygonal approximation of the obstacle and a finite element method. Our result applies in particular to obstacles with fractal boundary, such as the Koch Snowflake and certain filled Julia sets. Finally, we provide numerical experiments in MATLAB for a range of interesting obstacle domains.
翻译:暂无翻译