A non-parametric approach to detect patterns in binary sequences

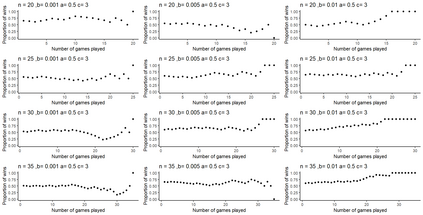
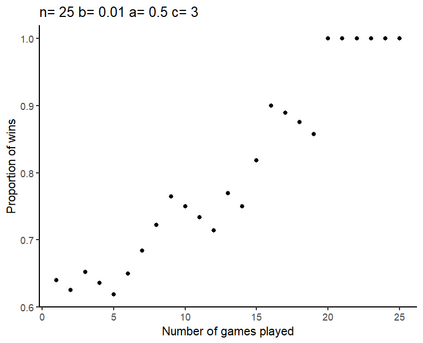
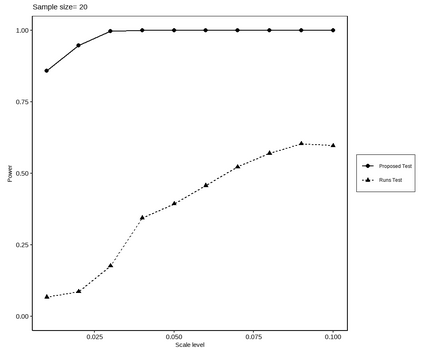
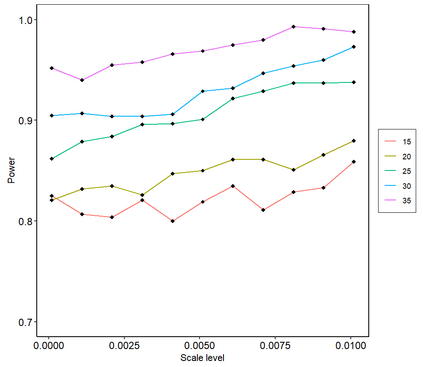
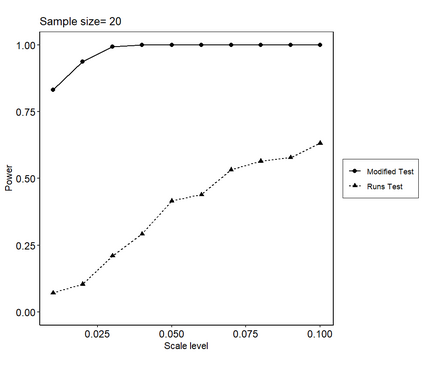
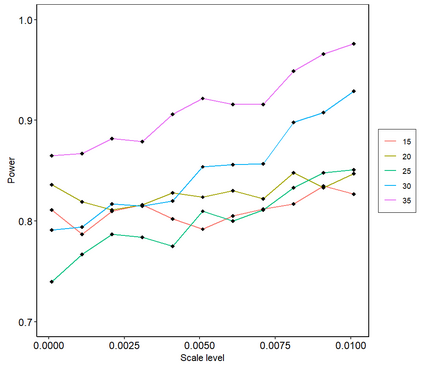
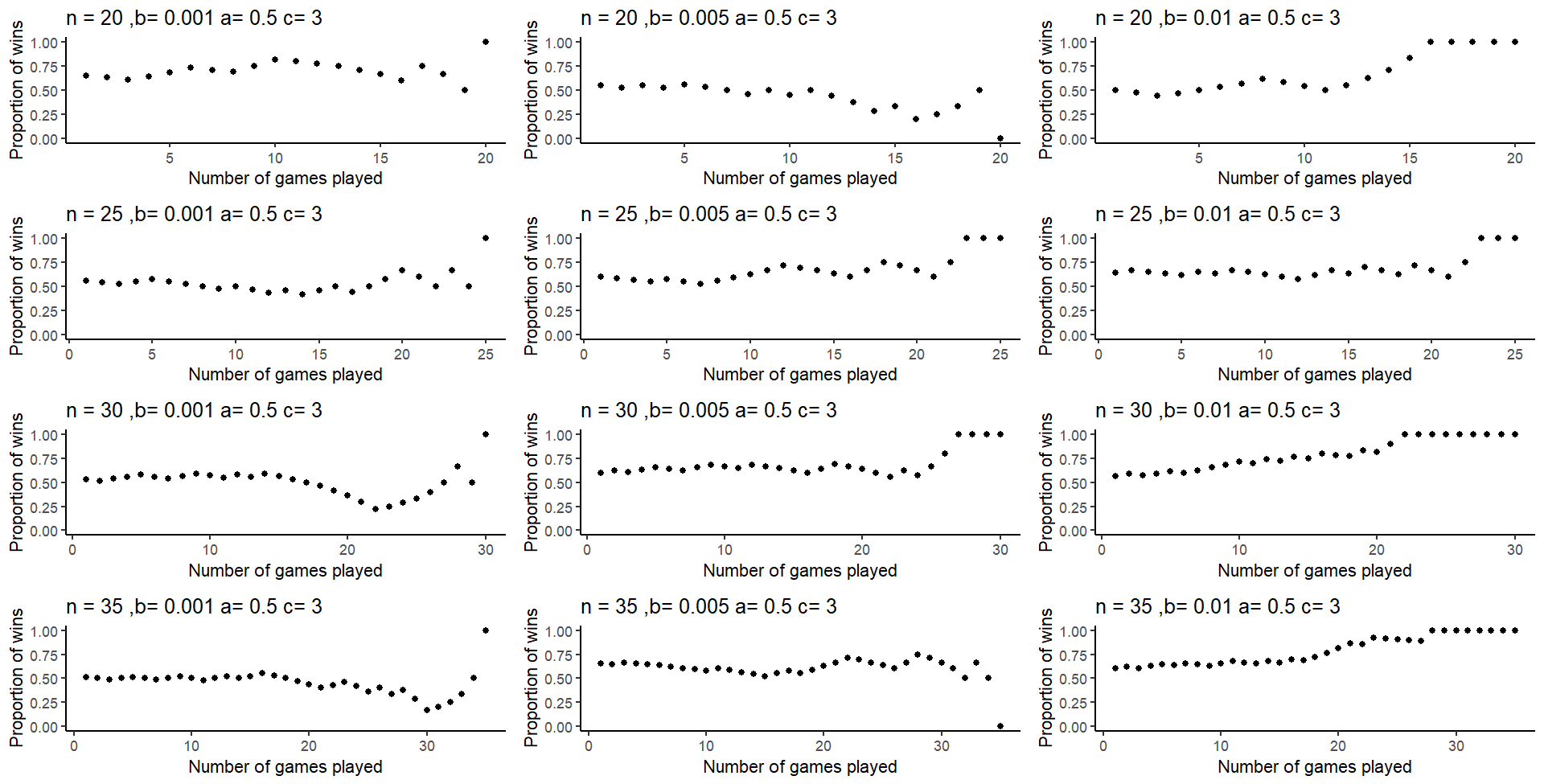
In many circumstances given an ordered sequence of one or more types of elements/ symbols, the objective is to determine any existence of randomness in occurrence of one of the elements,say type 1 element. Such a method can be useful in determining existence of any non-random pattern in the wins or loses of a player in a series of games played. Existing methods of tests based on total number of runs or tests based on length of longest run (Mosteller (1941)) can be used for testing the null hypothesis of randomness in the entire sequence, and not a specific type of element. Additionally, the Runs Test tends to show results contradictory to the intuition visualised by the graphs of say, win proportions over time due to method used in computation of runs. This paper develops a test approach to address this problem by computing the gaps between two consecutive type 1 elements and thereafter following the idea of "pattern" in occurrence and "directional" trend (increasing, decreasing or constant), employs the use of exact Binomial test, Kenall's Tau and Siegel-Tukey test for scale problem. Further modifications suggested by Jan Vegelius(1982) have been applied in the Siegel Tukey test to adjust for tied ranks and achieve more accurate results. This approach is distribution-free and suitable for small sizes. Also comparisons with the conventional runs test shows the superiority of the proposed approach under the null hypothesis of randomness in the occurrence of type 1 elements.