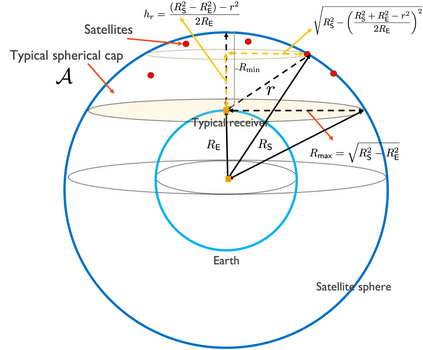
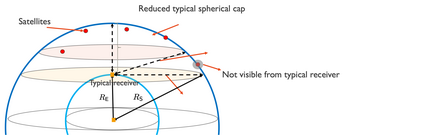
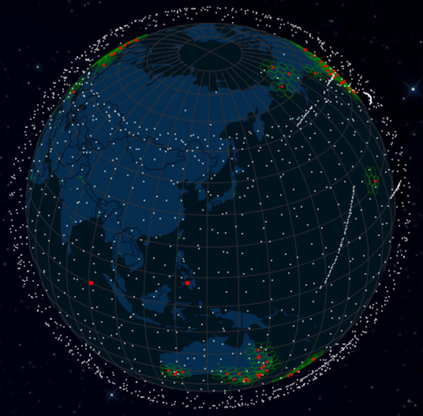
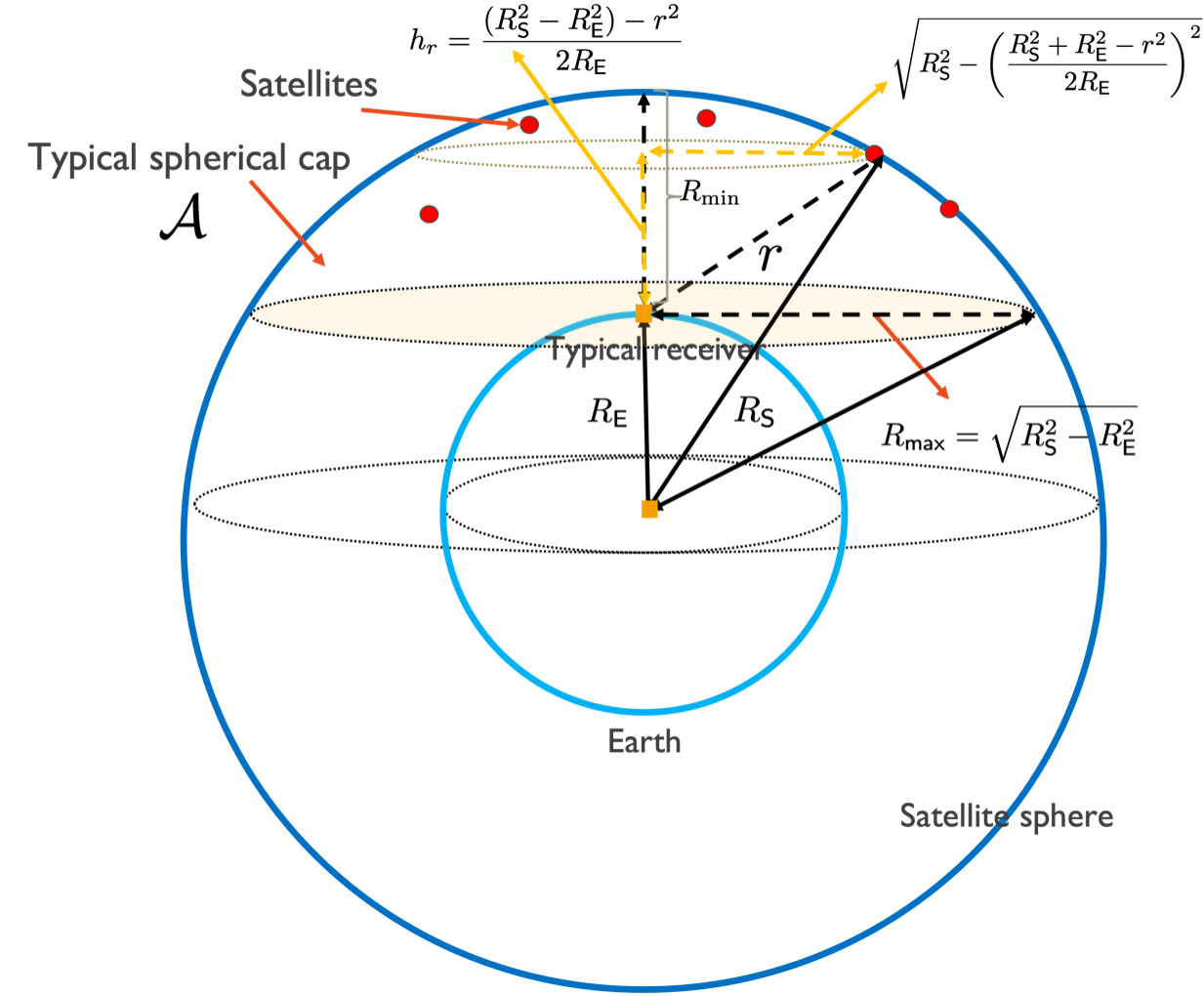
Satellite networks are promising to provide ubiquitous and high-capacity global wireless connectivity. Traditionally, satellite networks are modeled by placing satellites on a grid of multiple circular orbit geometries. Such a network model, however, requires intricate system-level simulations to evaluate coverage performance, and analytical understanding of the satellite network is limited. Continuing the success of stochastic geometry in a tractable analysis for terrestrial networks, in this paper, we develop novel models that are tractable for the coverage analysis of satellite networks using stochastic geometry. By modeling the locations of satellites and users using Poisson point processes on the surfaces of concentric spheres, we characterize analytical expressions for the coverage probability of a typical downlink user as a function of relevant parameters, including path-loss exponent, satellite height, density, and Nakagami fading parameter. Then, we also derive a tight lower bound of the coverage probability in tractable expression while keeping full generality. Leveraging the derived expression, we identify the optimal density of satellites in terms of the height and the path-loss exponent. Our key finding is that the optimal average number of satellites decreases logarithmically with the satellite height to maximize the coverage performance. Simulation results verify the exactness of the derived expressions.
翻译:传统上,卫星网络是通过将卫星置于多环轨道地理分布网格上的卫星模型建模而建模的。然而,这种网络模型需要复杂的系统级模拟来评价覆盖性,对卫星网络的分析理解有限。在对地面网络进行可移植的分析中,我们继续成功地进行随机几何测量,在本文件中,我们开发了新的模型,这些模型可用于利用随机几何测量法对卫星网络进行覆盖分析。通过将卫星和用户的位置建模,在同心层表面使用Poisson点进程进行模拟,我们将典型的下链接用户的覆盖概率作为相关参数的函数,包括路径损益度、卫星高度、密度和纳卡穆光伏参数。然后,我们还从可移动表达的覆盖概率中得出一个较窄的界限,同时保持完全的笼统性。我们利用衍生的表达法,从高度和路径损耗竭度的角度,确定卫星的最佳密度。我们的关键发现是,卫星最优平均性能率率将卫星的测算结果降低到精确性。