A differential approach to detecting projective equivalences and symmetries of rational 3D curves

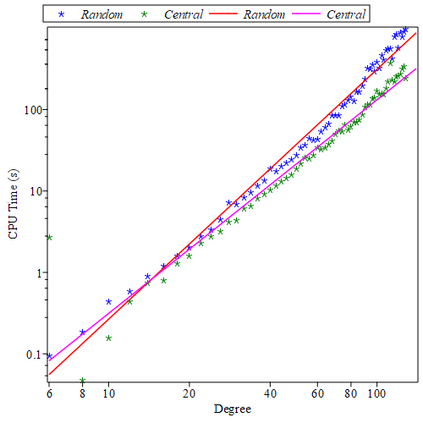
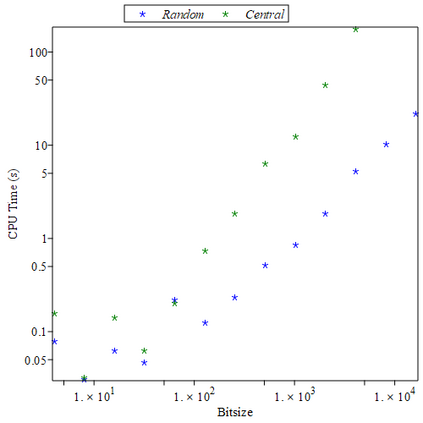
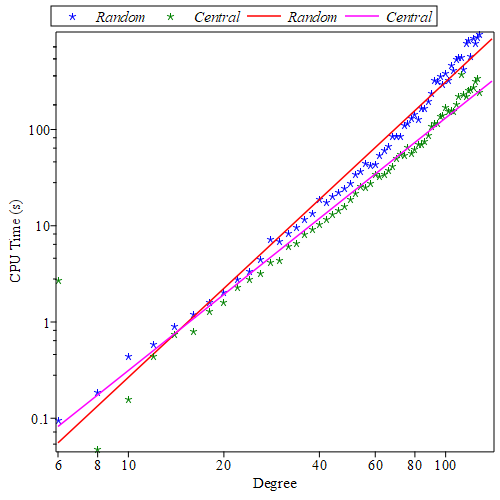
We present a new approach to detecting projective equivalences and symmetries of rational parametric 3D curves. To detect projective equivalences, we first derive two projective differential invariants that are also invariant with respect to the change of parameters called M\"obius transformations. Given two rational curves, we form a system consists of two homogeneous polynomials in four variables using the projective differential invariants. The solution of the system yields the M\"obius transformations, each of which corresponds to a projective equivalence. If the input curves are the same, then our method detects the projective symmetries of the input curve. Our method is substantially faster than methods addressing a similar problem and provides solutions even for the curves with degree up to 24 and coefficients up to 78 digits.