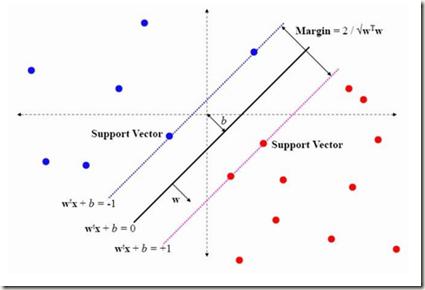
A "dark cloud" hangs over numerical optimization theory for decades, namely, whether an optimization algorithm $O(\log(n))$ iteration complexity exists. "Yes", this paper answers, with a new optimization algorithm and strict theory proof. It starts with box-constrained quadratic programming (Box-QP), and many practical optimization problems fall into Box-QP. General smooth quadratic programming (QP), nonsmooth Lasso, and support vector machine (or regression) can be reformulated as Box-QP via duality theory. It is the first time to present an $O(\log(n))$ iteration complexity QP algorithm, in particular, which behaves like a "direct" method: the required number of iterations is deterministic with exact value $\left\lceil\log\left(\frac{3.125n}{\epsilon}\right)/\log(1.5625)\right\rceil$. This significant breakthrough enables us to transition from the $O(\sqrt{n})$ to the $O(\log(n))$ optimization algorithm, whose amazing scalability is particularly relevant in today's era of big data and artificial intelligence.
翻译:暂无翻译