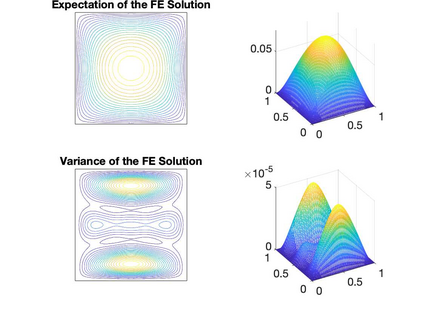
A general adaptive refinement strategy for solving linear elliptic partial differential equation with random data is proposed and analysed herein. The adaptive strategy extends the a posteriori error estimation framework introduced by Guignard and Nobile in 2018 (SIAM J. Numer. Anal., 56, 3121--3143) to cover problems with a nonaffine parametric coefficient dependence. A suboptimal, but nonetheless reliable and convenient implementation of the strategy involves approximation of the decoupled PDE problems with a common finite element approximation space. Computational results obtained using such a single-level strategy are presented in this paper (part I). Results obtained using a potentially more efficient multilevel approximation strategy, where meshes are individually tailored, will be discussed in part II of this work. The codes used to generate the numerical results are available online.
翻译:本文提出并分析了解决线性椭圆部分差分方程的一般性适应性完善战略,并随机提供数据。适应性战略扩展了Guignard和Nobile在2018年推出的事后误差估计框架(SIAM J.Numer.Anal., 56, 3121-3143),以涵盖非按成因参数系数依赖性的问题。战略执行不理想,但可靠和方便,包括将脱钩的PDE问题与共同的有限元素近似空间相近。本文件介绍了使用这种单一层次战略取得的计算结果(第一部分)。使用可能更加有效的多层次近似值战略取得的成果,其中的Memshes是单独定制的,将在这项工作的第二部分加以讨论。用于产生数字结果的代码可在网上查阅。