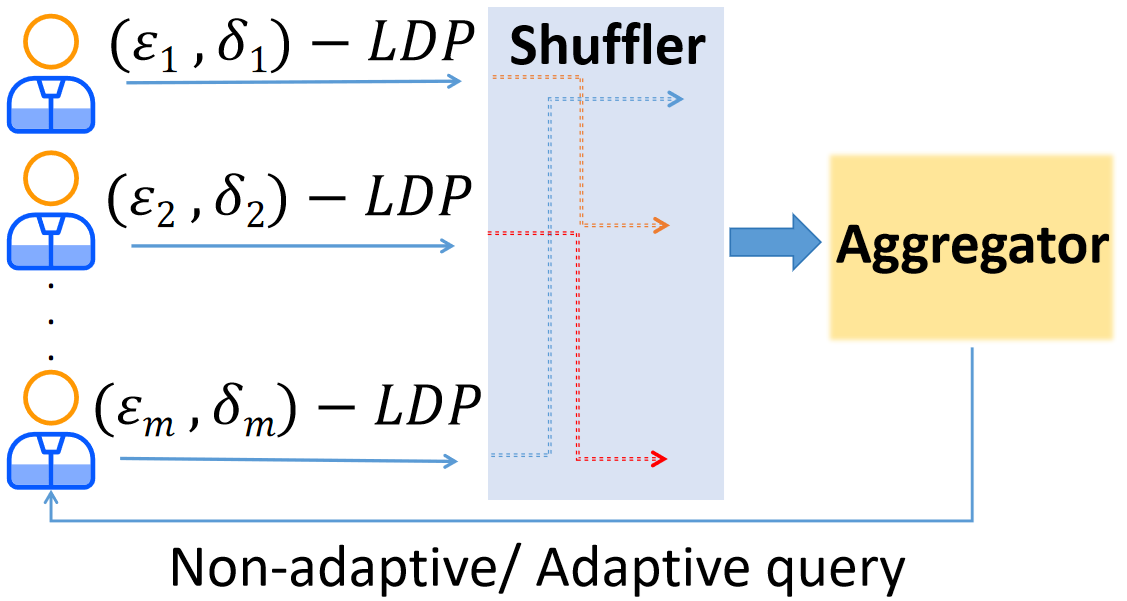
The shuffle model of local differential privacy is an advanced method of privacy amplification designed to enhance privacy protection with high utility. It achieves this by randomly shuffling sensitive data, making linking individual data points to specific individuals more challenging. However, most existing studies have focused on the shuffle model based on $(\epsilon_0,0)$-Locally Differentially Private (LDP) randomizers, with limited consideration for complex scenarios such as $(\epsilon_0,\delta_0)$-LDP or personalized LDP (PLDP). This hinders a comprehensive understanding of the shuffle model's potential and limits its application in various settings. To bridge this research gap, we propose a generalized shuffle framework that can be applied to any $(\epsilon_i,\delta_i)$-PLDP setting with personalized privacy parameters. This generalization allows for a broader exploration of the privacy-utility trade-off and facilitates the design of privacy-preserving analyses in diverse contexts. We prove that shuffled $(\epsilon_i,\delta_i)$-PLDP process approximately preserves $\mu$-Gaussian Differential Privacy with \mu = \sqrt{\frac{2}{\sum_{i=1}^{n} \frac{1-\delta_i}{1+e^{\epsilon_i}}-\max_{i}{\frac{1-\delta_{i}}{1+e^{\epsilon_{i}}}}}}. $ This approach allows us to avoid the limitations and potential inaccuracies associated with inequality estimations. To strengthen the privacy guarantee, we improve the lower bound by utilizing hypothesis testing} instead of relying on rough estimations like the Chernoff bound or Hoeffding's inequality. Furthermore, extensive comparative evaluations clearly show that our approach outperforms existing methods in achieving strong central privacy guarantees while preserving the utility of the global model. We have also carefully designed corresponding algorithms for average function, frequency estimation, and stochastic gradient descent.
翻译:暂无翻译