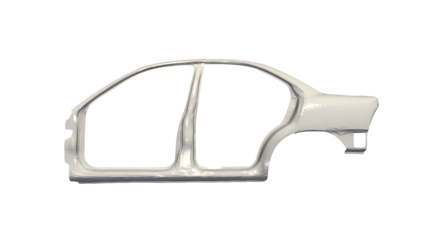
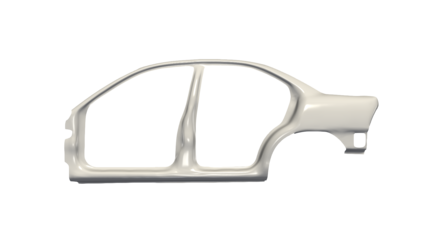
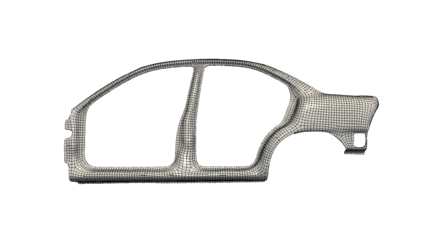
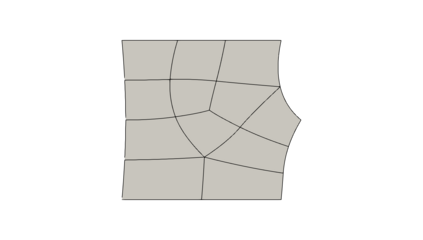
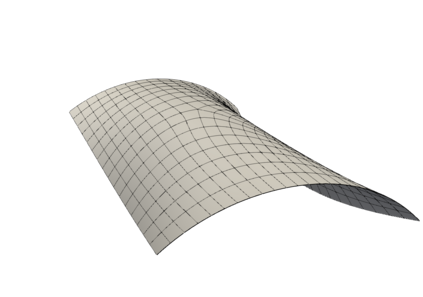
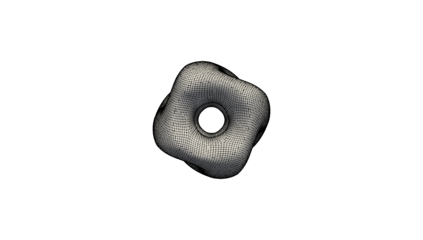
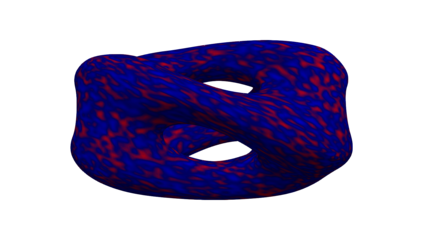
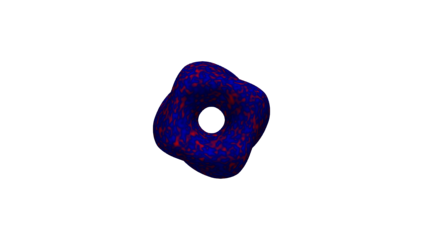
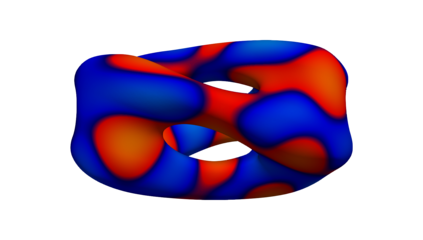
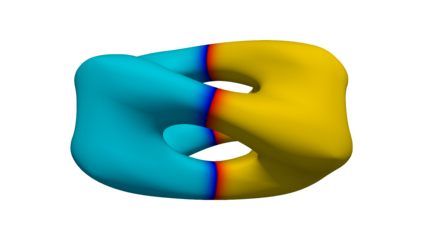
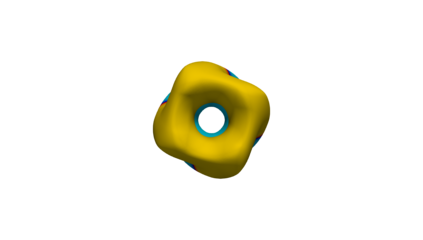
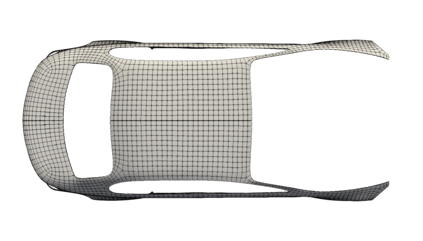
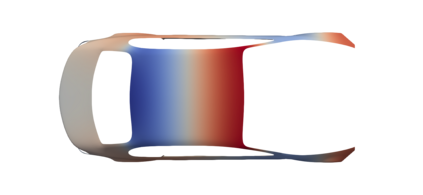
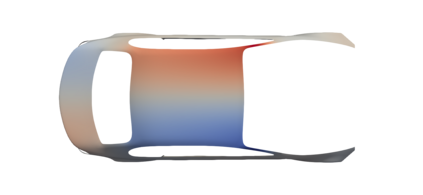
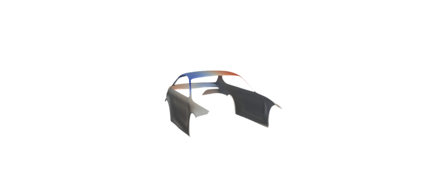
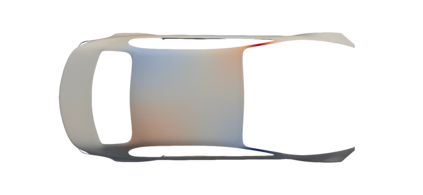
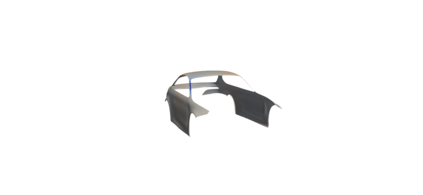
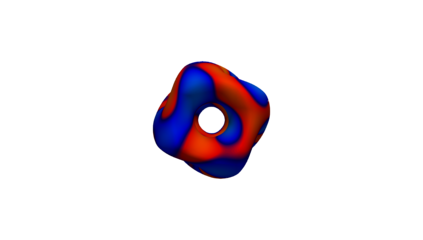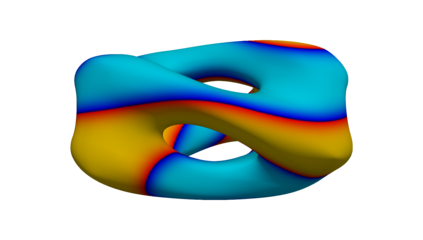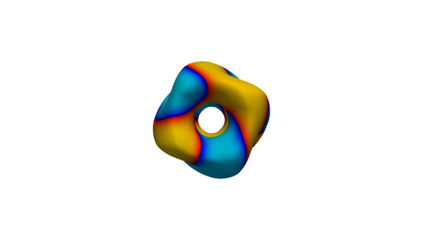Isogeometric Analysis generalizes classical finite element analysis and intends to integrate it with the field of Computer-Aided Design. A central problem in achieving this objective is the reconstruction of analysis-suitable models from Computer-Aided Design models, which is in general a non-trivial and time-consuming task. In this article, we present a novel spline construction, that enables model reconstruction as well as simulation of high-order PDEs on the reconstructed models. The proposed almost-$C^1$ are biquadratic splines on fully unstructured quadrilateral meshes (without restrictions on placements or number of extraordinary vertices). They are $C^1$ smooth almost everywhere, that is, at all vertices and across most edges, and in addition almost (i.e. approximately) $C^1$ smooth across all other edges. Thus, the splines form $H^2$-nonconforming analysis-suitable discretization spaces. This is the lowest-degree unstructured spline construction that can be used to solve fourth-order problems. The associated spline basis is non-singular and has several B-spline-like properties (e.g., partition of unity, non-negativity, local support), the almost-$C^1$ splines are described in an explicit B\'ezier-extraction-based framework that can be easily implemented. Numerical tests suggest that the basis is well-conditioned and exhibits optimal approximation behavior.
翻译:直观分析 直观分析 直观的经典定点元素分析, 并打算将其整合到计算机辅助设计领域 。 实现这一目标的一个中心问题是重塑计算机辅助设计模型的分析适用模型, 一般来说, 它是一个非三角和耗时的任务 。 在本篇文章中, 我们展示了一个新颖的样条结构, 使得模型重建以及模拟重塑模型中的高阶 PDE 。 拟议的几乎- $C $ 1 是在完全不结构的四边网( 不受放置限制或超常的顶脊数限制) 。 实现此目标的一个中心问题是, 从计算机辅助设计模型中重建分析适合的模型。 这些模型几乎在各地都是平坦的, 也就是在大多数边缘上, 平坦的、 平坦直径框架, 最平坦的、 平坦直径的平面、 直径平面框架 、 直径直的测试基础、 直平面的、 直径直线、 直的底基、 直径基、 直径基、 直径基、 直基、 直径直的、 直基、 直线、 直基、 直径基、 直基、 直基、 直基、 直基、 直的、 直基、 直基、 直是非基、 直基、 直基、 直、 直、 直、 直、 直基、 底、 直、 直、 底、 底、 底、 直基、 底、 直系、 底、 底、 底、 底、 底、 基、 直基、 底、 基、 底、 底、 直基、 非基、 非基、 、 、 非基、 底、 基、 、 、 、 基、 基、 基、 基、 、 基、 基、 非基、 底、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 非基、 底、 非基、 底、 非基、 底、 底、 基、 底、 底