Linear Delta Arrays for Dexterous Distributed Manipulation

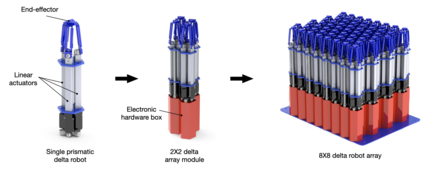
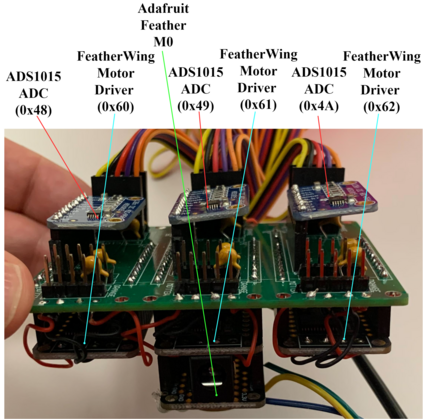
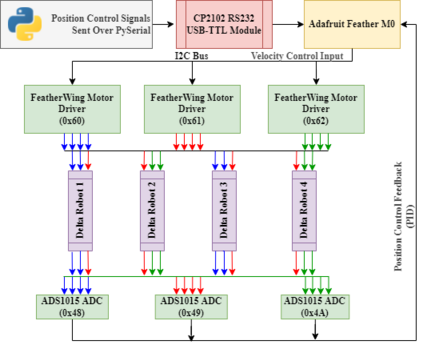
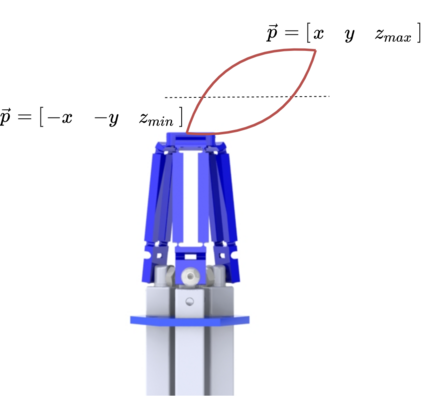
This paper presents a new type of distributed dexterous manipulators: delta arrays. Each delta array consists of a grid of linearly-actuated delta robots with compliant 3D-printed parallelogram links. These arrays can be used to perform planar transportation tasks, similar to smart conveyors. However, the deltas' additional degrees of freedom also afford a wide range of out-of-plane manipulations, as well as prehensile manipulations between sets of deltas. A delta array thus affords a wide range of distributed manipulation strategies. In this paper, we present the design of the delta arrays, including the individual deltas, a modular array structure, and distributed communication and control. We also construct and evaluate an 8x8 array using the proposed design. Our evaluations show that the resulting 192 DoF robot is capable of performing various coordinated distributed manipulations of a variety of objects, including translation, alignment, and prehensile squeezing.