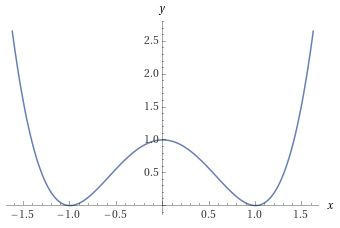
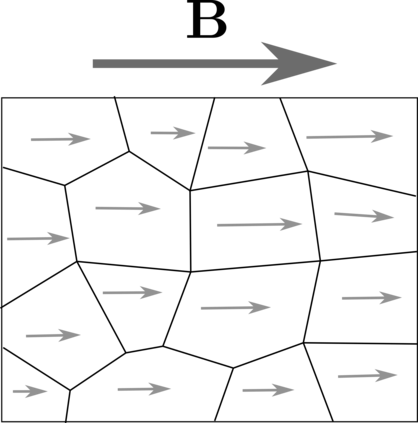
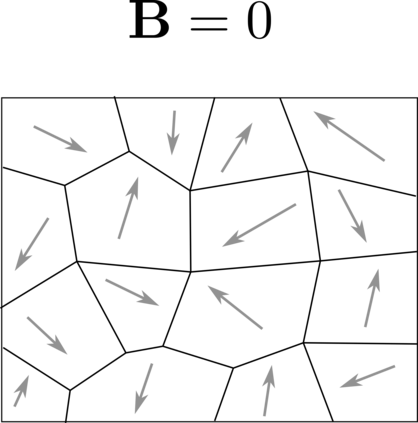
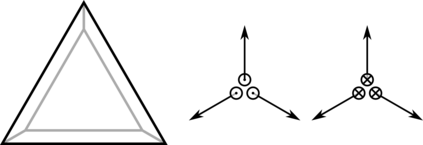
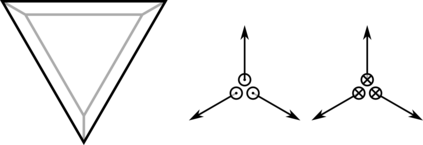
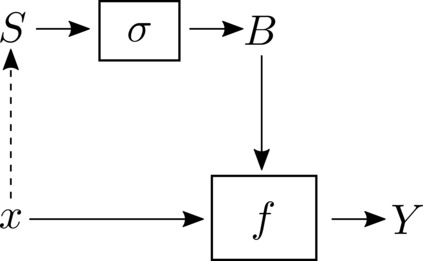
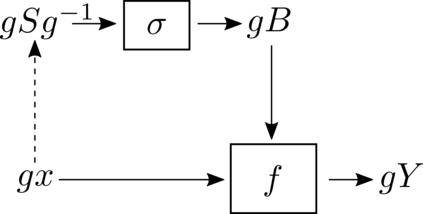
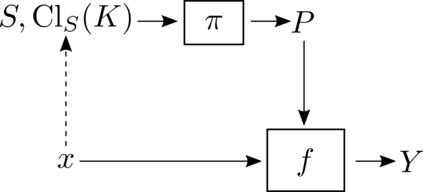
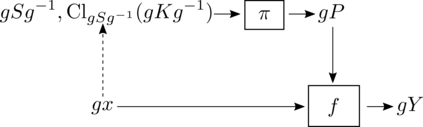
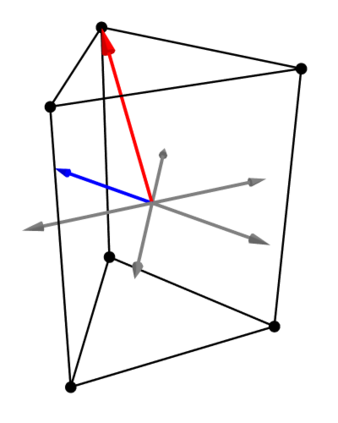
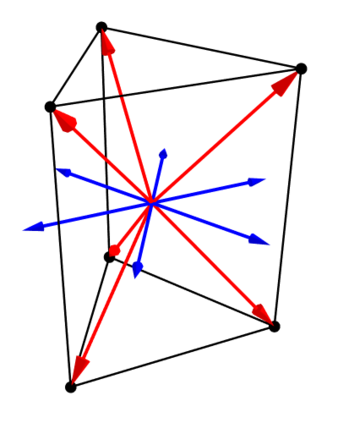
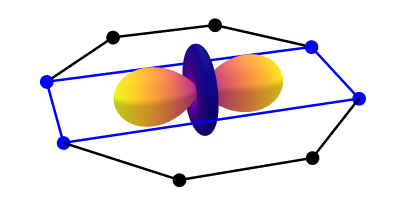
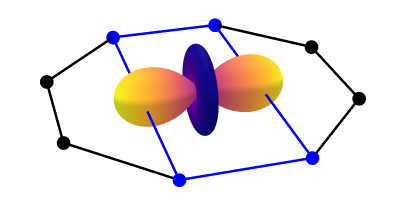
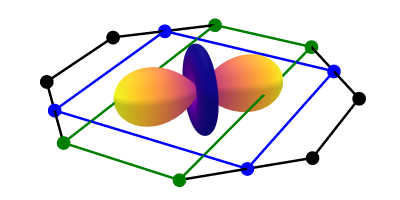
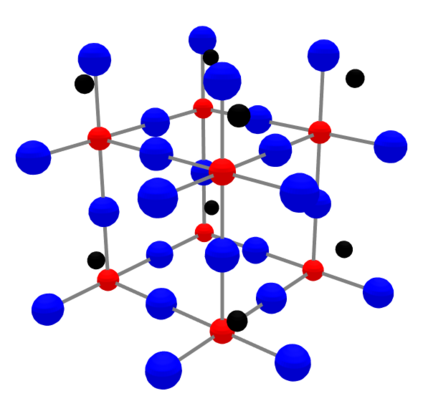
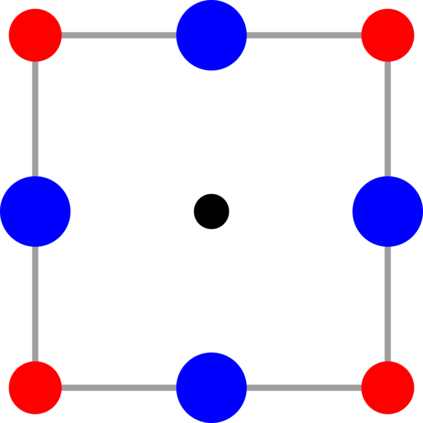
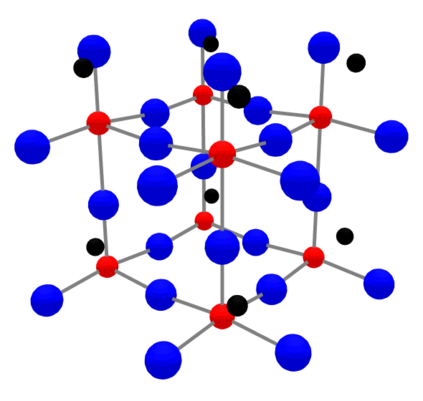
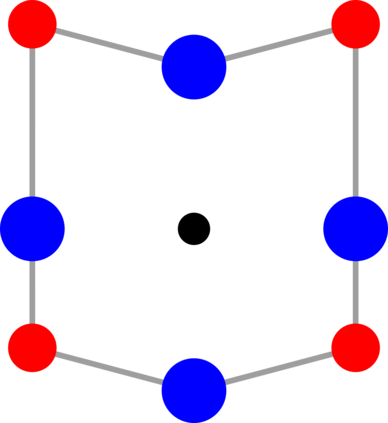
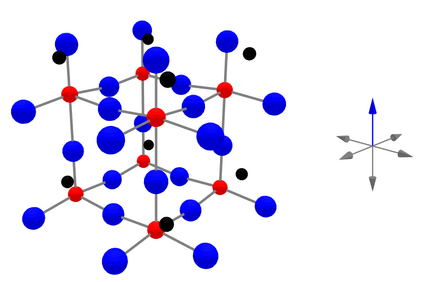
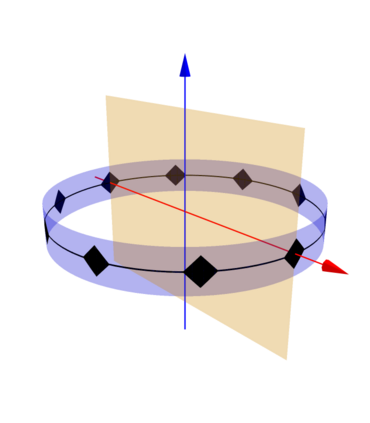
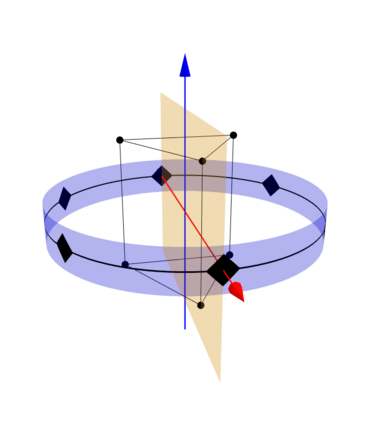
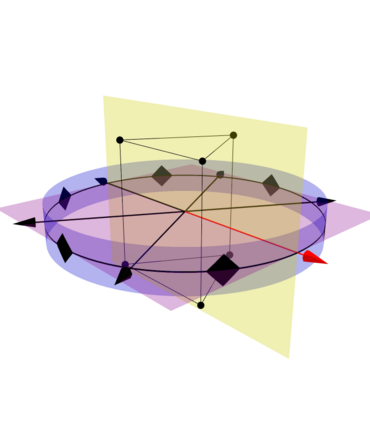
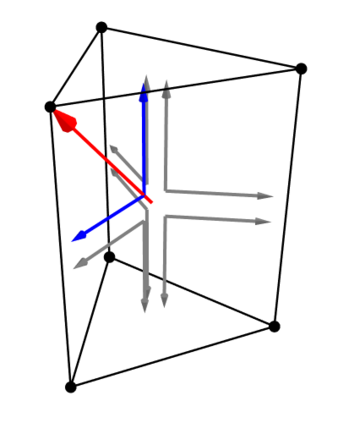
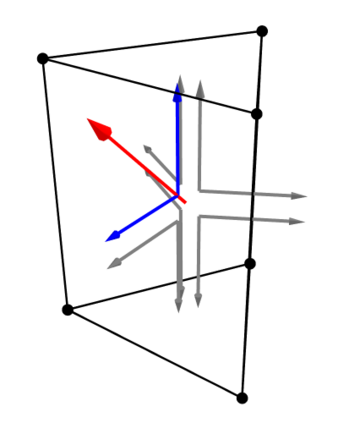
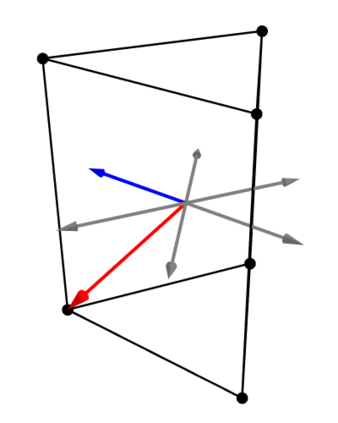
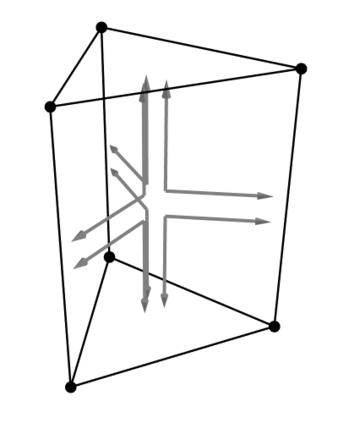
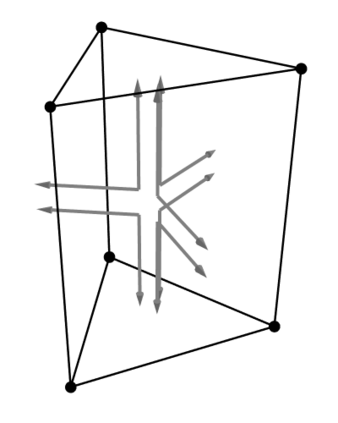
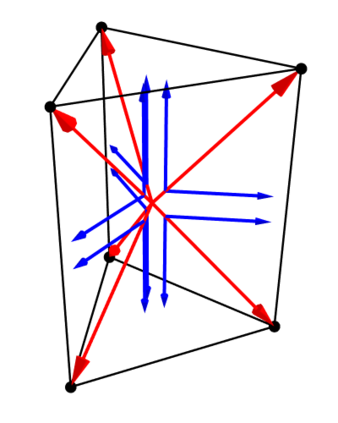
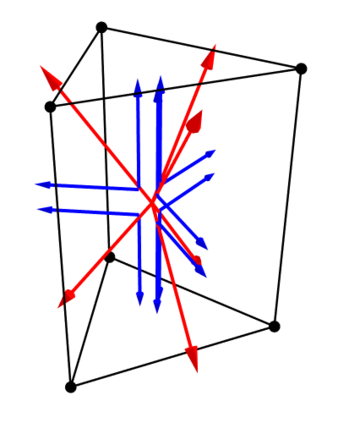
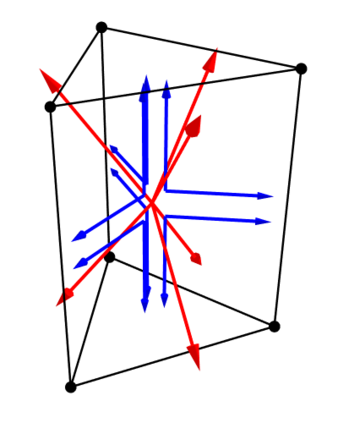
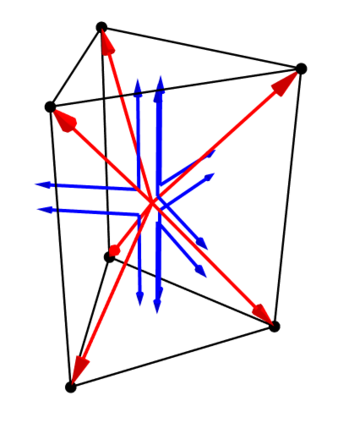
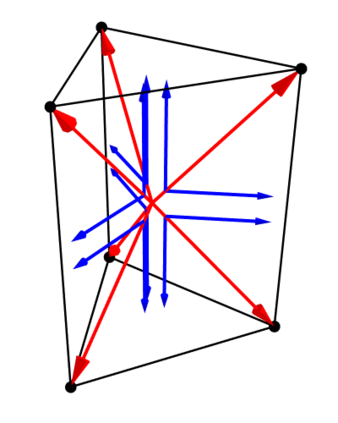
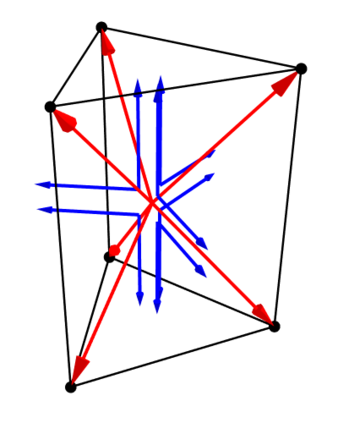
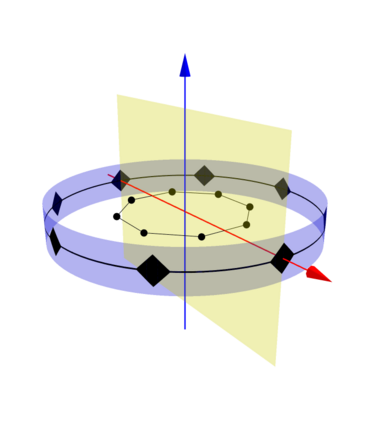
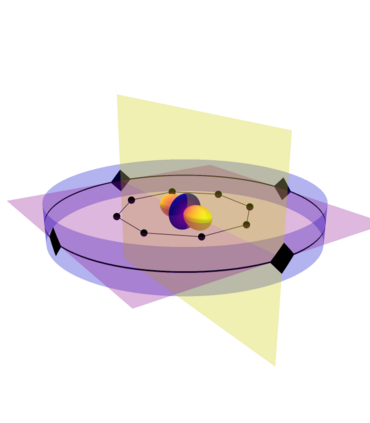
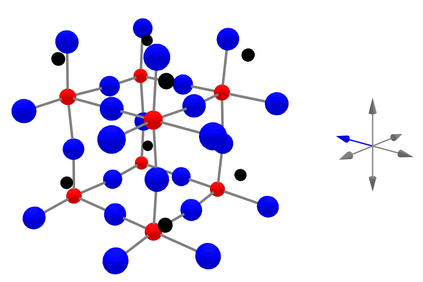
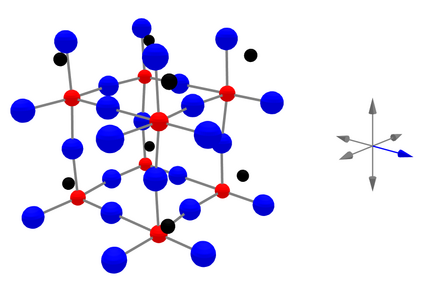
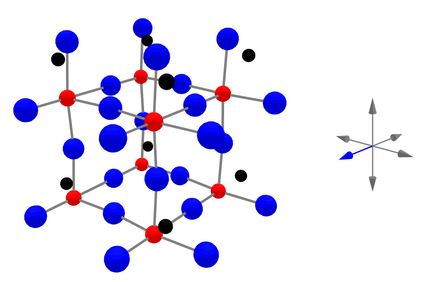
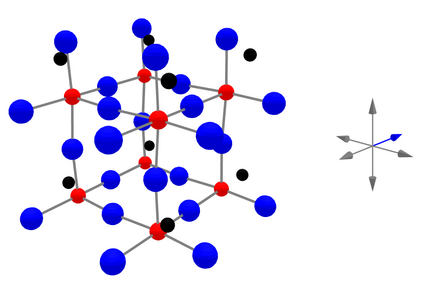
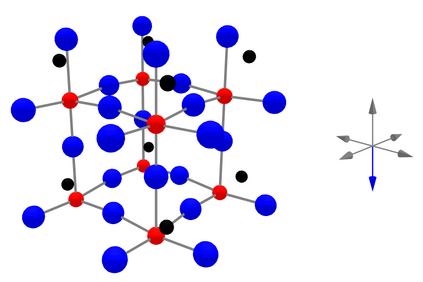
Equivariant neural networks (ENNs) have been shown to be extremely effective in applications involving underlying symmetries. By construction ENNs cannot produce lower symmetry outputs given a higher symmetry input. However, symmetry breaking occurs in many physical systems and we may obtain a less symmetric stable state from an initial highly symmetric one. Hence, it is imperative that we understand how to systematically break symmetry in ENNs. In this work, we propose a novel symmetry breaking framework that is fully equivariant and is the first which fully addresses spontaneous symmetry breaking. We emphasize that our approach is general and applicable to equivariance under any group. To achieve this, we introduce the idea of symmetry breaking sets (SBS). Rather than redesign existing networks, we design sets of symmetry breaking objects which we feed into our network based on the symmetry of our inputs and outputs. We show there is a natural way to define equivariance on these sets, which gives an additional constraint. Minimizing the size of these sets equates to data efficiency. We prove that minimizing these sets translates to a well studied group theory problem, and tabulate solutions to this problem for the point groups. Finally, we provide some examples of symmetry breaking to demonstrate how our approach works in practice. The code for these examples is available at \url{https://github.com/atomicarchitects/equivariant-SBS}.
翻译:暂无翻译