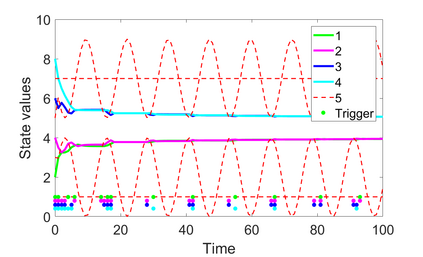
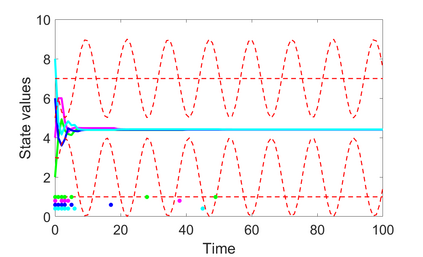
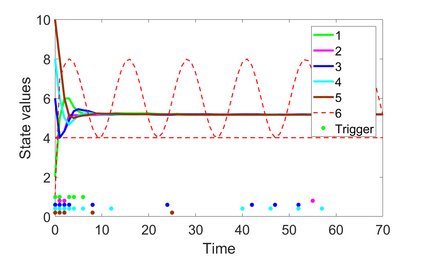
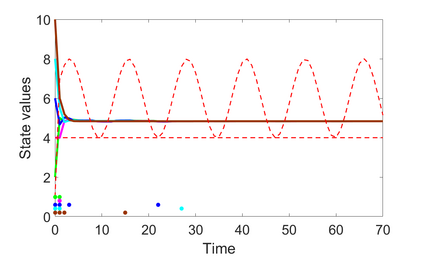
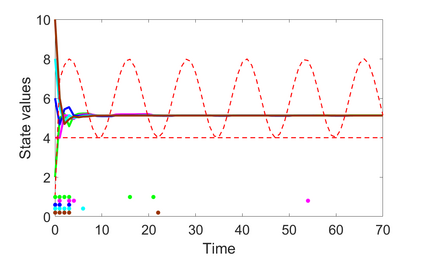
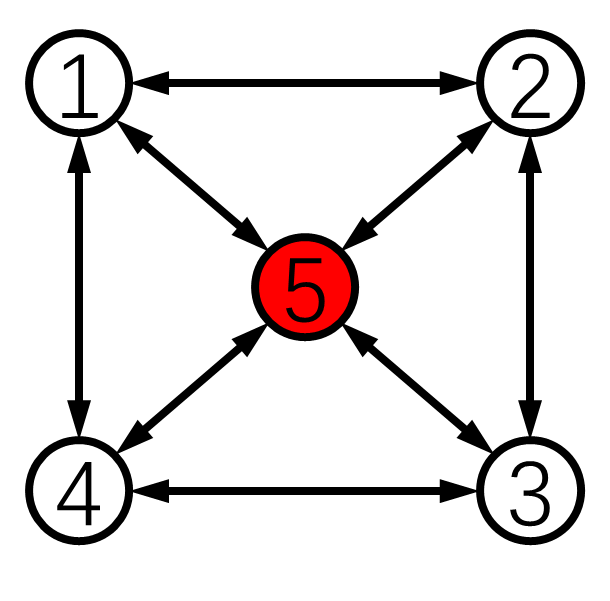
In this paper, we consider a resilient consensus problem for the multi-agent network where some of the agents are subject to Byzantine attacks and may transmit erroneous state values to their neighbors. In particular, we develop an event-triggered update rule to tackle this problem as well as reduce the communication for each agent. Our approach is based on the mean subsequence reduced (MSR) algorithm with agents being capable to communicate with multi-hop neighbors. Since delays are critical in such an environment, we provide necessary graph conditions for the proposed algorithm to perform well with delays in the communication. We highlight that through multi-hop communication, the network connectivity can be reduced especially in comparison with the common onehop communication case. Lastly, we show the effectiveness of the proposed algorithm by a numerical example.
翻译:在本文中,我们考虑了多试剂网络的弹性共识问题,在多试剂网络中,一些代理商受到拜占庭袭击,可能会将错误的国家价值传递给邻居。特别是,我们制定了一个事件触发更新规则,以解决这一问题并减少每个代理商的通信量。我们的方法是基于平均后继序列减少(MSR)算法,代理商能够与多霍特邻居进行通信。由于在这种环境中延误至关重要,我们为拟议的算法提供了必要的图表条件,以便随着通信的延误而运行。我们强调,通过多霍通信,网络连接可以减少,特别是与普通的单霍普通信案例相比。最后,我们用数字示例展示了提议的算法的有效性。