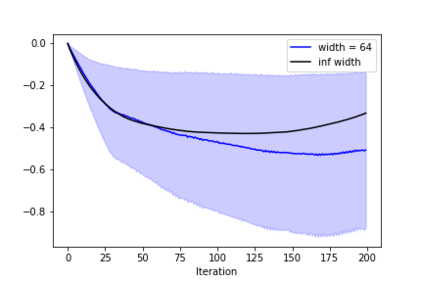
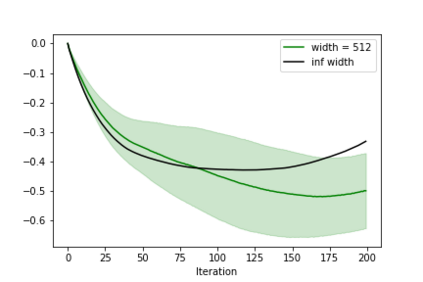
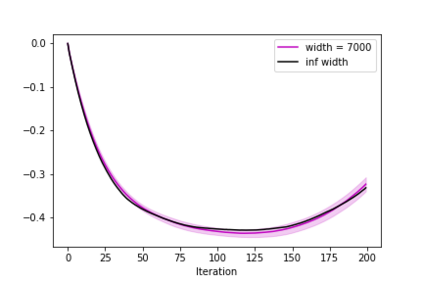
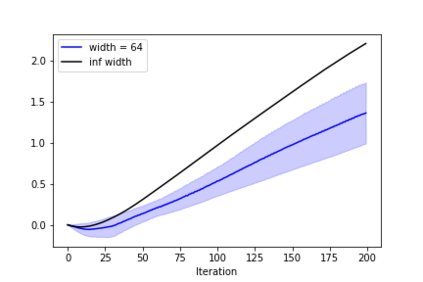
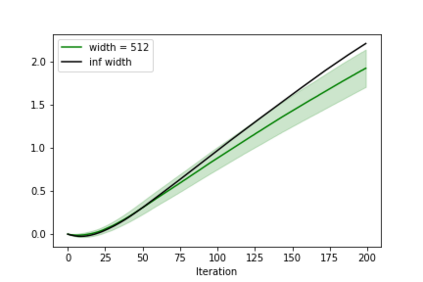
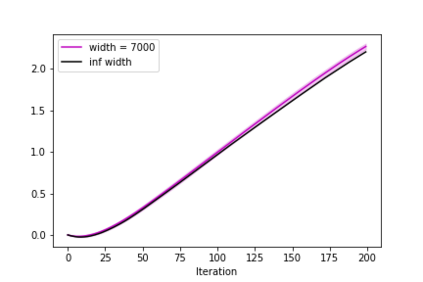
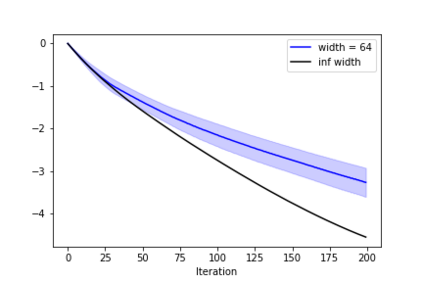
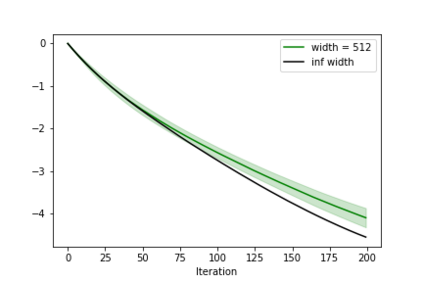
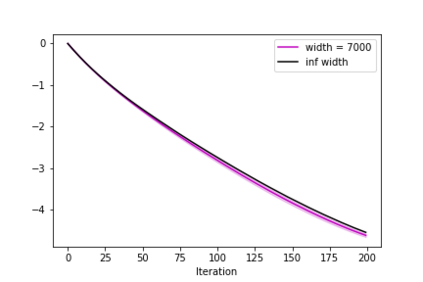
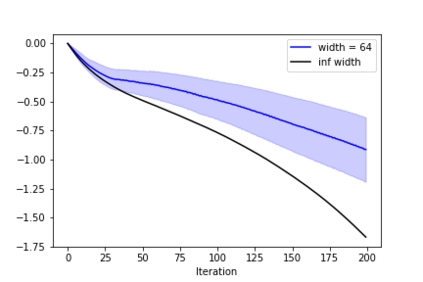
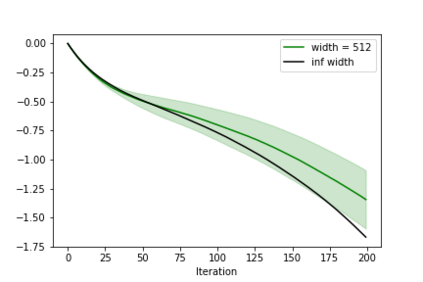
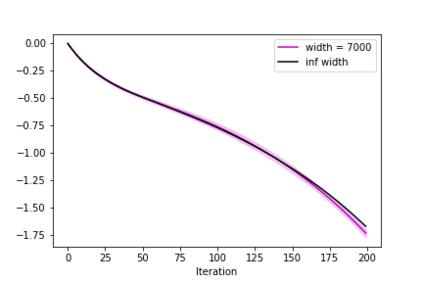
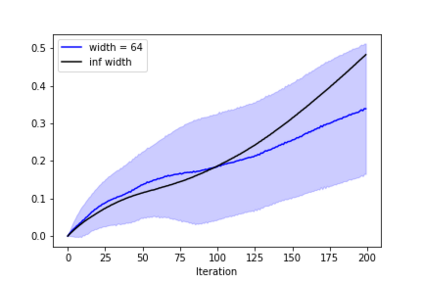
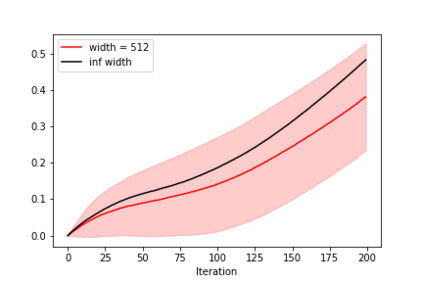
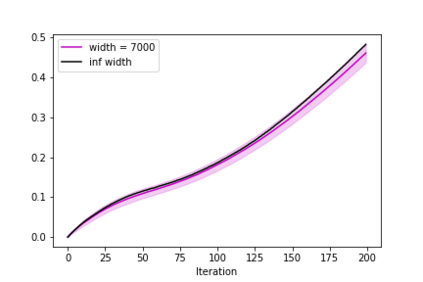
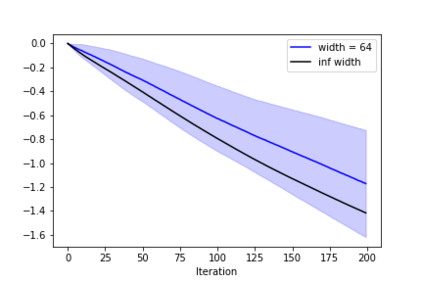
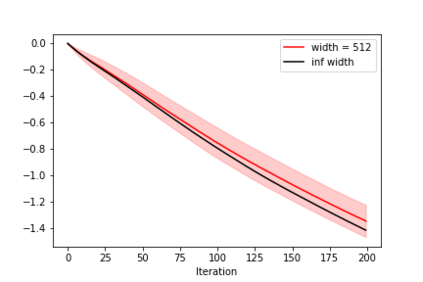
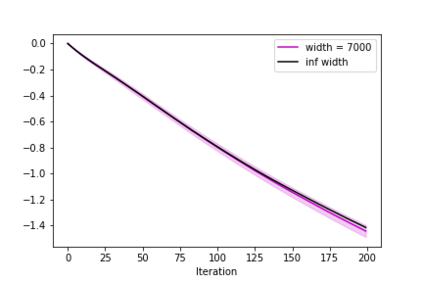
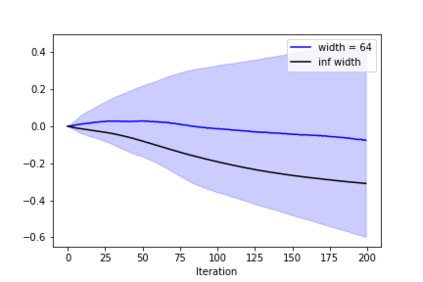
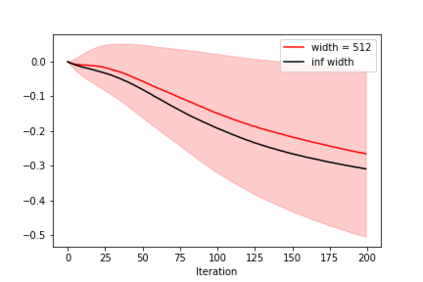
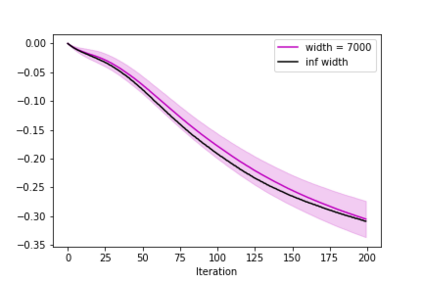
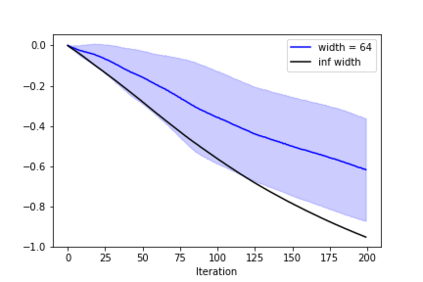
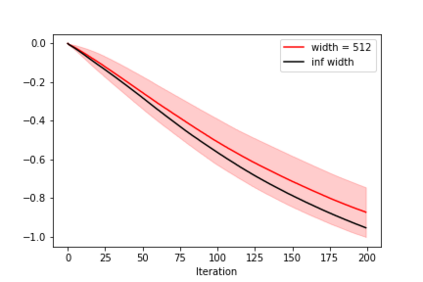
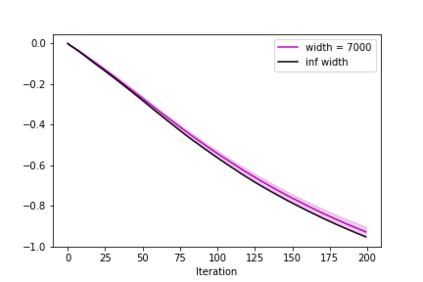
Going beyond stochastic gradient descent (SGD), what new phenomena emerge in wide neural networks trained by adaptive optimizers like Adam? Here we show: The same dichotomy between feature learning and kernel behaviors (as in SGD) holds for general optimizers as well, including Adam -- albeit with a nonlinear notion of "kernel." We derive the corresponding "neural tangent" and "maximal update" limits for any architecture. Two foundational advances underlie the above results: 1) A new Tensor Program language, NEXORT, that can express how adaptive optimizers process gradients into updates. 2) The introduction of bra-ket notation to drastically simplify expressions and calculations in Tensor Programs. This work summarizes and generalizes all previous results in the Tensor Programs series of papers.
翻译:暂无翻译