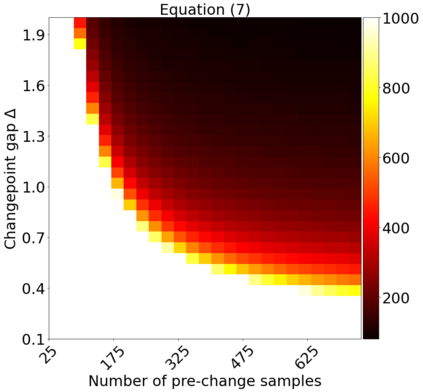
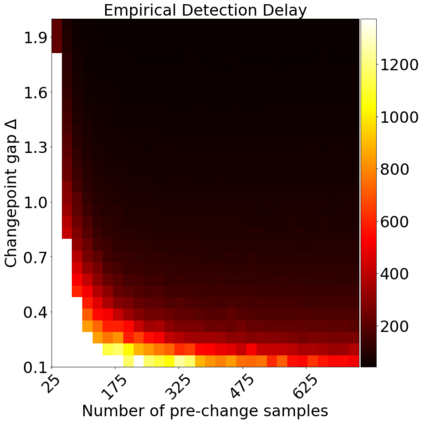
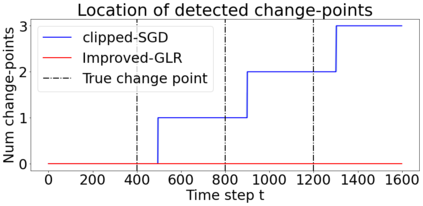
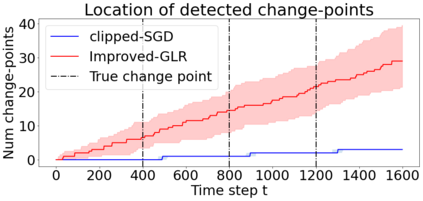
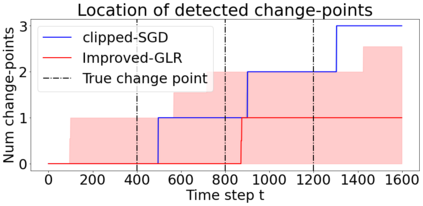
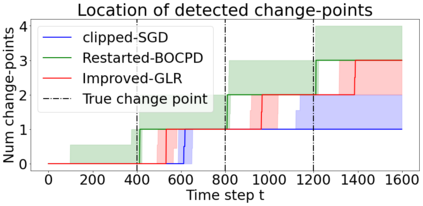
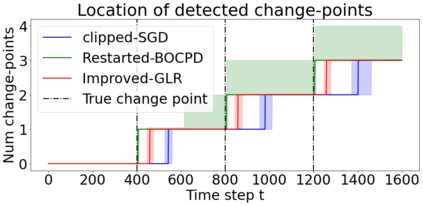
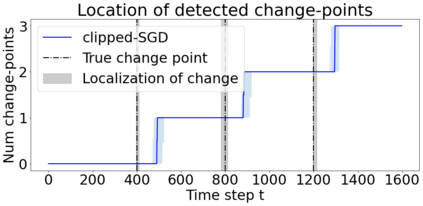
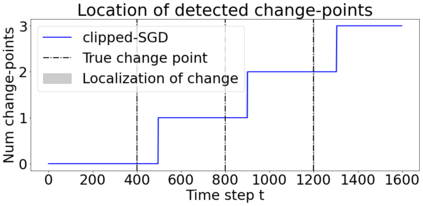
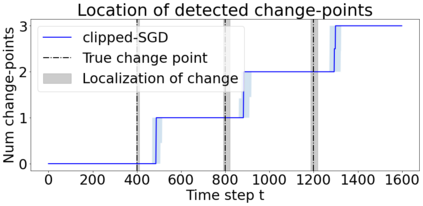
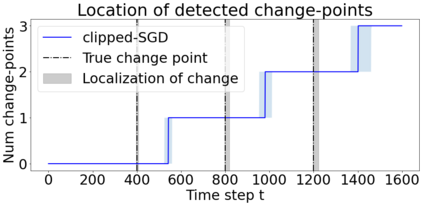
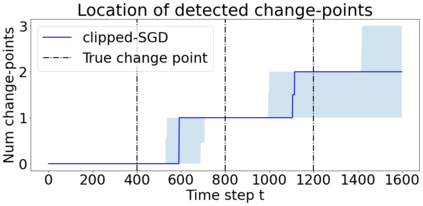
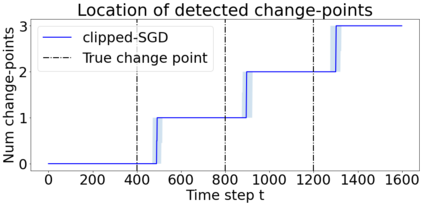
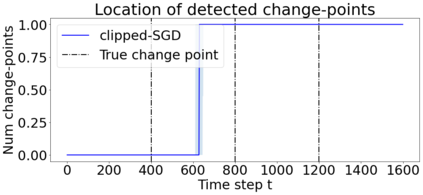
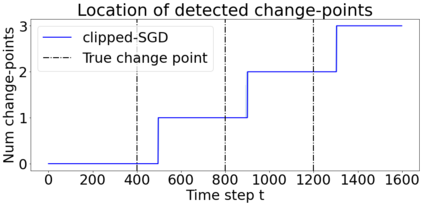
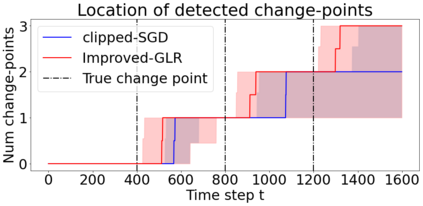
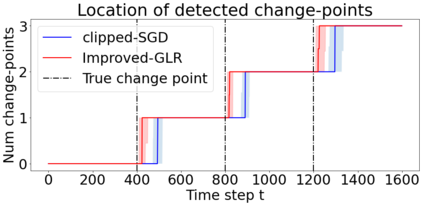
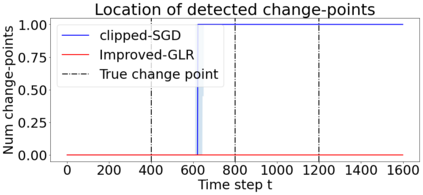
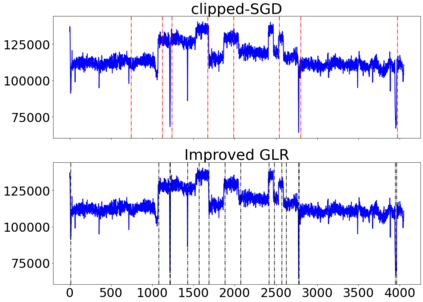
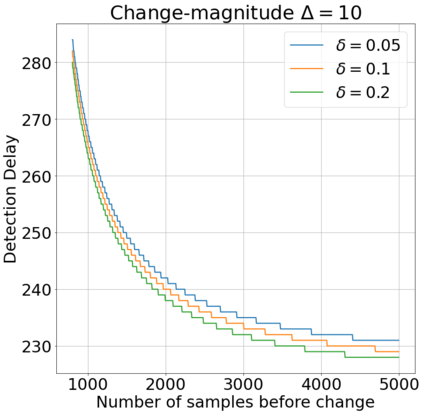
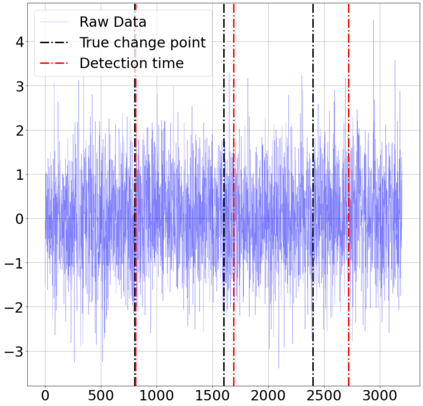
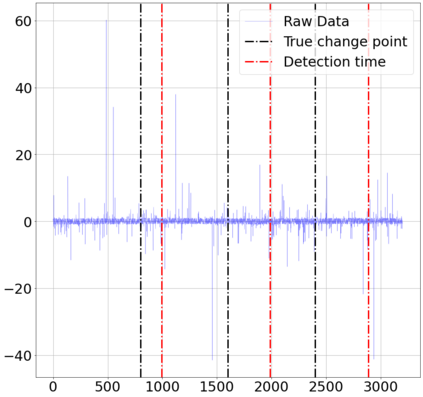
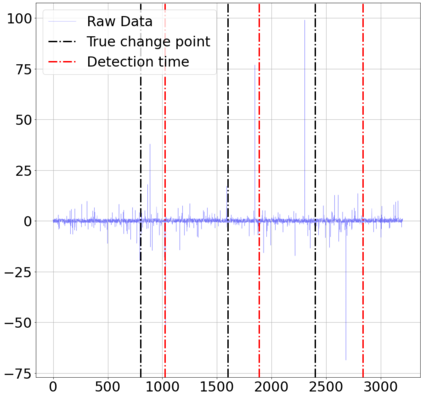
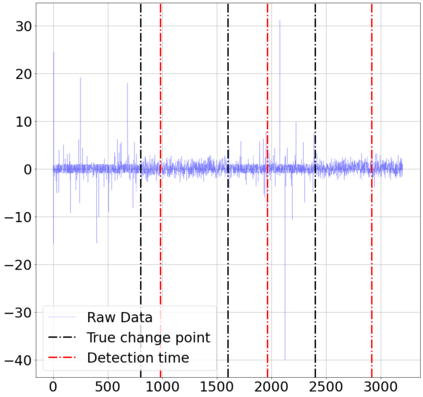
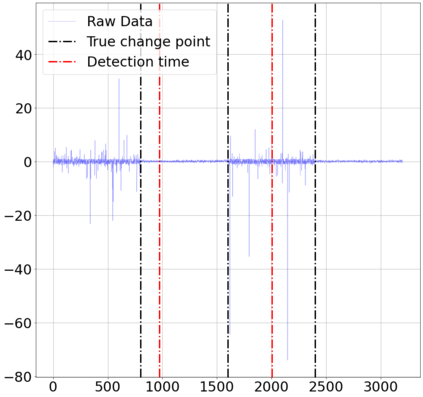
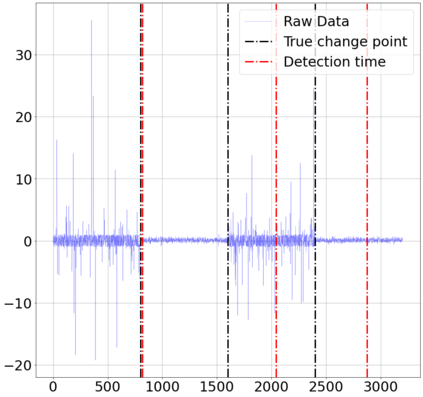
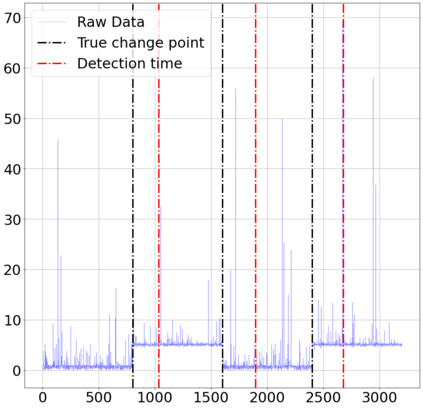
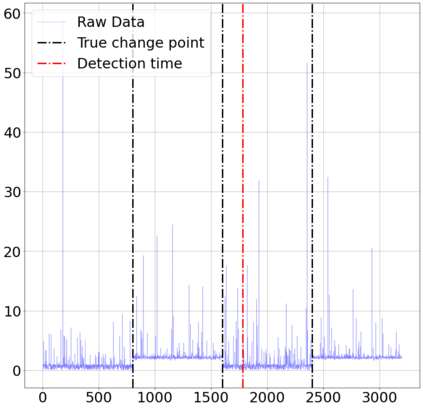
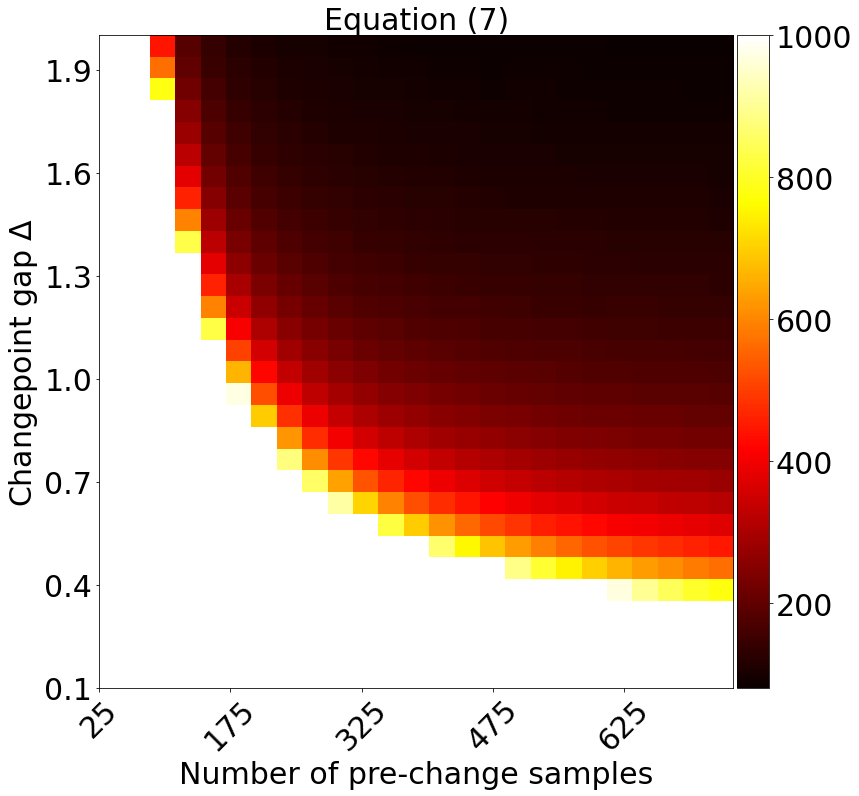
We study algorithms for online change-point detection (OCPD), where samples that are potentially heavy-tailed, are presented one at a time and a change in the underlying mean must be detected as early as possible. We present an algorithm based on clipped Stochastic Gradient Descent (SGD), that works even if we only assume that the second moment of the data generating process is bounded. We derive guarantees on worst-case, finite-sample false-positive rate (FPR) over the family of all distributions with bounded second moment. Thus, our method is the first OCPD algorithm that guarantees finite-sample FPR, even if the data is high dimensional and the underlying distributions are heavy-tailed. The technical contribution of our paper is to show that clipped-SGD can estimate the mean of a random vector and simultaneously provide confidence bounds at all confidence values. We combine this robust estimate with a union bound argument and construct a sequential change-point algorithm with finite-sample FPR guarantees. We show empirically that our algorithm works well in a variety of situations, whether the underlying data are heavy-tailed, light-tailed, high dimensional or discrete. No other algorithm achieves bounded FPR theoretically or empirically, over all settings we study simultaneously.
翻译:暂无翻译