A Structure-preserving, Operator Splitting Scheme for Reaction-Diffusion Equations Involving the Law of Mass Action

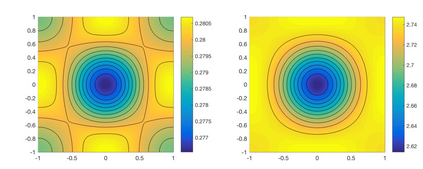
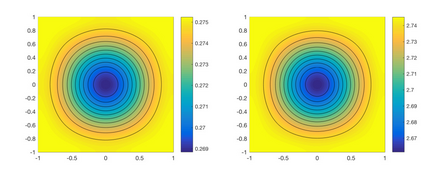
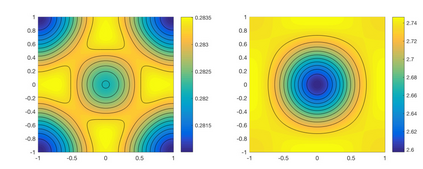
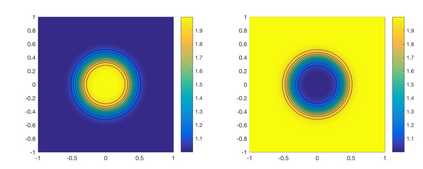
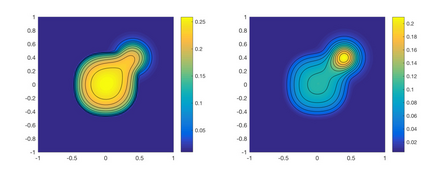
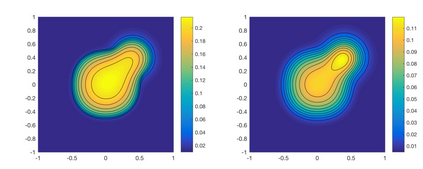
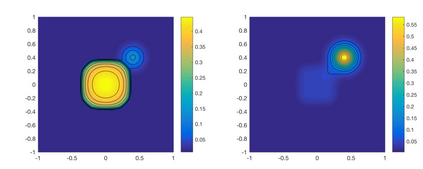
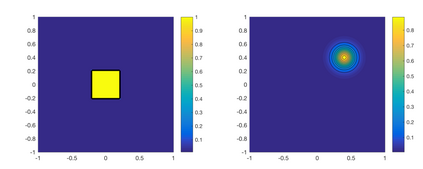
In this paper, we propose and analyze a positivity-preserving, energy stable numerical scheme for certain type reaction-diffusion systems involving the Law of Mass Action with the detailed balance condition. The numerical scheme is constructed based on a recently developed energetic variational formulation, in which the reaction part is reformulated in terms of reaction trajectories, and both the reaction and the diffusion parts dissipate the same free energy. This subtle fact opens a path of an operator splitting scheme for these systems. At the reaction stage, we solve equations of reaction trajectories by treating all the logarithmic terms in the reformulated form implicitly due to their convex nature. The positivity-preserving property and unique solvability can be theoretically proved, based on the singular behavior of the logarithmic function around the limiting value. Moreover, the energy stability of this scheme at the reaction stage can be proved by a careful convexity analysis. Similar techniques are used to establish the positivity-preserving property and energy stability for the standard semi-implicit solver at the diffusion stage. As a result, a combination of these two stages leads to a positivity-preserving and energy stable numerical scheme for the original reaction-diffusion system. To our best knowledge, it is the first time to report an energy-dissipation-law-based operator splitting scheme to a nonlinear PDE with variational structures. Several numerical examples are presented to demonstrate the robustness of the proposed operator splitting scheme.