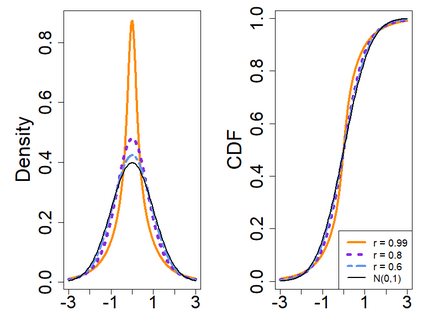
We present a general methodology to construct triplewise independent sequences of random variables having a common but arbitrary marginal distribution $F$ (satisfying very mild conditions). For two specific sequences, we obtain in closed form the asymptotic distribution of the sample mean. It is non-Gaussian (and depends on the specific choice of $F$). This allows us to illustrate the extent of the 'failure' of the classical central limit theorem (CLT) under triplewise independence. Our methodology is simple and can also be used to create, for any integer $K$, new $K$-tuplewise independent sequences that are not mutually independent. For $K \geq 4$, it appears that the sequences created using our methodology do verify a CLT, and we explain heuristically why this is the case.
翻译:我们提出了一个总体方法,用以构建三维独立的随机变量序列,这些变量具有共同但任意的边际分配费$(满足非常温和的条件),对于两个具体序列,我们以封闭的形式获得了样本平均值的无光度分布。它不是Gausian(并且取决于具体选择的$F美元)。这使我们能够说明在三维独立的情况下经典中央界限理论(CLT)的“失败”程度。我们的方法很简单,也可以用来为任何整数的$K美元创建非相互独立的新的基元-双基元独立序列。对于$K\geq 4美元,我们用我们的方法设定的序列似乎确实验证了CLT,我们从理论上解释为什么情况如此。