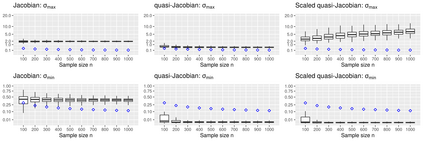
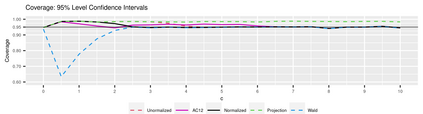
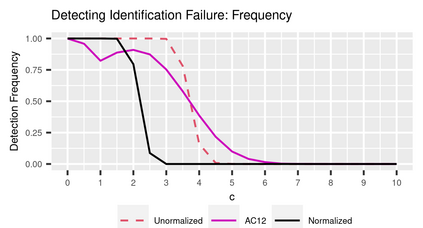
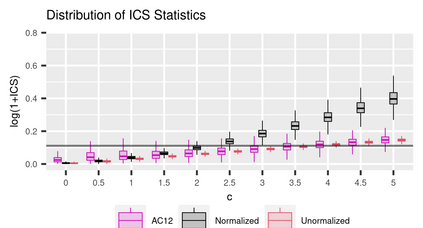
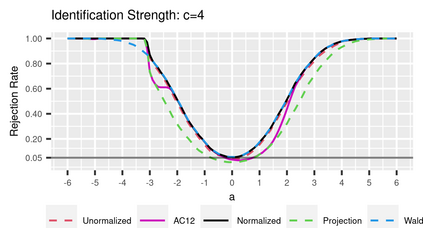
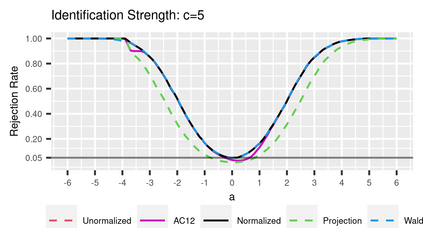
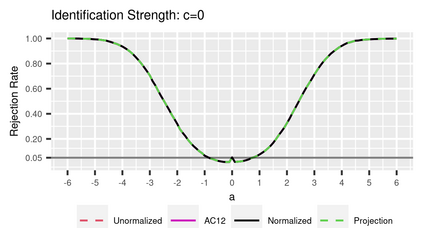
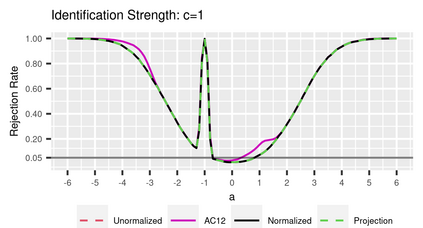
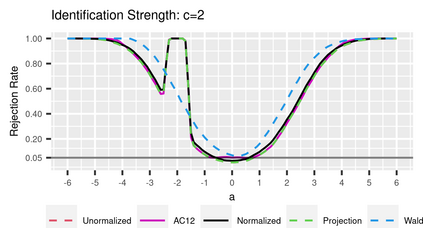
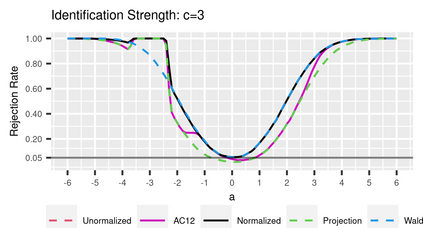
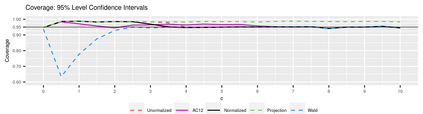
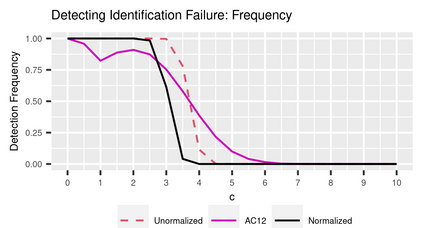
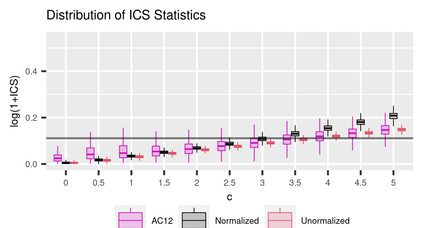
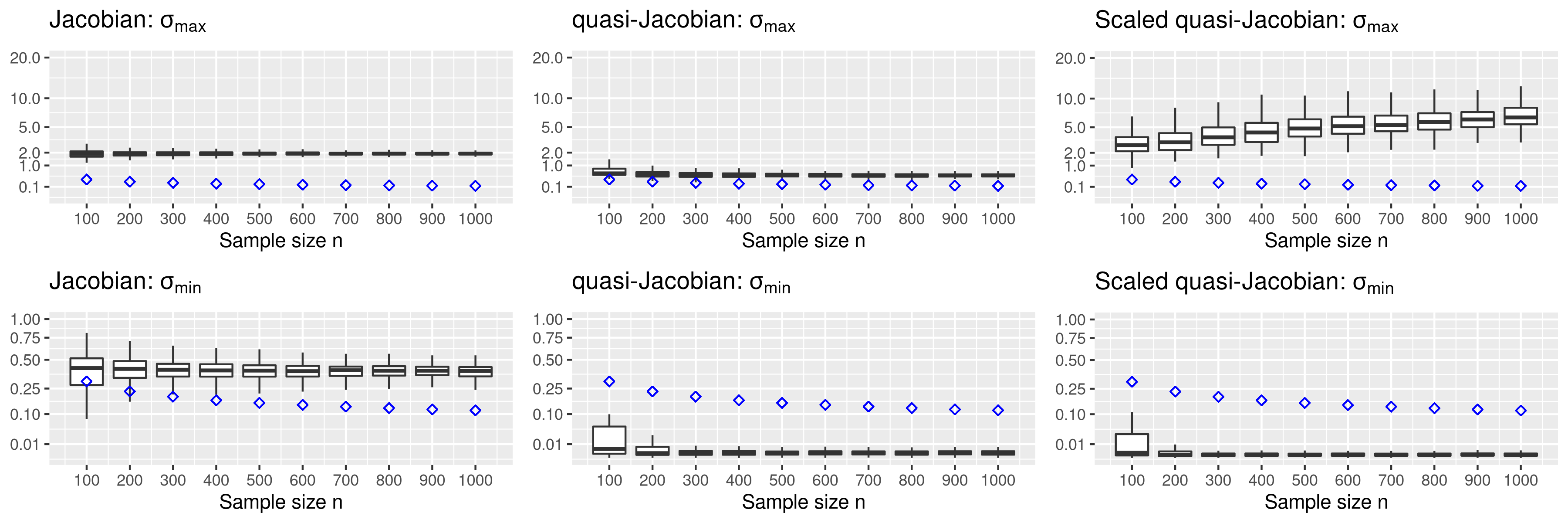
This paper develops an approach to detect identification failure in moment condition models. This is achieved by introducing a quasi-Jacobian matrix computed as the slope of a linear approximation of the moments on an estimate of the identified set. It is asymptotically singular when local and/or global identification fails, and equivalent to the usual Jacobian matrix which has full rank when the model is point and locally identified. Building on this property, a simple test with chi-squared critical values is introduced to conduct subvector inferences allowing for strong, semi-strong, and weak identification without \textit{a priori} knowledge about the underlying identification structure. Monte-Carlo simulations and an empirical application to the Long-Run Risks model illustrate the results.
翻译:本文开发了一种在瞬间状态模型中检测识别失败的方法。 这是通过引入一个准Jacobian矩阵来实现的。 该矩阵是作为所确定数据集估计时间的线性近似值的斜坡计算的。 当本地和(或)全球识别失败时,该矩阵与通常的Jacobian矩阵相当,当模型是点和本地识别时,该矩阵具有完全的等级。 以这一属性为基础,引入了一种带有基方临界值的简单测试,以进行子变量推论,允许进行强力、半坚固和薄弱的识别,而无需对基本识别结构的了解。 Monte-Carlo模拟和长程风险模型的经验应用都说明了结果。