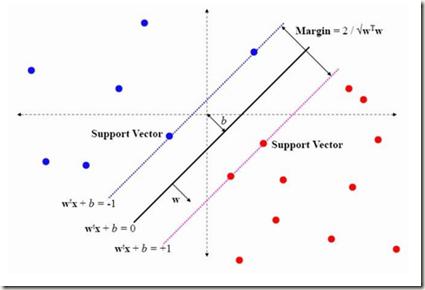
Quadratic programming is a fundamental problem in the field of convex optimization. Many practical tasks can be formulated as quadratic programming, for example, the support vector machine (SVM). Linear SVM is one of the most popular tools over the last three decades in machine learning before deep learning method dominating. In general, a quadratic program has input size $\Theta(n^2)$ (where $n$ is the number of variables), thus takes $\Omega(n^2)$ time to solve. Nevertheless, quadratic programs coming from SVMs has input size $O(n)$, allowing the possibility of designing nearly-linear time algorithms. Two important classes of SVMs are programs admitting low-rank kernel factorizations and low-treewidth programs. Low-treewidth convex optimization has gained increasing interest in the past few years (e.g.~linear programming [Dong, Lee and Ye 2021] and semidefinite programming [Gu and Song 2022]). Therefore, an important open question is whether there exist nearly-linear time algorithms for quadratic programs with these nice structures. In this work, we provide the first nearly-linear time algorithm for solving quadratic programming with low-rank factorization or low-treewidth, and a small number of linear constraints. Our results imply nearly-linear time algorithms for low-treewidth or low-rank SVMs.
翻译:暂无翻译