Order Constraints in Optimal Transport

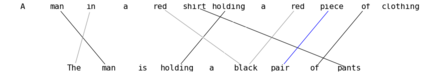
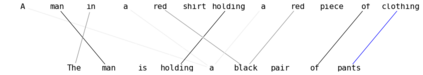
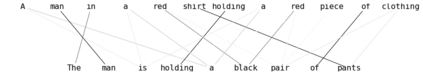
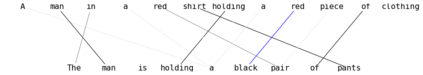
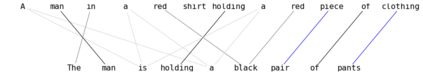
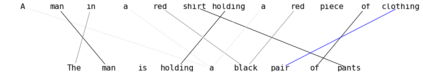
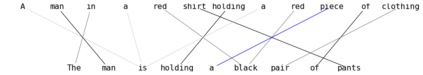
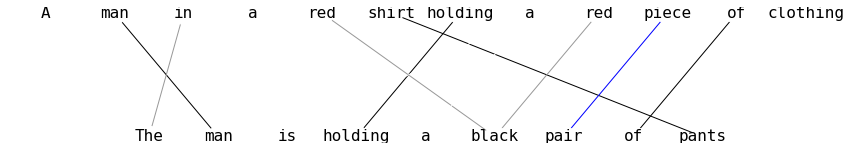
Optimal transport is a framework for comparing measures whereby a cost is incurred for transporting one measure to another. Recent works have aimed to improve optimal transport plans through the introduction of various forms of structure. We introduce novel order constraints into the optimal transport formulation to allow for the incorporation of structure. While there will are now quadratically many constraints as before, we prove a $\delta-$approximate solution to the order-constrained optimal transport problem can be obtained in $\mathcal{O}(L^2\delta^{-2} \kappa(\delta(2cL_\infty (1+(mn)^{1/2}))^{-1}) \cdot mn\log mn)$ time. We derive computationally efficient lower bounds that allow for an explainable approach to adding structure to the optimal transport plan through order constraints. We demonstrate experimentally that order constraints improve explainability using the e-SNLI (Stanford Natural Language Inference) dataset that includes human-annotated rationales for each assignment.