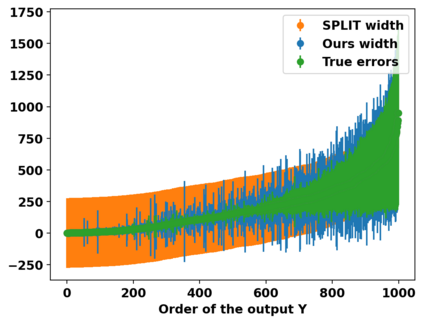
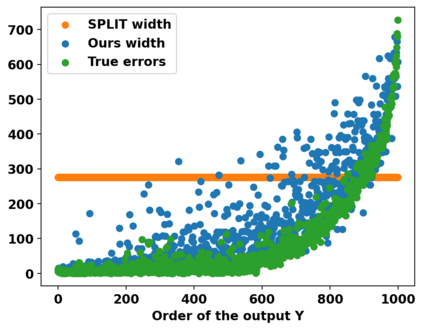
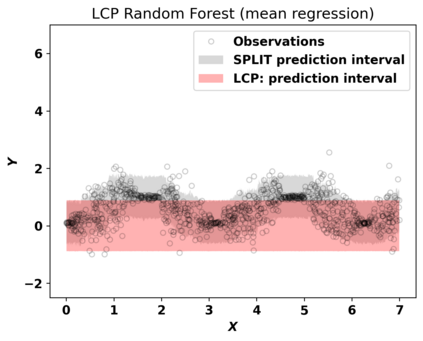
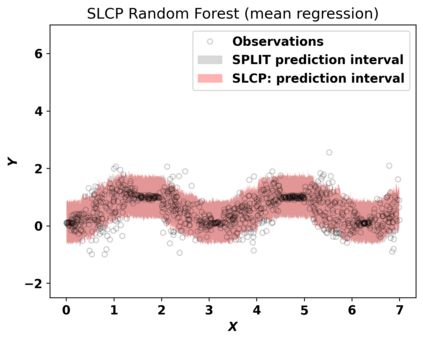
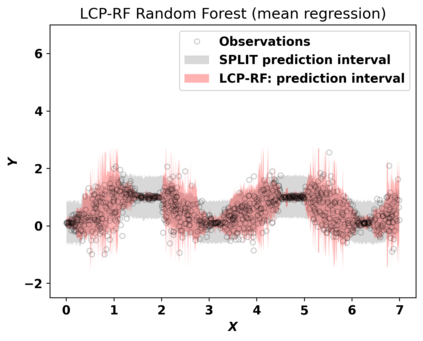
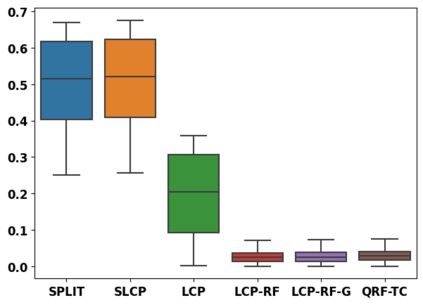
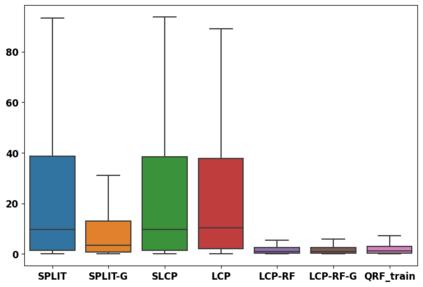
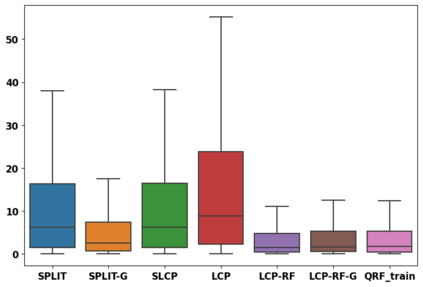
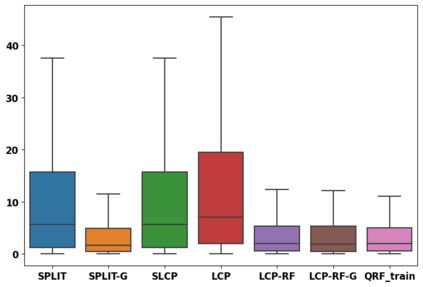
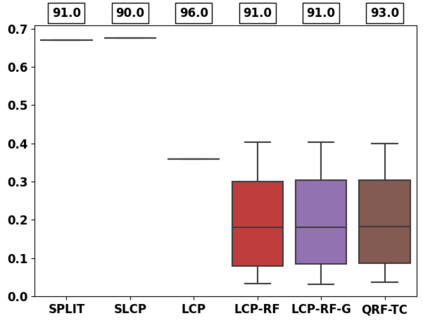
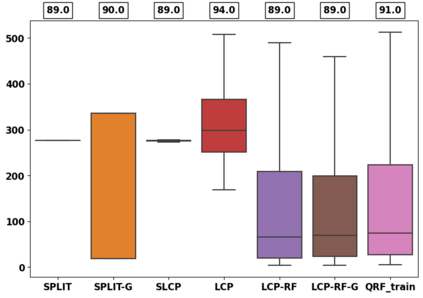
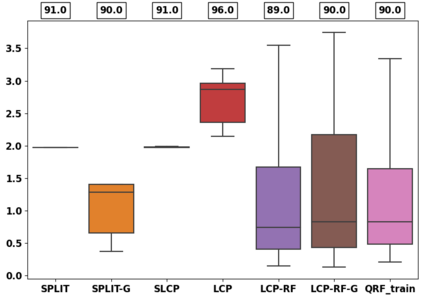
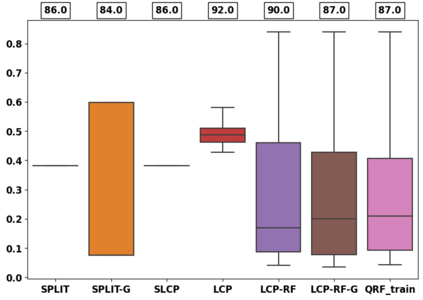
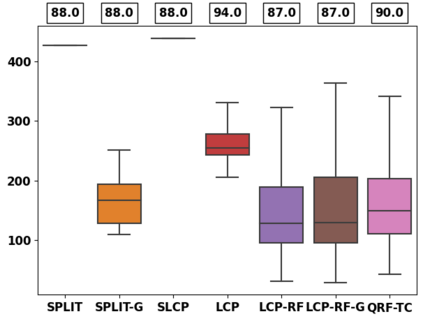
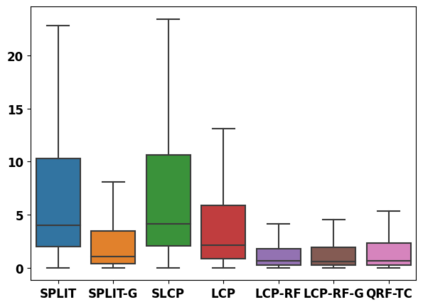
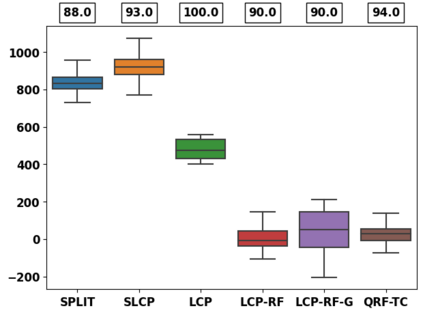
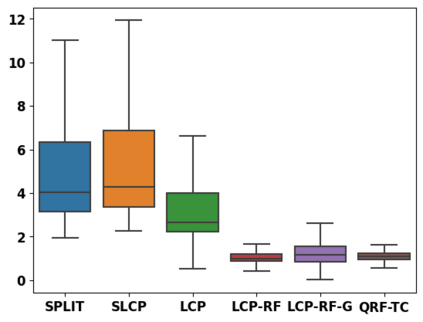
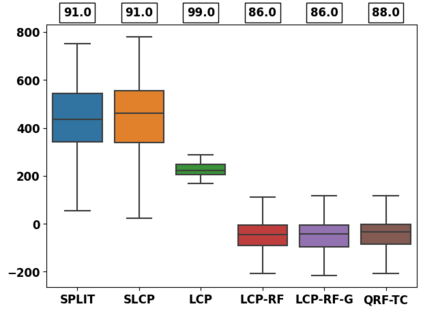
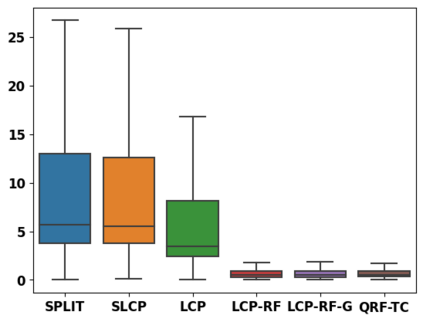
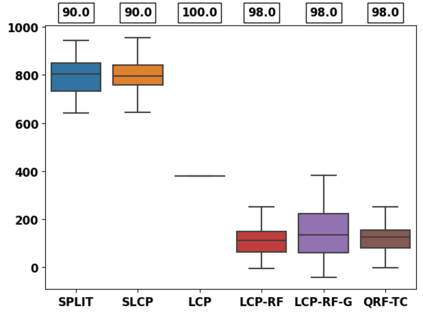
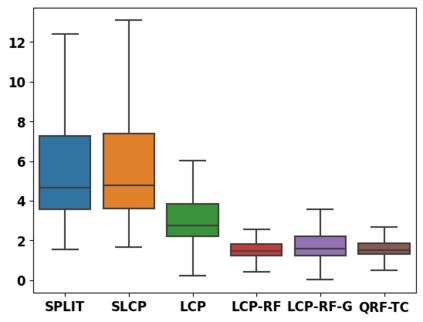
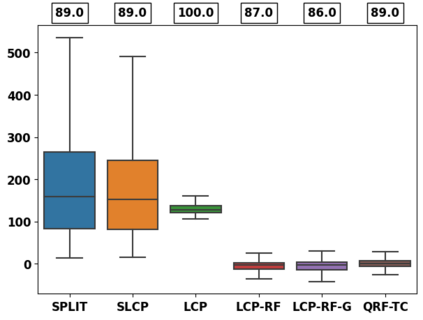
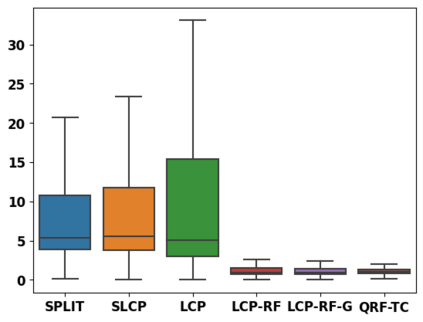
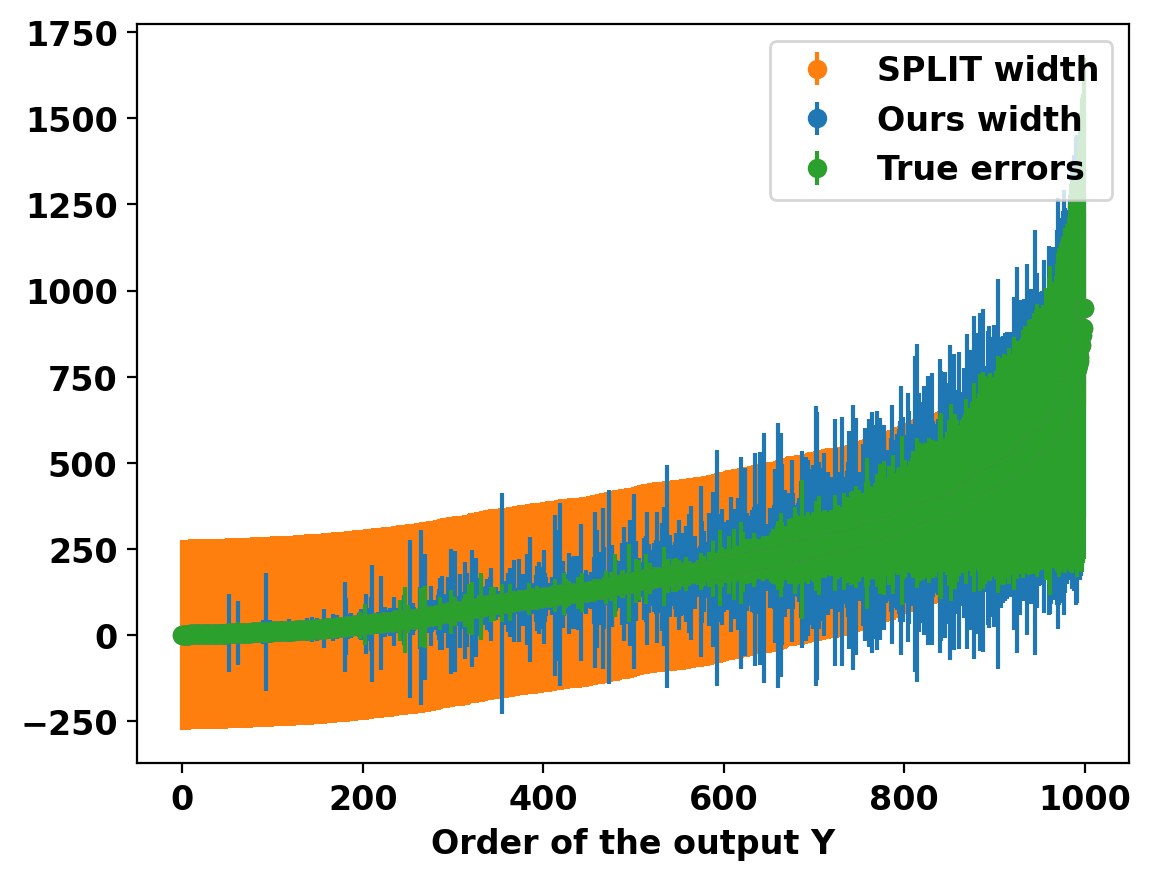
Despite attractive theoretical guarantees and practical successes, Predictive Interval (PI) given by Conformal Prediction (CP) may not reflect the uncertainty of a given model. This limitation arises from CP methods using a constant correction for all test points, disregarding their individual uncertainties, to ensure coverage properties. To address this issue, we propose using a Quantile Regression Forest (QRF) to learn the distribution of nonconformity scores and utilizing the QRF's weights to assign more importance to samples with residuals similar to the test point. This approach results in PI lengths that are more aligned with the model's uncertainty. In addition, the weights learnt by the QRF provide a partition of the features space, allowing for more efficient computations and improved adaptiveness of the PI through groupwise conformalization. Our approach enjoys an assumption-free finite sample marginal and training-conditional coverage, and under suitable assumptions, it also ensures conditional coverage. Our methods work for any nonconformity score and are available as a Python package. We conduct experiments on simulated and real-world data that demonstrate significant improvements compared to existing methods.
翻译:暂无翻译