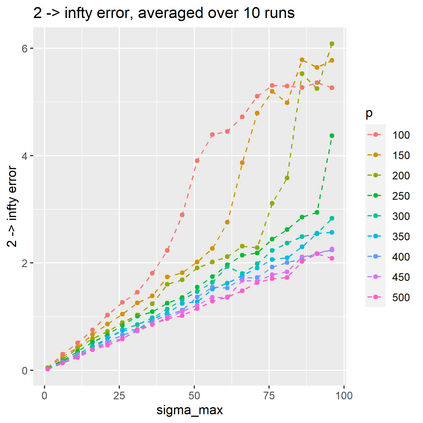
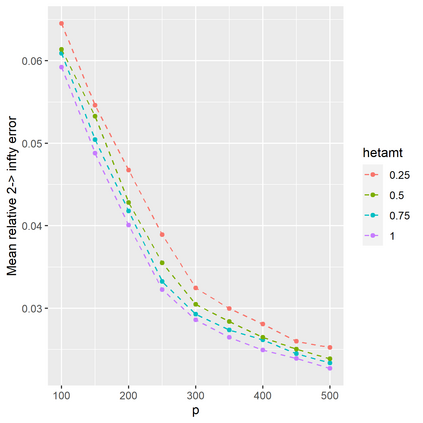
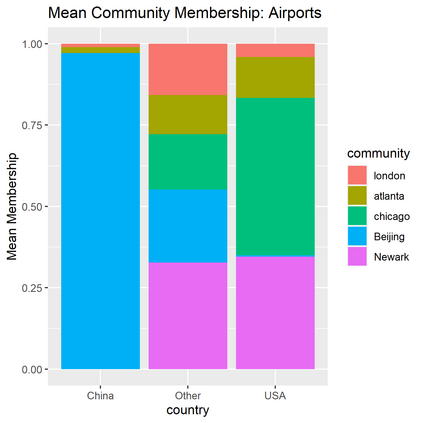
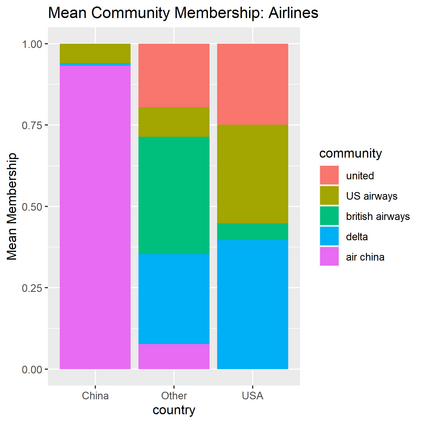
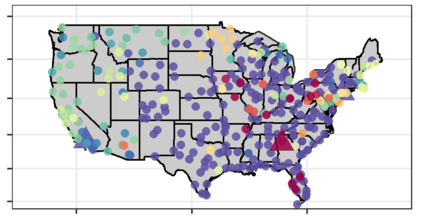
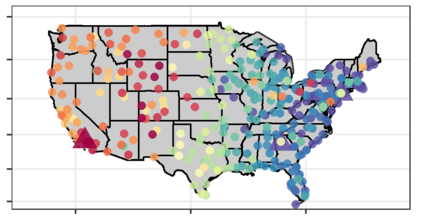
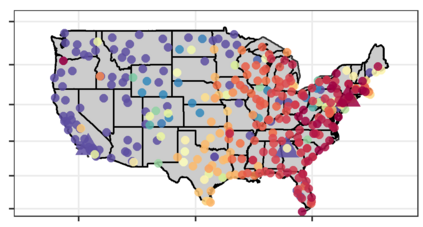
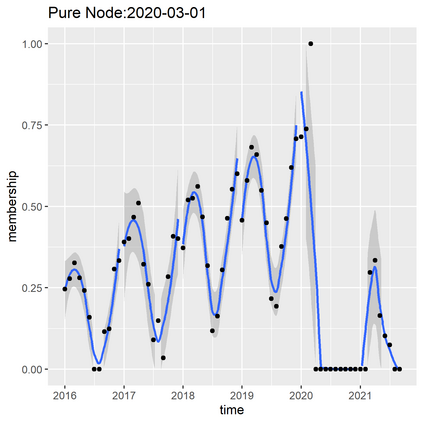
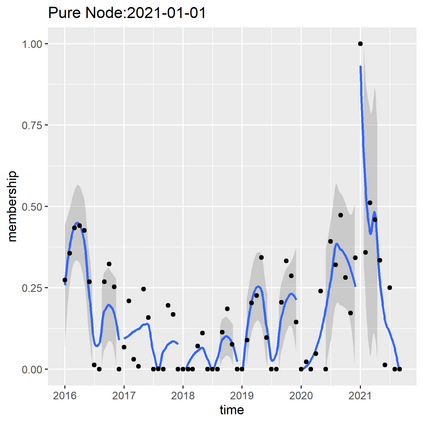
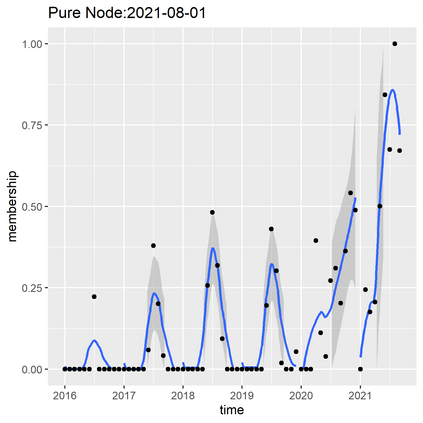
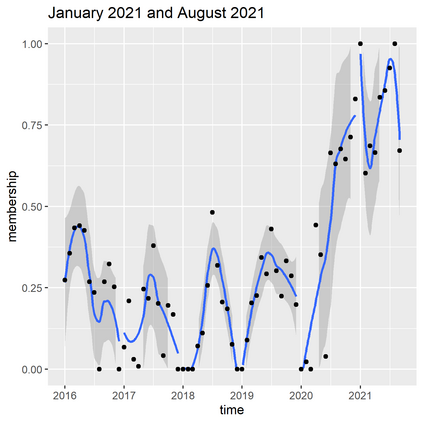
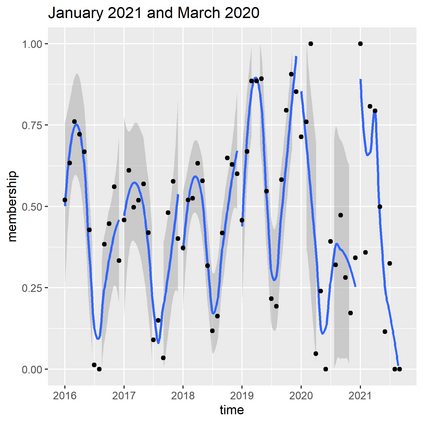
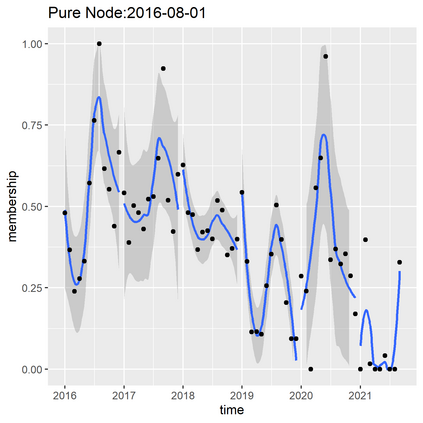
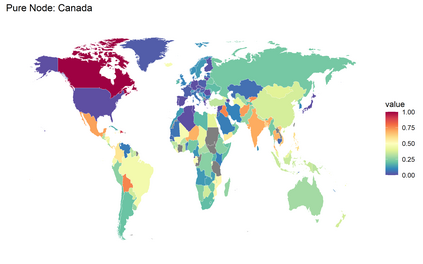
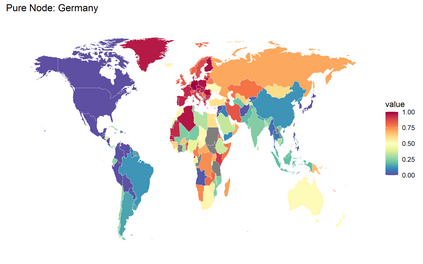
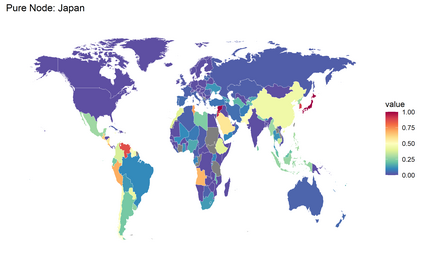
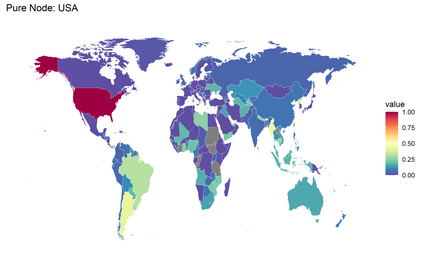
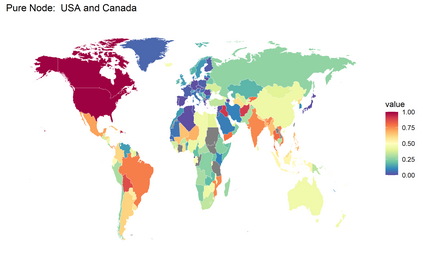
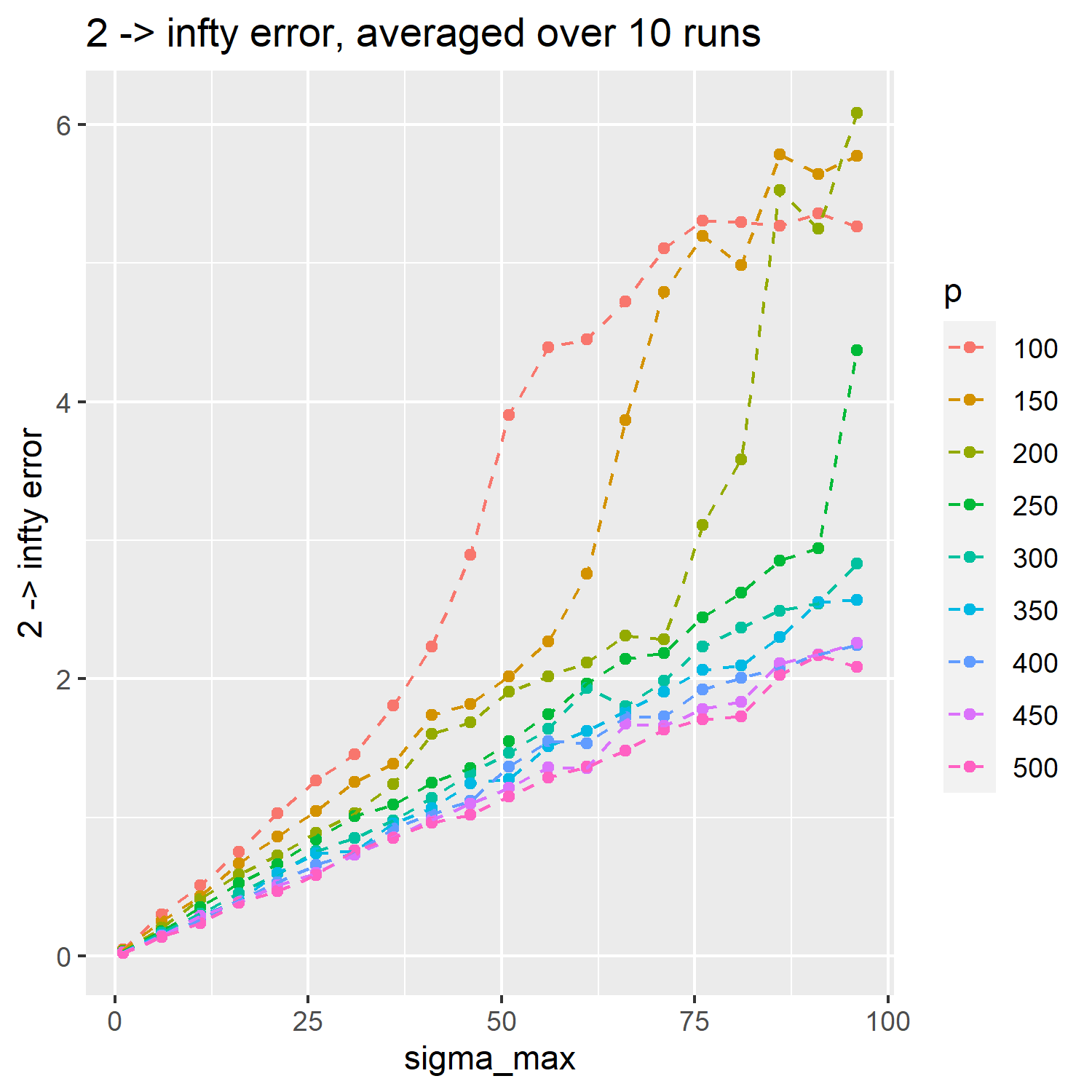
Higher-order multiway data is ubiquitous in machine learning and statistics and often exhibits community-like structures, where each component (node) along each different mode has a community membership associated with it. In this paper we propose the tensor mixed-membership blockmodel, a generalization of the tensor blockmodel positing that memberships need not be discrete, but instead are convex combinations of latent communities. We establish the identifiability of our model and propose a computationally efficient estimation procedure based on the higher-order orthogonal iteration algorithm (HOOI) for tensor SVD composed with a simplex corner-finding algorithm. We then demonstrate the consistency of our estimation procedure by providing a per-node error bound, which showcases the effect of higher-order structures on estimation accuracy. To prove our consistency result, we develop the $\ell_{2,\infty}$ tensor perturbation bound for HOOI under independent, possibly heteroskedastic, subgaussian noise that may be of independent interest. Our analysis uses a novel leave-one-out construction for the iterates, and our bounds depend only on spectral properties of the underlying low-rank tensor under nearly optimal signal-to-noise ratio conditions such that tensor SVD is computationally feasible. Whereas other leave-one-out analyses typically focus on sequences constructed by analyzing the output of a given algorithm with a small part of the noise removed, our leave-one-out analysis constructions use both the previous iterates and the additional tensor structure to eliminate a potential additional source of error. Finally, we apply our methodology to real and simulated data, including applications to two flight datasets and a trade network dataset, demonstrating some effects not identifiable from the model with discrete community memberships.
翻译:暂无翻译