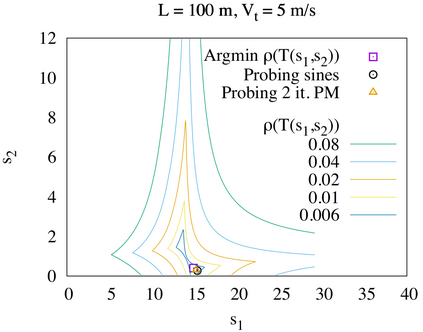
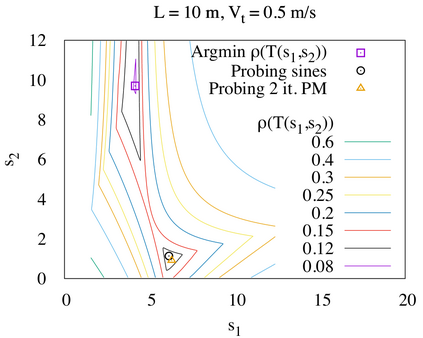
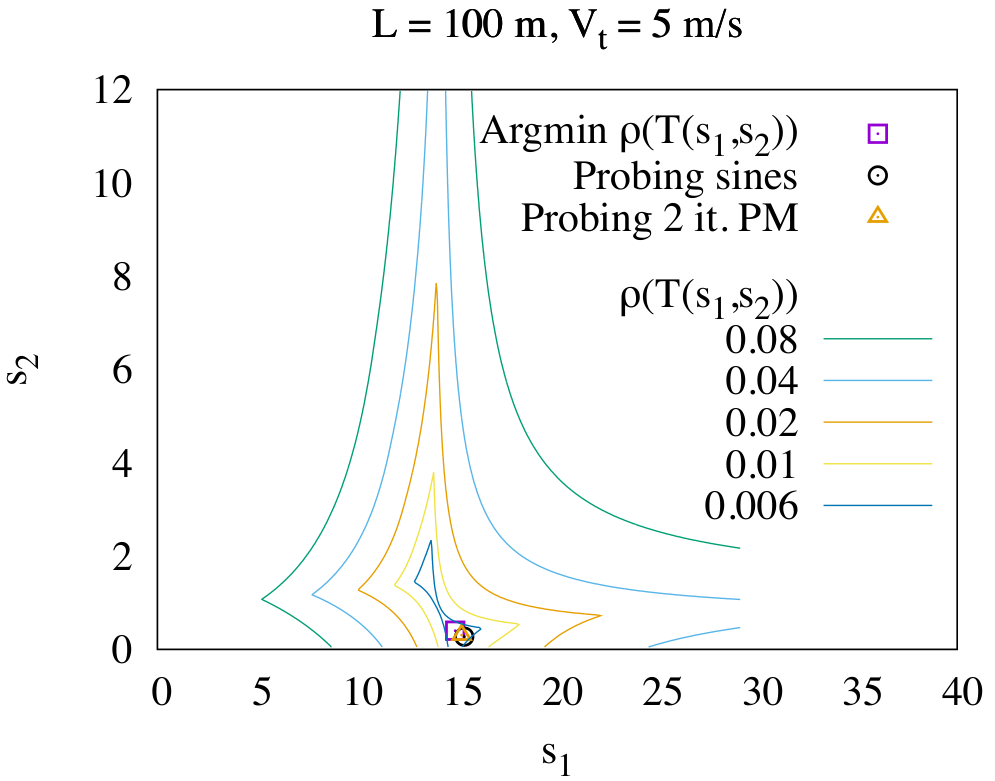
Optimized Schwarz Methods (OSMs) are based on optimized transmission conditions along the interfaces between the subdomains. Optimized transmission conditions are derived at the theoretical level, using techniques developed in the last decades. The hypothesis behind these analyses are quite strong, so that the applicability of OSMs is still limited. In this manuscript, we present a numerical algorithm to obtain optimized transmission conditions for any given problem at hand. This algorithm requires few subdomain solves to be performed in an offline phase. This additional cost is usually negligible due to the resulting faster convergence, even in a single-query context.
翻译:优化Schwarz方法(OSMS)基于子域之间界面的优化传输条件。优化传输条件是在理论层面上产生的,使用过去几十年开发的技术。这些分析背后的假设相当有力,因此OSMs的适用性仍然有限。在本手稿中,我们提出了一个数字算法,以便为手头的任何特定问题获得优化传输条件。这种算法要求在离线阶段完成的子域域解决方案很少。这种额外成本通常微不足道,因为由此导致的更快的趋同,即使是在单一孔隙。