Independent finite approximations for Bayesian nonparametric inference

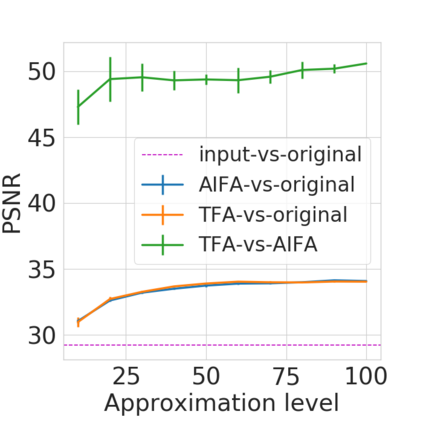
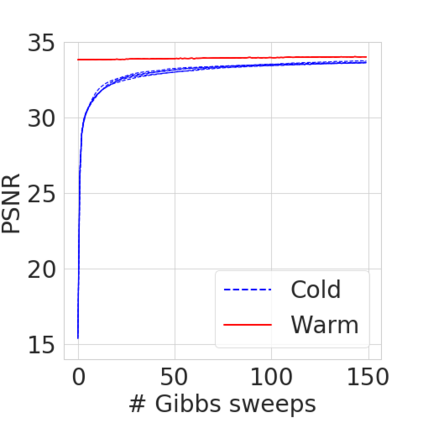
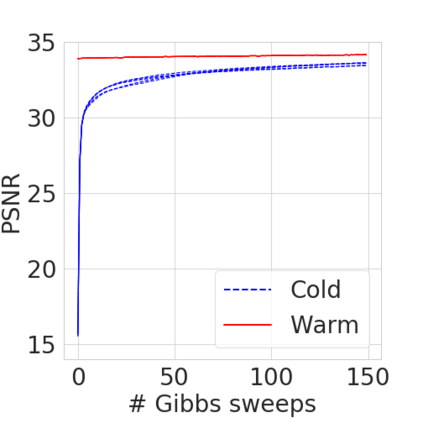
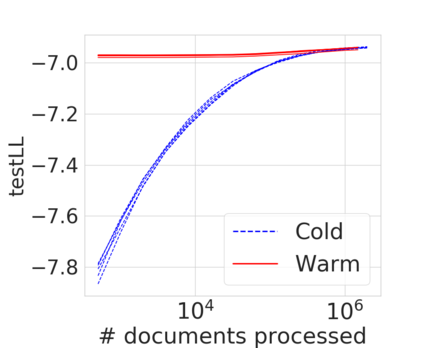
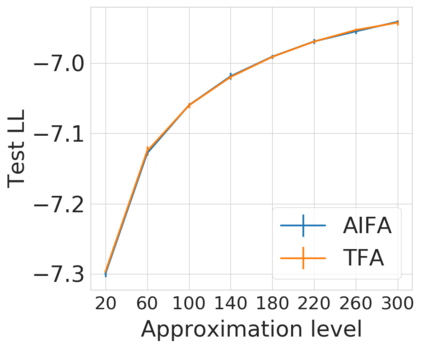
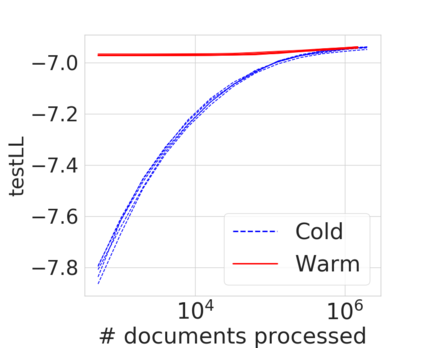
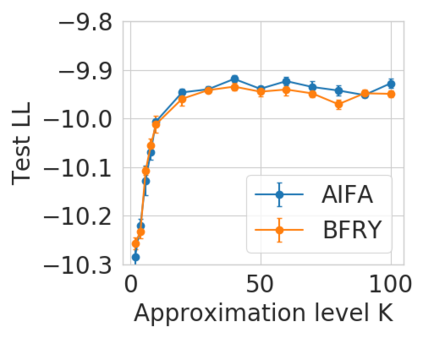
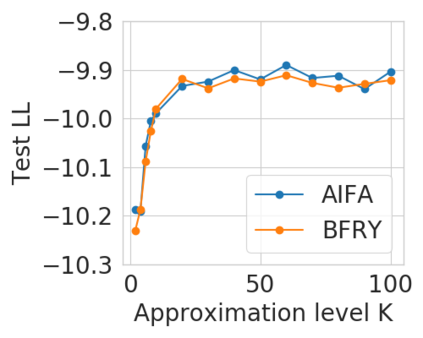
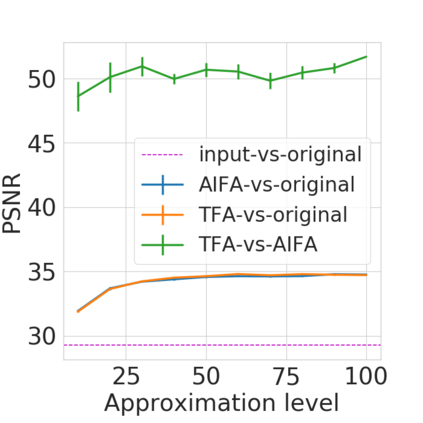
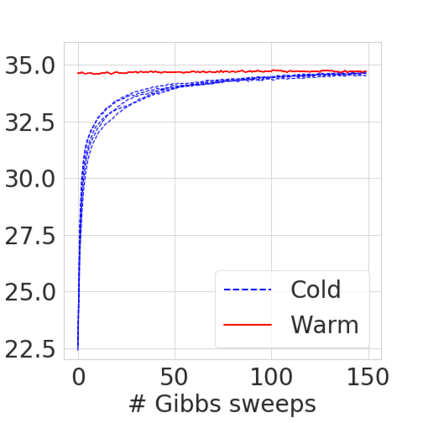
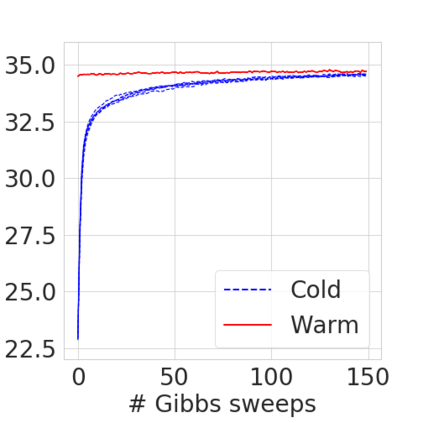
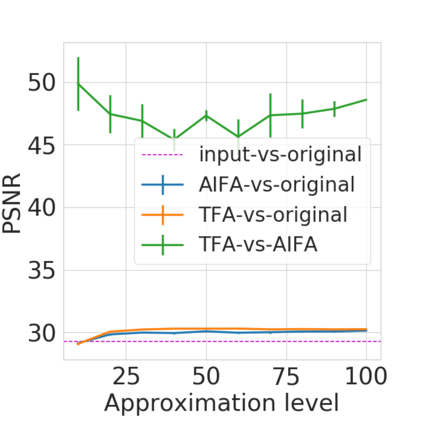
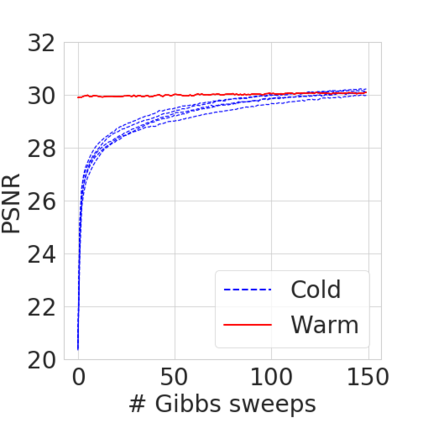
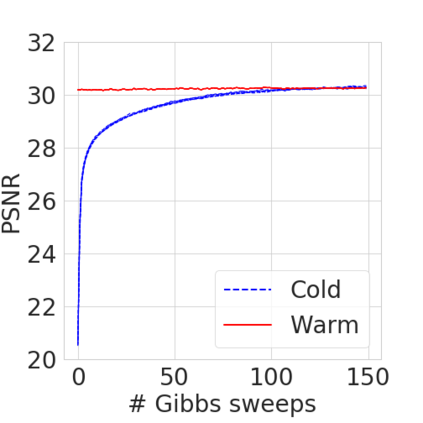
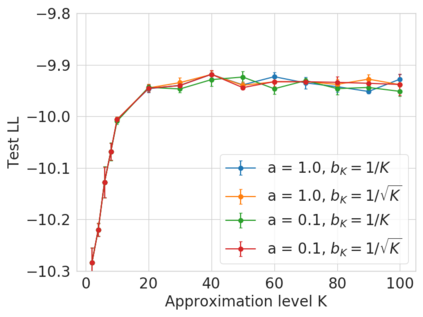
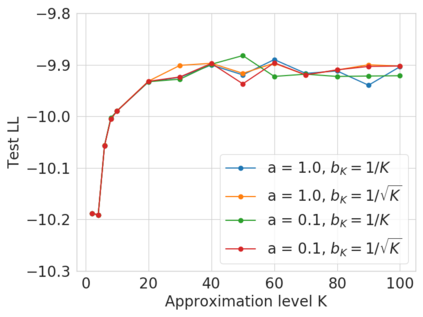
Bayesian nonparametric priors based on completely random measures (CRMs) offer a flexible modeling approach when the number of latent components in a dataset is unknown. However, managing the infinite dimensionality of CRMs typically requires practitioners to derive ad-hoc algorithms, preventing the use of general-purpose inference methods and often leading to long compute times. We propose a general but explicit recipe to construct a simple finite-dimensional approximation that can replace the infinite-dimensional CRMs. Our independent finite approximation (IFA) is a generalization of important cases that are used in practice. The independence of atom weights in our approximation (i) makes the construction well-suited for parallel and distributed computation and (ii) facilitates more convenient inference schemes. We quantify the approximation error between IFAs and the target nonparametric prior. We compare IFAs with an alternative approximation scheme -- truncated finite approximations (TFAs), where the atom weights are constructed sequentially. We prove that, for worst-case choices of observation likelihoods, TFAs are a more efficient approximation than IFAs. However, in real-data experiments with image denoising and topic modeling, we find that IFAs perform very similarly to TFAs in terms of task-specific accuracy metrics.