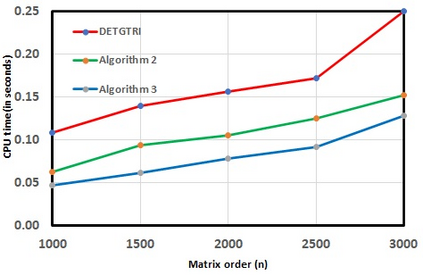
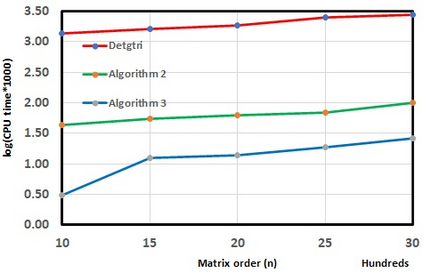
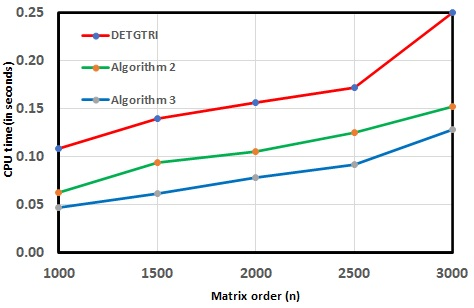
The principal minors of a tridiagonal matrix satisfy two-term and three-term recurrences [1, 2]. Based on these facts, the current article presents a new efficient and reliable hybrid numerical algorithm for evaluating general n-th order tridiagonal determinants in linear time. The hybrid numerical algorithm avoid all symbolic computations. The algorithm is suited for implementation using computer languages such as FORTRAN, PASCAL, ALGOL, MAPLE, MACSYMA and MATHEMATICA. Some illustrative examples are given. Test results indicate the superiority of the hybrid numerical algorithm.
翻译：根据这些事实,本条款提出了一种新的高效可靠的混合数字算法,用于在线性时间评价一般n-n-th顺序的三对形决定因素。混合数字算法避免所有象征性计算。这种算法适合于使用计算机语言实施,如ForTRAN、PASAL、ALGOL、MAPLE、MACSYMA和MAtheMATICA。提供了一些示例。测试结果表明混合数字算法的优越性。
相关内容
微信扫码咨询专知VIP会员