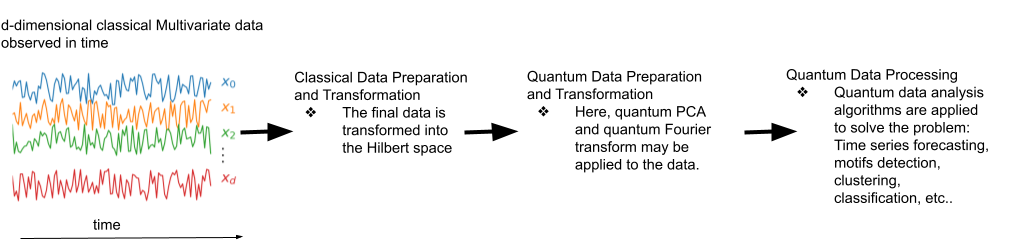
Because of the rotational components on quantum circuits, some quantum neural networks based on variational circuits can be considered equivalent to the classical Fourier networks. In addition, they can be used to predict Fourier coefficients of continuous functions. Time series data indicates a state of a variable in time. Since some time series data can be also considered as continuous functions, we can expect quantum machine learning models to do do many data analysis tasks successfully on time series data. Therefore, it is important to investigate new quantum logics for temporal data processing and analyze intrinsic relationships of data on quantum computers. In this paper, we go through the quantum analogues of classical data preprocessing and forecasting with ARIMA models by using simple quantum operators requiring a few number of quantum gates. Then we discuss future directions and some of the tools/algorithms that can be used for temporal data analysis on quantum computers.
翻译:由于量子电路的旋转部件,一些基于变换电路的量子神经网络可以被视为等同于古典Fourier网络。此外,它们还可以用来预测连续函数的Fourier系数。时间序列数据表明一个时间变量的状态。由于某些时间序列数据也可以被视为连续函数,我们可以期望量子机器学习模型能够成功地完成时间序列数据方面的许多数据分析任务。因此,必须调查用于时间数据处理的新的量子逻辑,分析量子计算机数据的内在关系。在本文中,我们通过使用需要少数量子门的简单量子操作器对古典数据预处理和预测模型进行量子模拟。然后我们讨论未来的方向和一些可用于量子计算机时间数据分析的工具/算法。
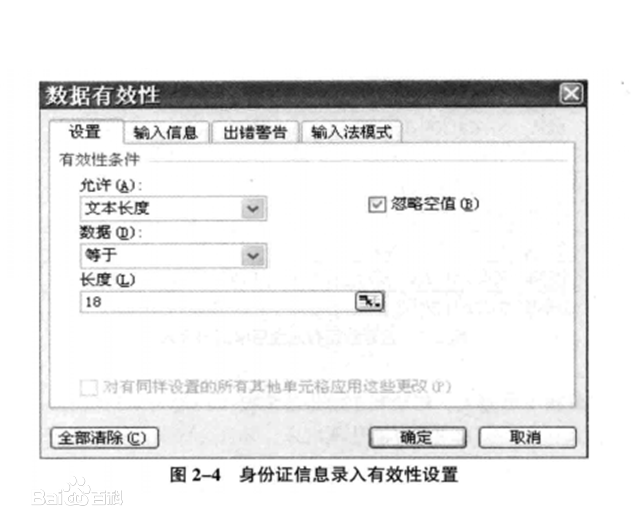
相关内容
Source: Apple - iOS 8