ROM inversion of monostatic data lifted to full MIMO

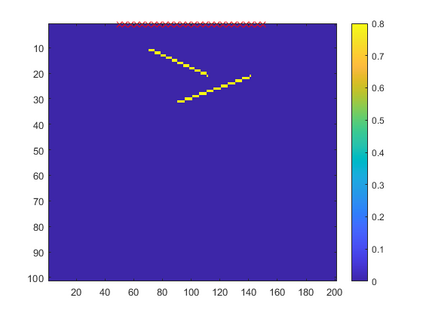
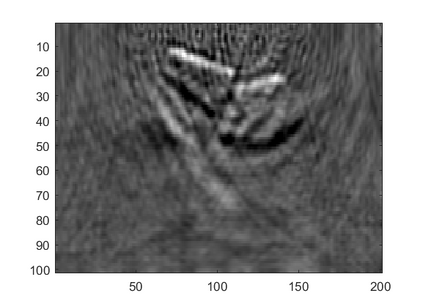
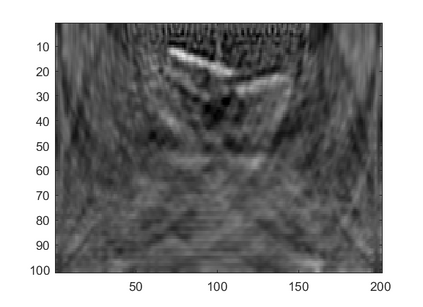
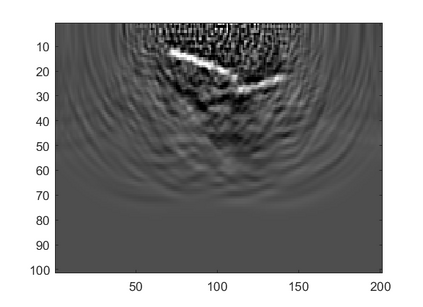
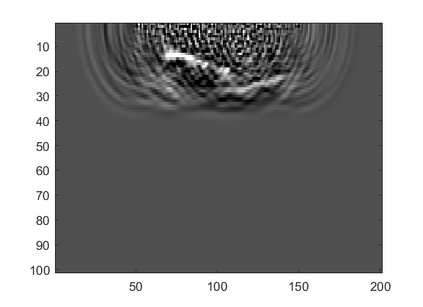
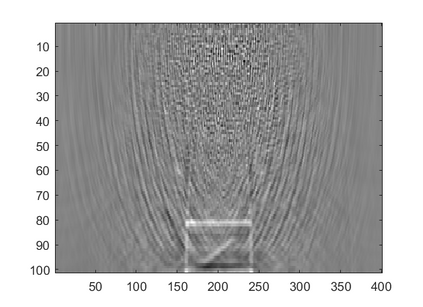
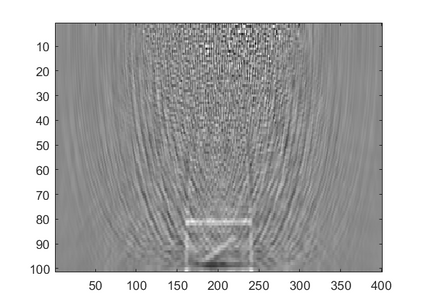
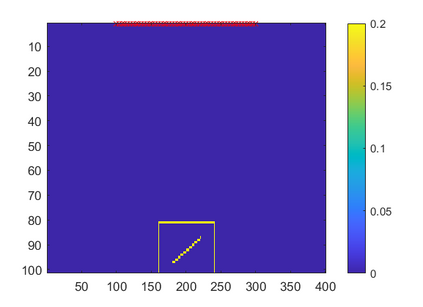
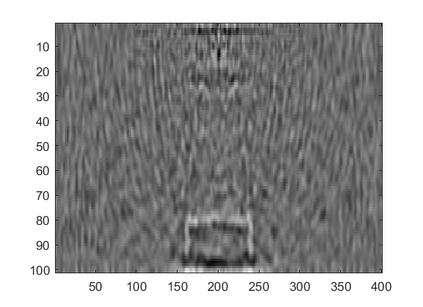
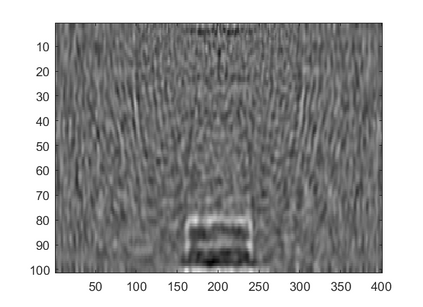
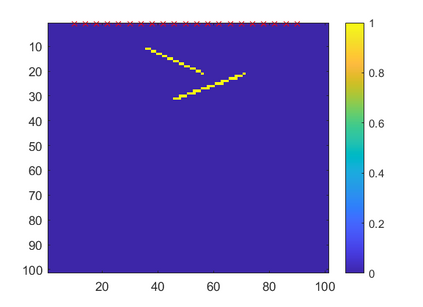
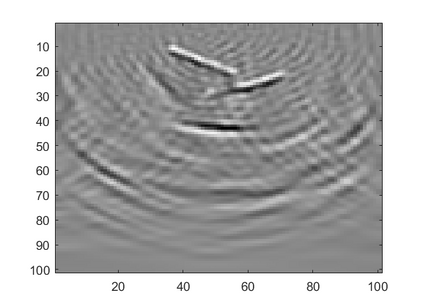
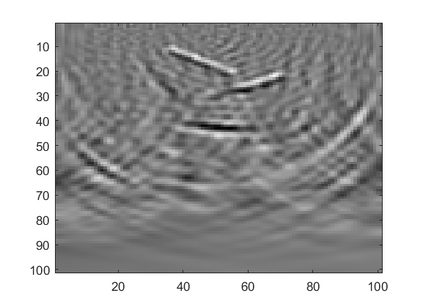
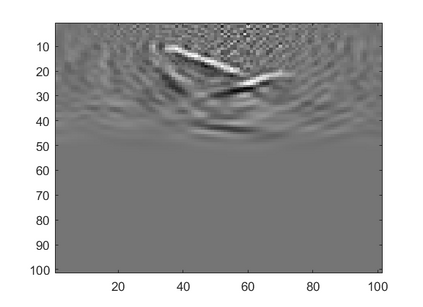
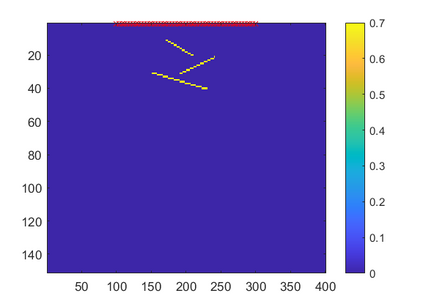
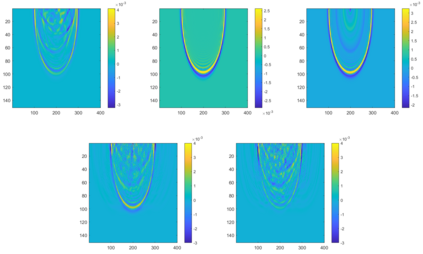
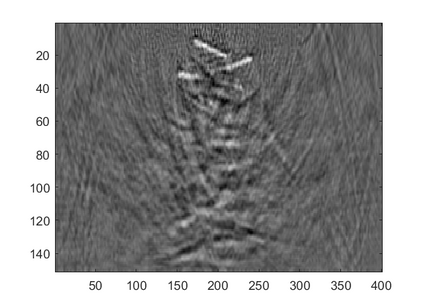
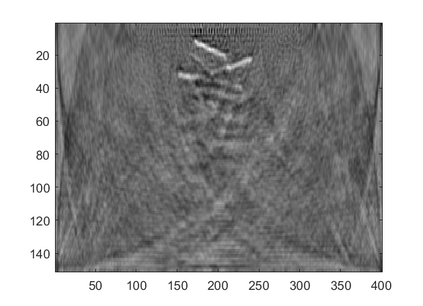
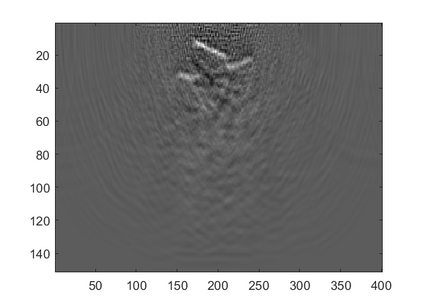
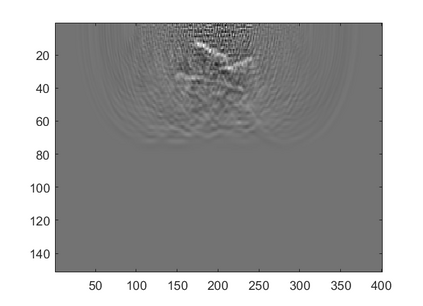
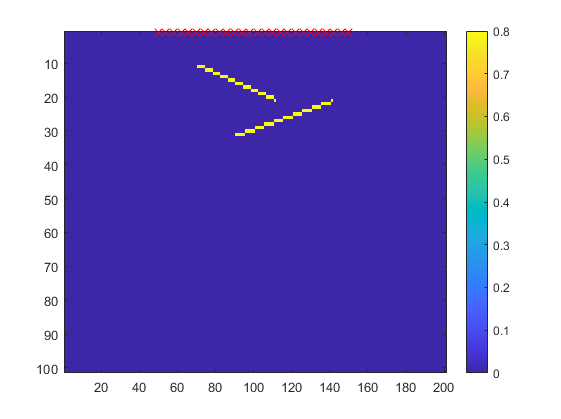
The Lippmann--Schwinger--Lanczos (LSL) algorithm has recently been shown to provide an efficient tool for imaging and direct inversion of synthetic aperture radar data in multi-scattering environments [17], where the data set is limited to the monostatic, a.k.a. single input/single output (SISO) measurements. The approach is based on constructing data-driven estimates of internal fields via a reduced-order model (ROM) framework and then plugging them into the Lippmann-Schwinger integral equation. However, the approximations of the internal solutions may have more error due to missing the off diagonal elements of the multiple input/multiple output (MIMO) matrix valued transfer function. This, in turn, may result in multiple echoes in the image. Here we present a ROM-based data completion algorithm to mitigate this problem. First, we apply the LSL algorithm to the SISO data as in [17] to obtain approximate reconstructions as well as the estimate of internal field. Next, we use these estimates to calculate a forward Lippmann-Schwinger integral to populate the missing off-diagonal data (the lifting step). Finally, to update the reconstructions, we solve the Lippmann-Schwinger equation using the original SISO data, where the internal fields are constructed from the lifted MIMO data. The steps of obtaining the approximate reconstructions and internal fields and populating the missing MIMO data entries can be repeated for complex models to improve the images even further. Efficiency of the proposed approach is demonstrated on 2D and 2.5D numerical examples, where we see reconstructions are improved substantially.