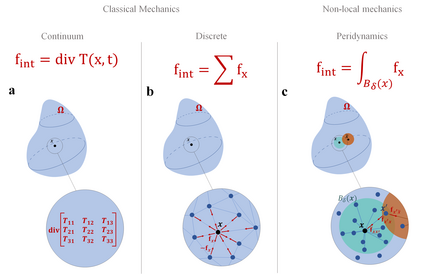
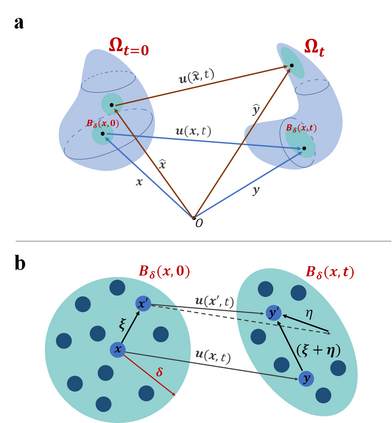
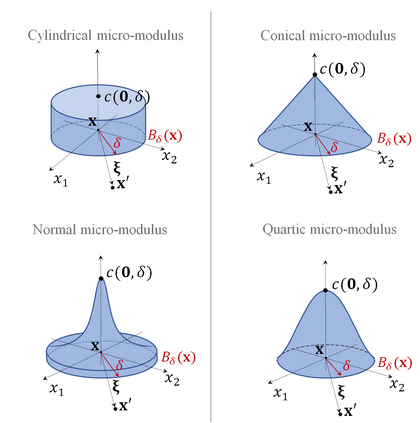
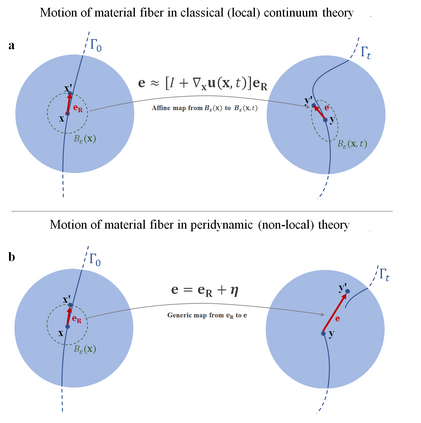
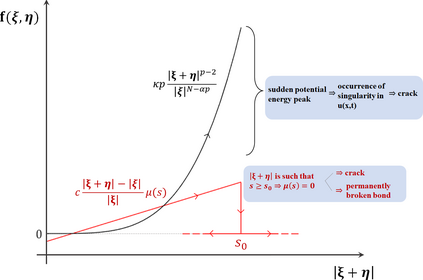
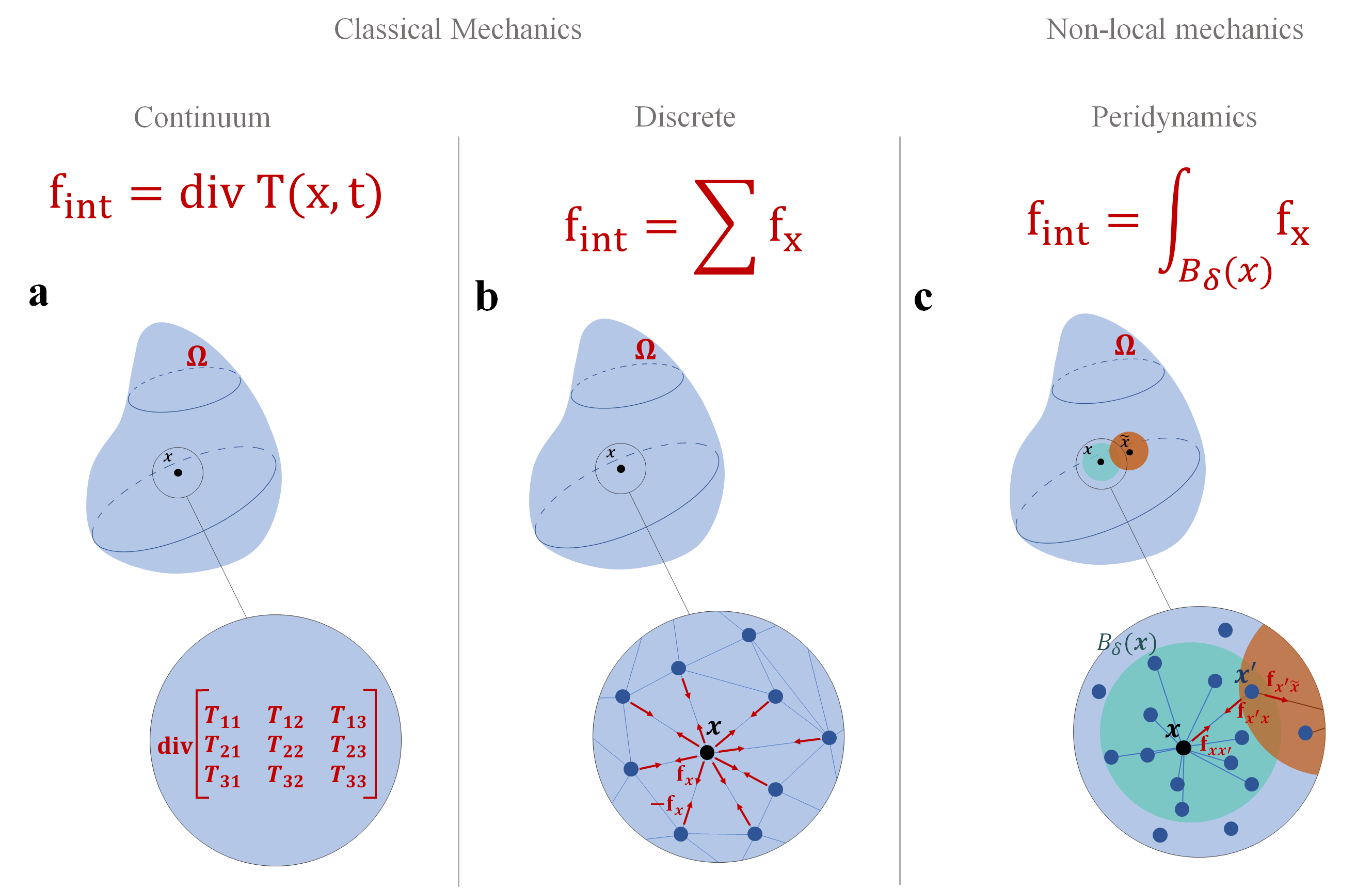
Peridynamic (PD) theories have gained widespread diffusion among various research areas, due to the ability of modeling discontinuities formation and evolution in materials. Bond-Based Peridynamics (BB-PD), notwithstanding some modeling limitations, is widely employed in numerical simulations, due to its easy implementation combined with physical intuitiveness and stability. In the present paper, several aspects of bond-based peridynamic models have been reviewed and investigated. A detailed description of peridynamics theory, applications and numerical models has been presented. Employed BB-PD integral kernels have been displayed, together with their differences and commonalities; then, some consequences of their mathematical structure have been discussed. The kinematic role of nonlocality, the relation between kernel structure and material impenetrability, and the role of PD kernel nonlinearity in cracks formation prediction have been critically analyzed and commented. Finally the idea of extending BB-PD to fluids is proposed and presented in the framework of fading memory material, drawing some perspectives for a deeper and more comprehensive understanding of the peridynamics in fluids.
翻译:由于模拟不连续形成和材料进化的能力,各种研究领域已广泛扩散了潜动力学(PD)理论。尽管存在一些建模限制,但BB-PD(BB-PD)在数字模拟中被广泛采用,因为其实施容易,而且物理直观性和稳定性也比较稳定。在本文件中,对基于债券的潜动力学模型的若干方面进行了审查和调查。提出了关于潜动力学理论、应用和数字模型的详细描述。使用的BBB-PD综合内核及其差异和共性已经展示;然后,讨论了其数学结构的一些后果。非地性、内核结构和材料不易性之间的关系以及PD内核无线性在裂缝形成预测中的作用,已经进行了严格分析和评论。最后,在消化记忆材料的框架内提出并提出了将BB-PD扩大到液体的想法,为更深入、更全面地了解流体动力学提供了一些观点。