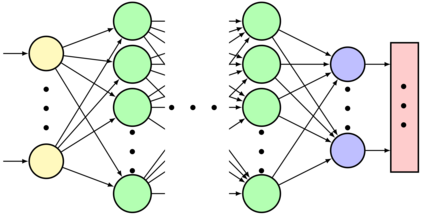
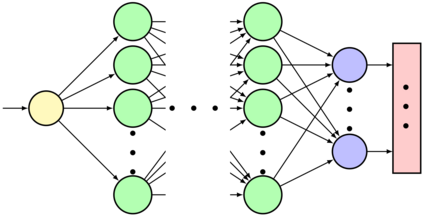
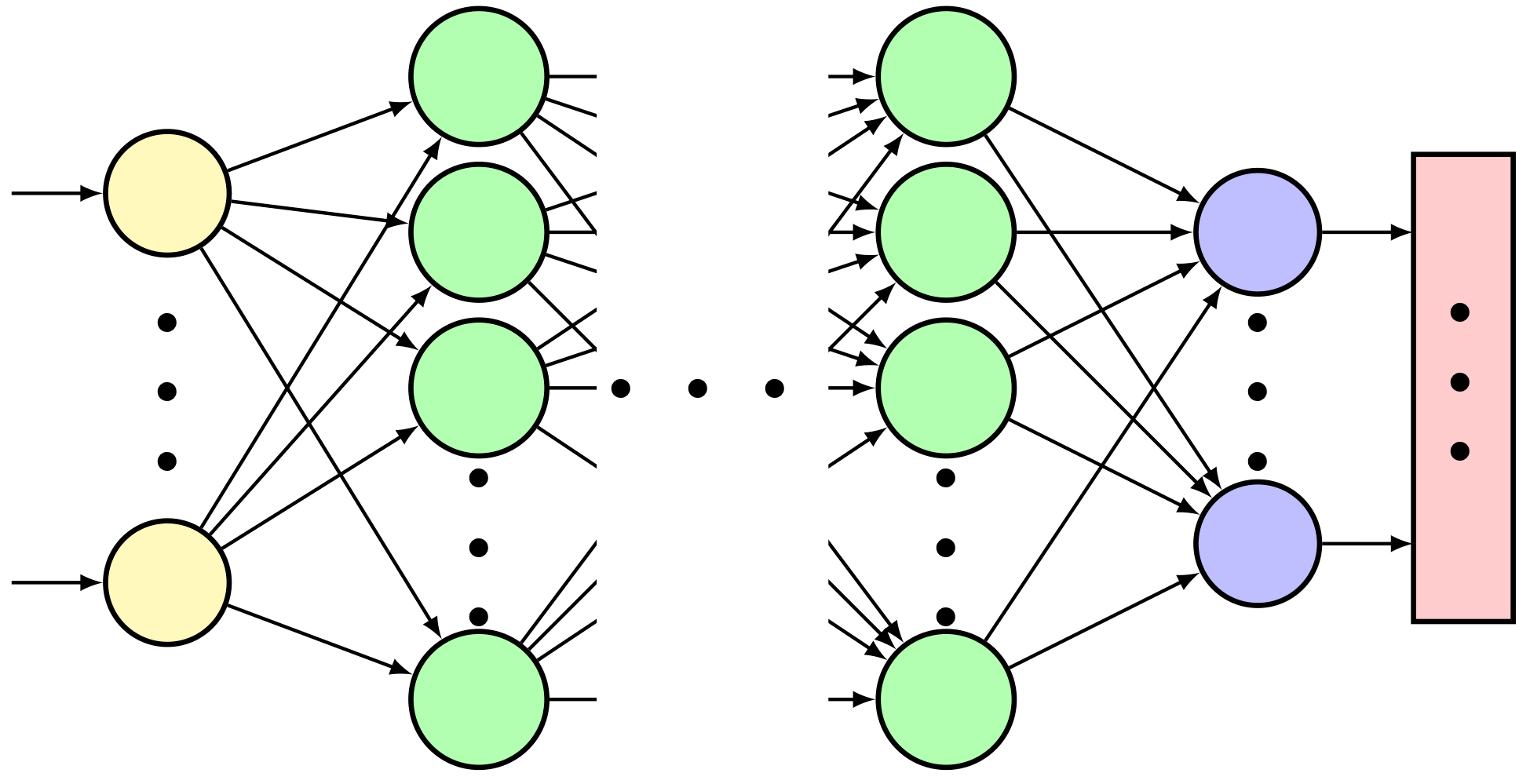
We introduce a novel deep operator network (DeepONet) framework that incorporates generalised variational inference (GVI) using R\'enyi's $\alpha$-divergence to learn complex operators while quantifying uncertainty. By incorporating Bayesian neural networks as the building blocks for the branch and trunk networks, our framework endows DeepONet with uncertainty quantification. The use of R\'enyi's $\alpha$-divergence, instead of the Kullback-Leibler divergence (KLD), commonly used in standard variational inference, mitigates issues related to prior misspecification that are prevalent in Variational Bayesian DeepONets. This approach offers enhanced flexibility and robustness. We demonstrate that modifying the variational objective function yields superior results in terms of minimising the mean squared error and improving the negative log-likelihood on the test set. Our framework's efficacy is validated across various mechanical systems, where it outperforms both deterministic and standard KLD-based VI DeepONets in predictive accuracy and uncertainty quantification. The hyperparameter $\alpha$, which controls the degree of robustness, can be tuned to optimise performance for specific problems. We apply this approach to a range of mechanics problems, including gravity pendulum, advection-diffusion, and diffusion-reaction systems. Our findings underscore the potential of $\alpha$-VI DeepONet to advance the field of data-driven operator learning and its applications in engineering and scientific domains.
翻译:暂无翻译