Topological Universality of the Art Gallery Problem

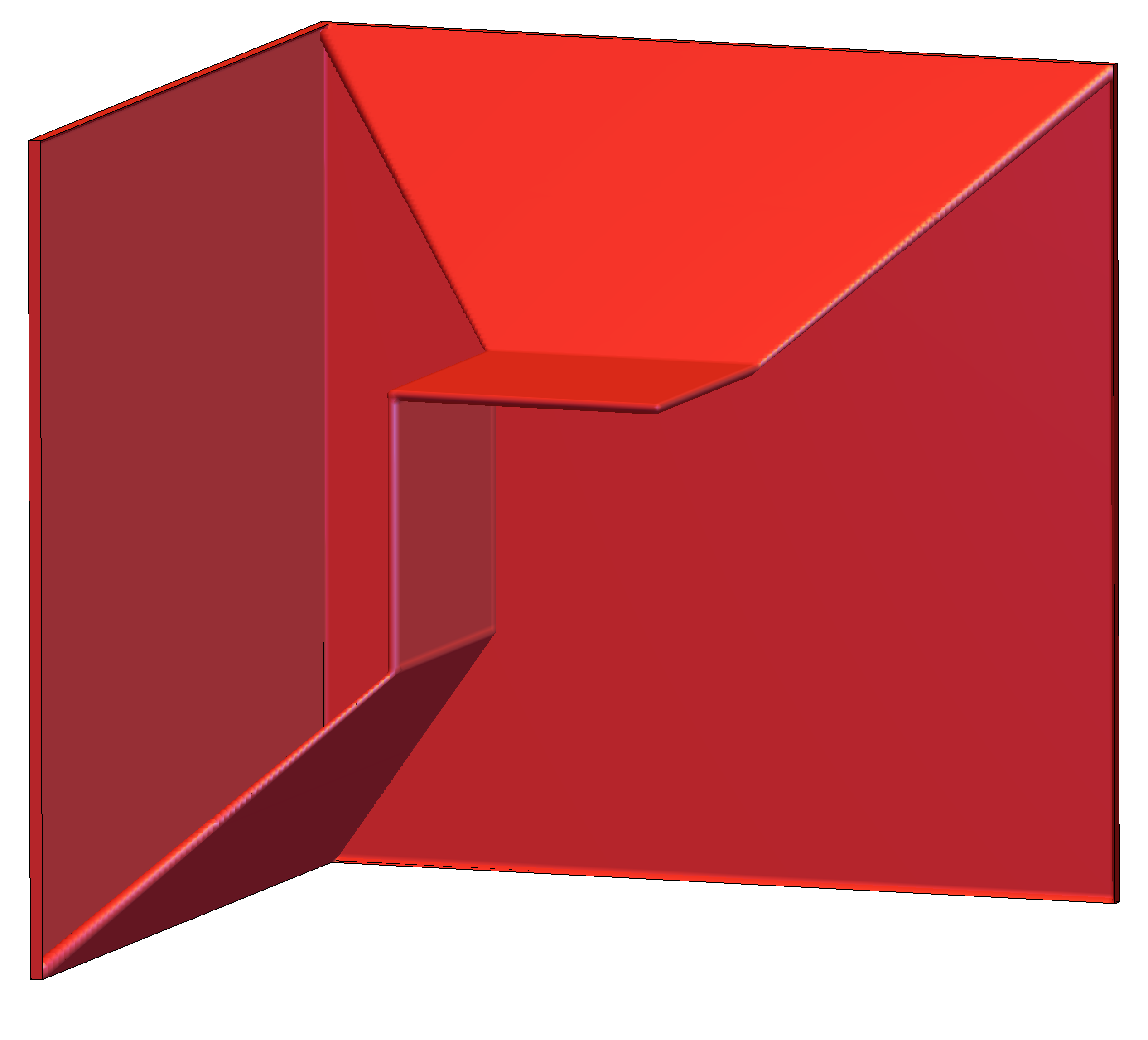
We prove that any compact semi-algebraic set is homeomorphic to the solution space of some art gallery problem. Previous works have established similar universality theorems, but holding only up to homotopy equivalence, rather than homeomorphism, and prior to this work, the existence of art galleries even for simple spaces such as the M\"obius strip or the three-holed torus were unknown. Our construction relies on an elegant and versatile gadget to copy guard positions with minimal overhead, and is thus simpler than previous constructions, consisting of a single rectangular room with convex slits cut out from the edges. We additionally show that both the orientable and non-orientable surfaces of genus $n$ require galleries with only $O(n)$ vertices.