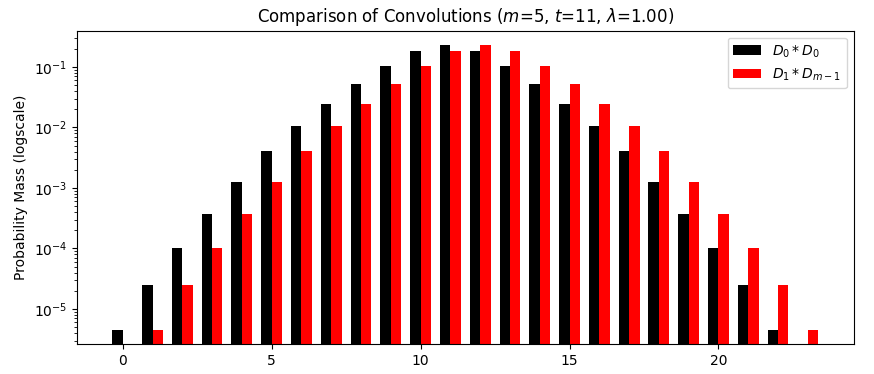
Recent work in differential privacy has explored the prospect of combining local randomization with a secure intermediary. Specifically, there are a variety of protocols in the secure shuffle model (where an intermediary randomly permutes messages) as well as the secure aggregation model (where an intermediary adds messages). Most of these protocols are limited to approximate differential privacy. An exception is the shuffle protocol by Ghazi, Golowich, Kumar, Manurangsi, Pagh, and Velingker (arXiv:2002.01919): it computes bounded sums under pure differential privacy. Its additive error is $\tilde{O}(1/\varepsilon^{3/2})$, where $\varepsilon$ is the privacy parameter. In this work, we give a new protocol that ensures $O(1/\varepsilon)$ error under pure differential privacy. We also show how to use it to test uniformity of distributions over $[d]$. The tester's sample complexity has an optimal dependence on $d$. Our work relies on a novel class of secure intermediaries which are of independent interest.
翻译:不同隐私的近期工作探索了将本地随机化与安全中介结合的前景。 具体地说, 安全洗牌模式( 中间随机移动信息) 以及安全汇总模式( 中间人添加信息 ) 中有许多协议( 中间移动信息 ) 和安全汇总模式( 中间移动信息 ) 。 这些协议大多局限于近似差异隐私 。 一个例外是 Ghazi、 Gololowich、 Kumar、 Manurangsi、 Pagh 和 Velingker 的洗牌协议( ar Xiv: 2002. 01919): 它在纯差异隐私下计算受约束的金额。 它的添加错误是 $\ tilde{O} ( 1/\ varepsilon\ 3/2} $ ) 。 它的添加错误是 $\ varepslon 是隐私参数 。 在这项工作中, 我们给出了一个新的协议, 保证在纯差异隐私下 $( 1/\ varepsilon) ) 错误。 我们还演示如何使用它来测试 $ 。 。 。 的样本复杂度对 $ 的配置对 美元有最佳依赖 $ 。 我们的工作依赖 。