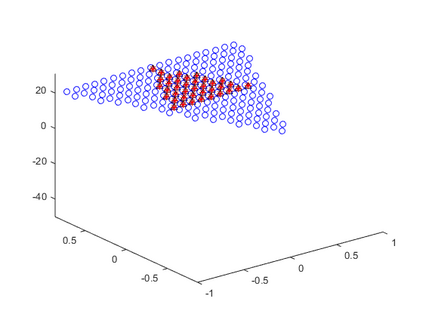
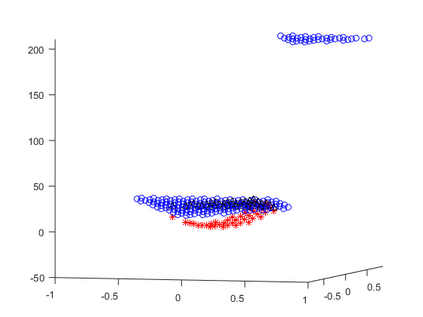
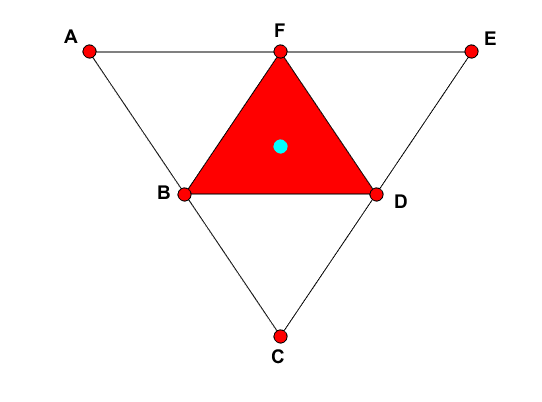
In this paper we introduce a new nonlinear reconstruction operator over two dimensional triangularized domains with equilateral triangles. We focus on the local definition of the operator. The ideas behind this definition come from some basic properties of the Harmonic mean of three positive values. We prove some results regarding the approximation properties of the operator and we carry out some numerical tests giving evidence of the avoidance of any Gibbs effects.
翻译:在本文中,我们介绍一个新的非线性重建操作员,负责两个多维三角域,并带有等边三角形。我们侧重于操作员的本地定义。这个定义背后的想法来自三个正值的调和平均值的某些基本特性。我们证明了操作员近似特性的一些结果,我们进行了一些数字测试,以证明避免了任何Gibbs效应。
相关内容
Arxiv
0+阅读 · 2022年4月14日
Arxiv
15+阅读 · 2021年4月12日