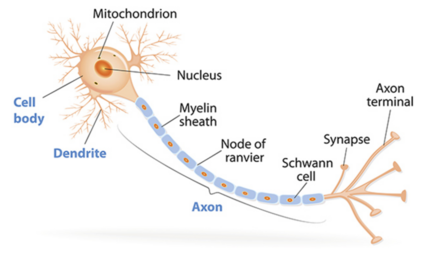
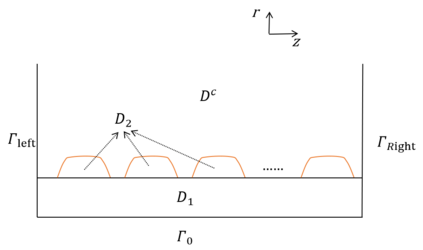
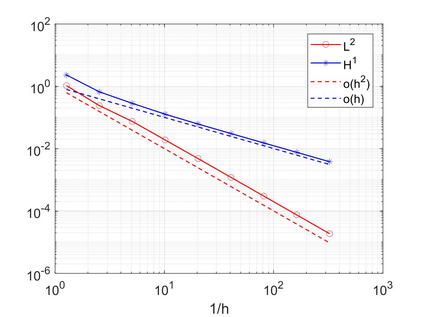
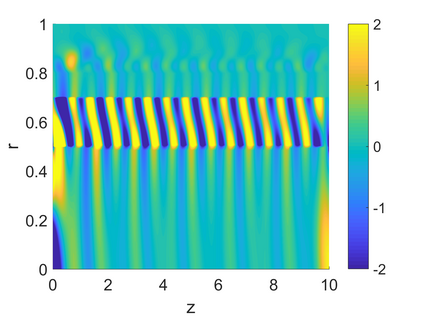
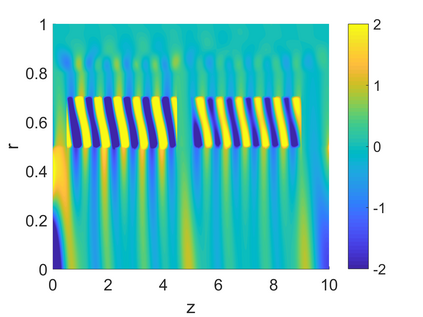
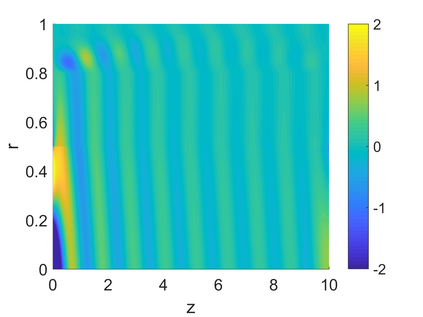
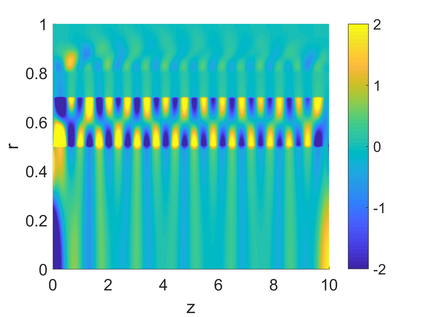
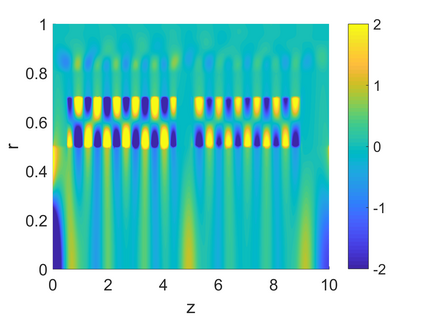
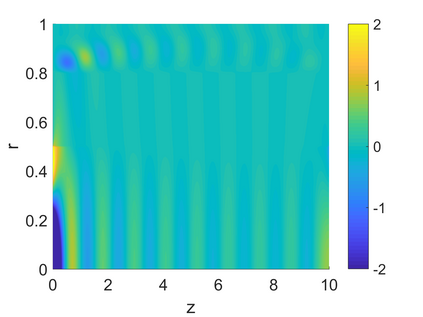
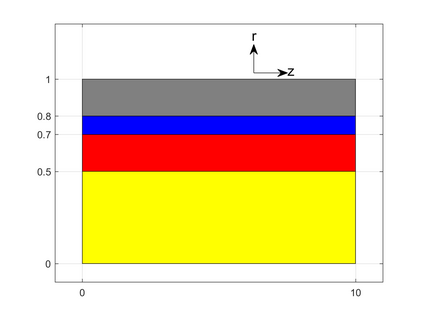
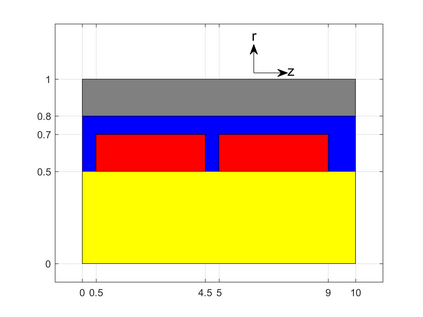
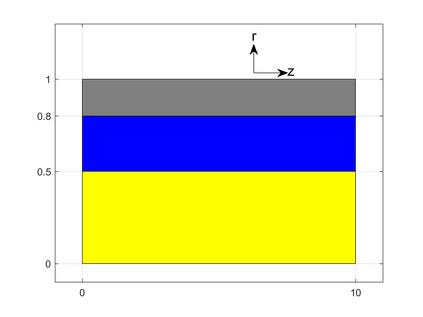
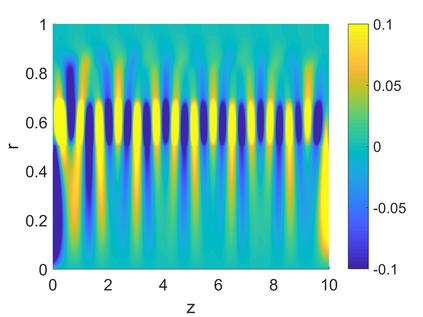
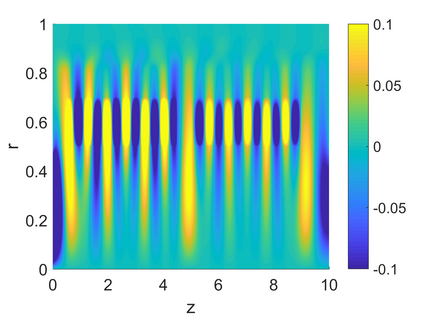
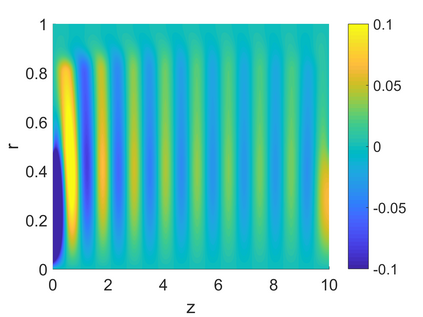
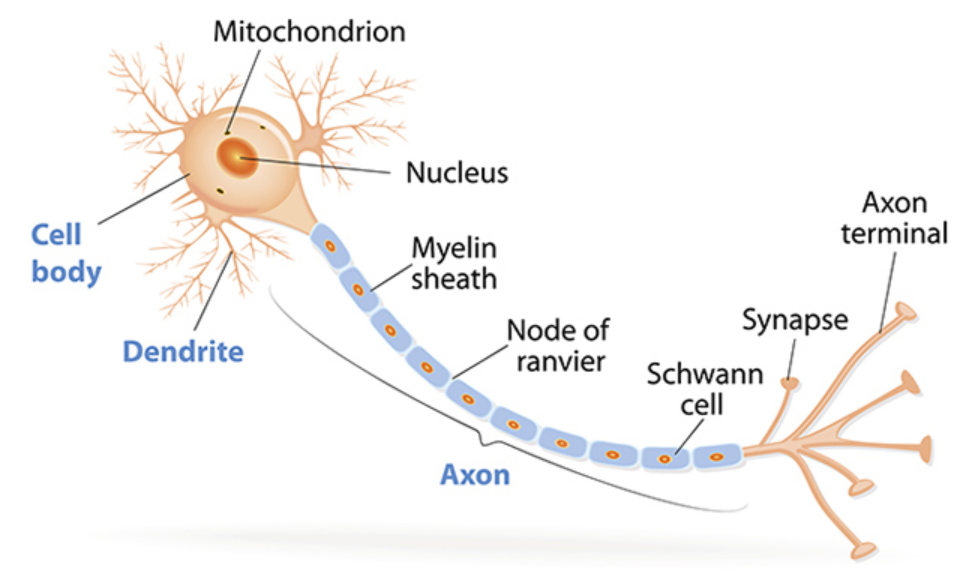
This work is focused on the modelling of signal propagations in myelinated axons to characterize the functions of the myelin sheath in the neural structure. Based on reasonable assumptions on the medium properties, we derive a two-dimensional neural-signaling model in cylindrical coordinates from the time-harmonic Maxwell's equations. The well-posedness of model is established upon Dirichlet boundary conditions at the two ends of the neural structure and the radiative condition in the radial direction of the structure. Using the perfectly matched layer (PML) method, we truncate the unbounded background medium and propose an approximate problem on the truncated domain. The well-posedness of the PML problem and the exponential convergence of the approximate solution to the exact solution are established. Numerical experiments based on finite element discretization are presented to demonstrate the theoretical results and the efficiency of our methods to simulate the signal propagation in axons.
翻译:这项工作的重点是模拟近离轴中的信号传播,以描述神经结构中弥散层的功能。根据对介质特性的合理假设,我们从时间-和谐Maxwell的方程式的圆柱形坐标中得出一个二维神经信号信号信号模型。模型的精度是在神经结构两端Drichlet边界条件和结构放射方向的辐射条件上确定的。我们使用与极匹配的层(PML)方法,对无线背景介质进行截断,并提出疏漏域的近似问题。确定了PML问题的准确位置和接近解决方案与确切解决方案的指数趋同。根据有限元素离散进行的数字实验是为了展示模拟轴中信号传播的理论结果和效率。