On the bottleneck stability of rank decompositions of multi-parameter persistence modules

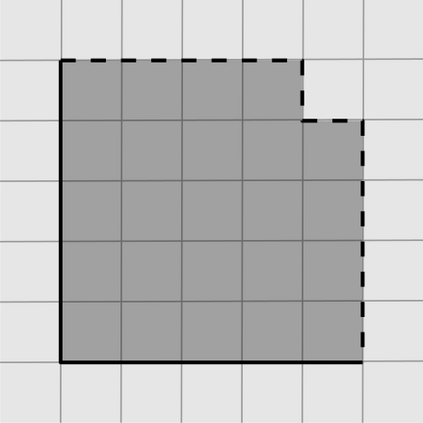
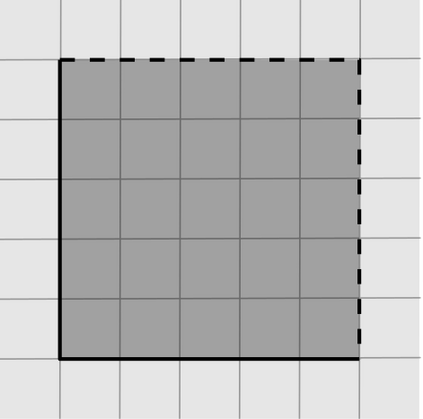
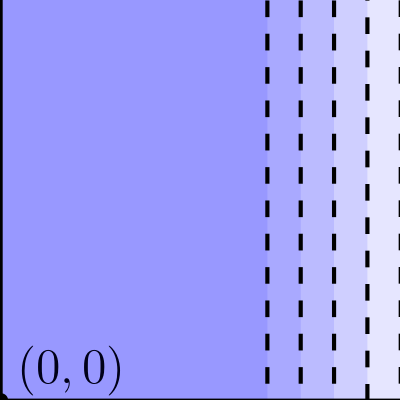
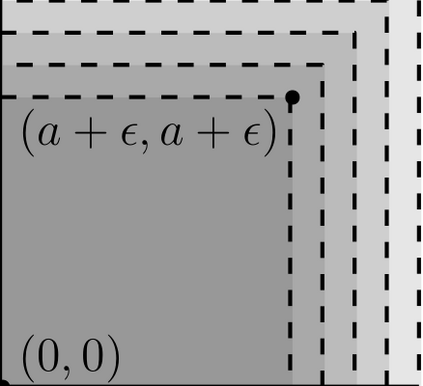
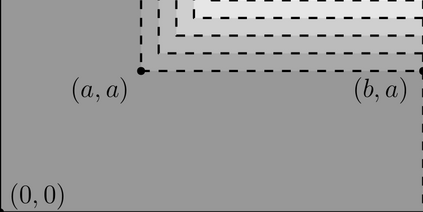
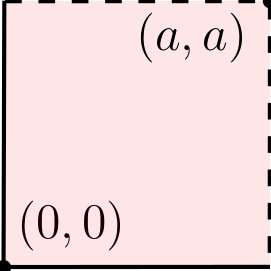
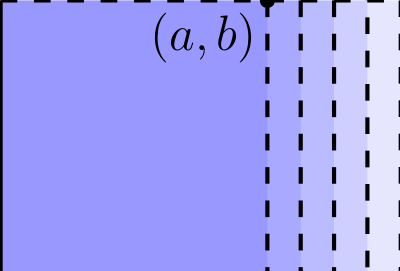
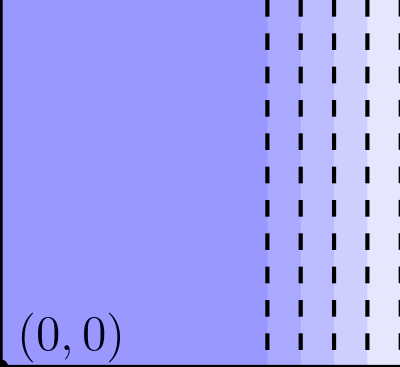
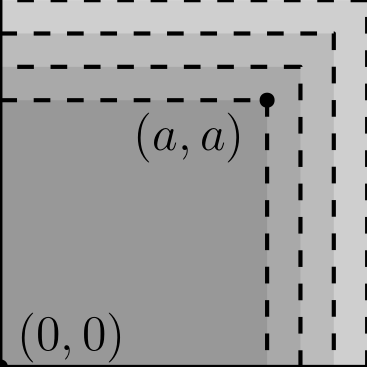
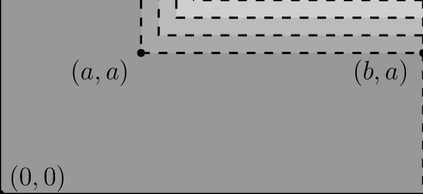
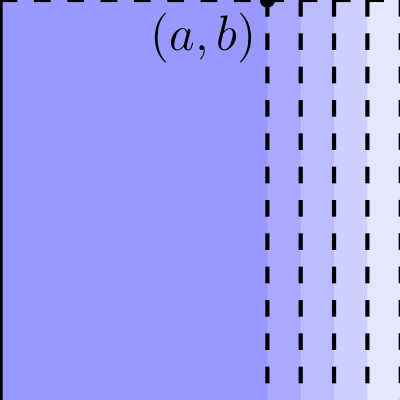
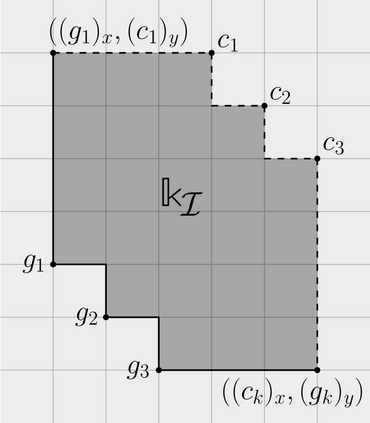
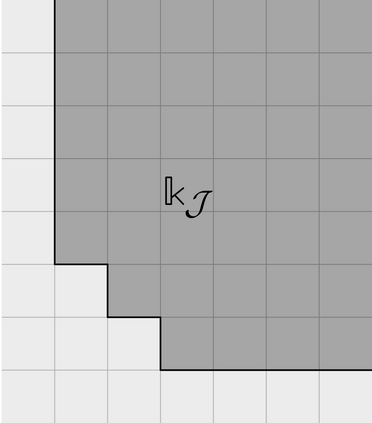
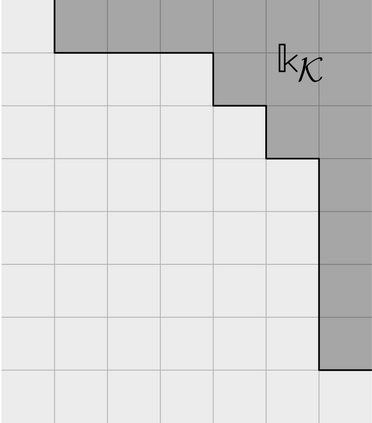
A significant part of modern topological data analysis is concerned with the design and study of algebraic invariants of poset representations -- often referred to as multi-parameter persistence modules. One such invariant is the minimal rank decomposition, which encodes the ranks of all the structure morphisms of the persistence module by a single ordered pair of rectangle-decomposable modules, interpreted as a signed barcode. This signed barcode generalizes the concept of persistence barcode from one-parameter persistence to any number of parameters, raising the question of its bottleneck stability. We show in this paper that the minimal rank decomposition is not stable under the natural notion of signed bottleneck matching between signed barcodes. We remedy this by turning our focus to the rank exact decomposition, a related signed barcode induced by the minimal projective resolution of the module relative to the so-called rank exact structure, which we prove to be bottleneck stable under signed matchings. As part of our proof, we obtain two intermediate results of independent interest: we compute the global dimension of the rank exact structure on the category of finitely presentable multi-parameter persistence modules, and we prove a bottleneck stability result for hook-decomposable modules. We also give a bound for the size of the rank exact decomposition that is polynomial in the size of the usual minimal projective resolution, we prove a universality result for the dissimilarity function induced by the notion of signed matching, and we compute, in the two-parameter case, the global dimension of a different exact structure related to the upsets of the indexing poset. This set of results combines concepts from topological data analysis and from the representation theory of posets, and we believe is relevant to both areas.