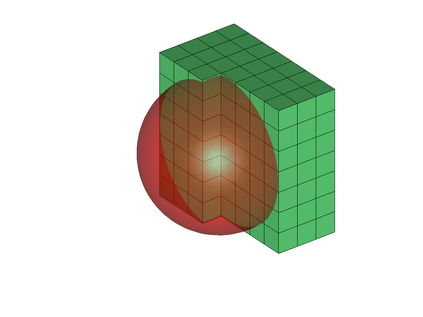
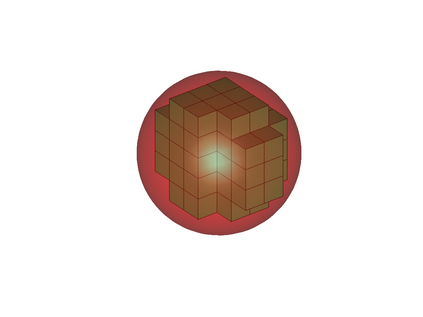
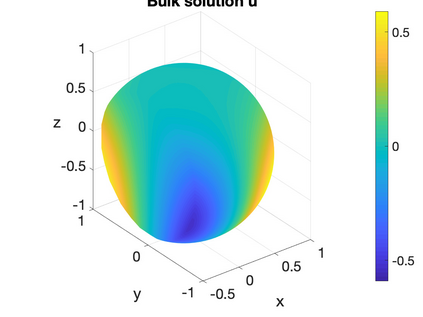
In this work we present a novel bulk-surface virtual element method (BSVEM) for the numerical approximation of elliptic bulk-surface partial differential equations (BSPDEs) in three space dimensions. The BSVEM is based on the discretisation of the bulk domain into polyhedral elements with arbitrarily many faces. The polyhedral approximation of the bulk induces a polygonal approximation of the surface. Firstly, we present a geometric error analysis of bulk-surface polyhedral meshes independent of the numerical method. Hence, we show that BSVEM has optimal second-order convergence in space, provided the exact solution is $H^{2+3/4}$ in the bulk and $H^2$ on the surface, where the additional $\frac{3}{4}$ is due to the combined effect of surface curvature and polyhedral elements close to the boundary. We show that general polyhedra can be exploited to reduce the computational time of the matrix assembly. To support our convergence results, a numerical example is presented which demonstrates optimal convergence of an elliptic BSPDE in three space dimensions.
翻译:在这项工作中,我们为三个空间维度的椭圆散表面部分偏差方程式(BSPDEs)的数字近似值提出了一个新的散状表面虚拟要素方法(BSVEM)。BSVEM的基础是将散列域分解成多面的多元元素。散列的多面近似形形形形形形形形色色形形形形形形色色形形形形形形色色色形形形形色色色色色色色色色色色色色色色色色色微微微色色色色色色色色色色色色色色色色色色色色色色色的几何性分析。因此,我们证明BSVEM在空间有最佳的第二阶趋近点趋近点,只要准确的解决方案在散数上是$H2+3/4}美元和在表面的$H2$2美元色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色微微微微微微微微微微微微微微微微微微微色色色色色色色色色色色色微微微微微微微微微微微微微微微微微微微微微微色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色色微色微色色色微色微色微微微微微微微微微微微微微微色微色微色微色微色微色微色微色微色微微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色微色