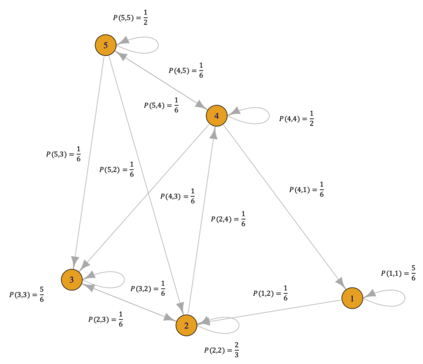
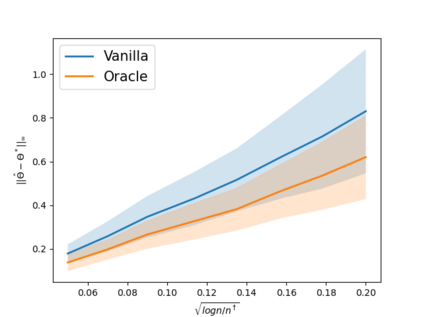
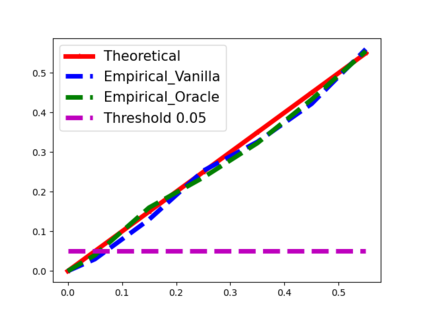
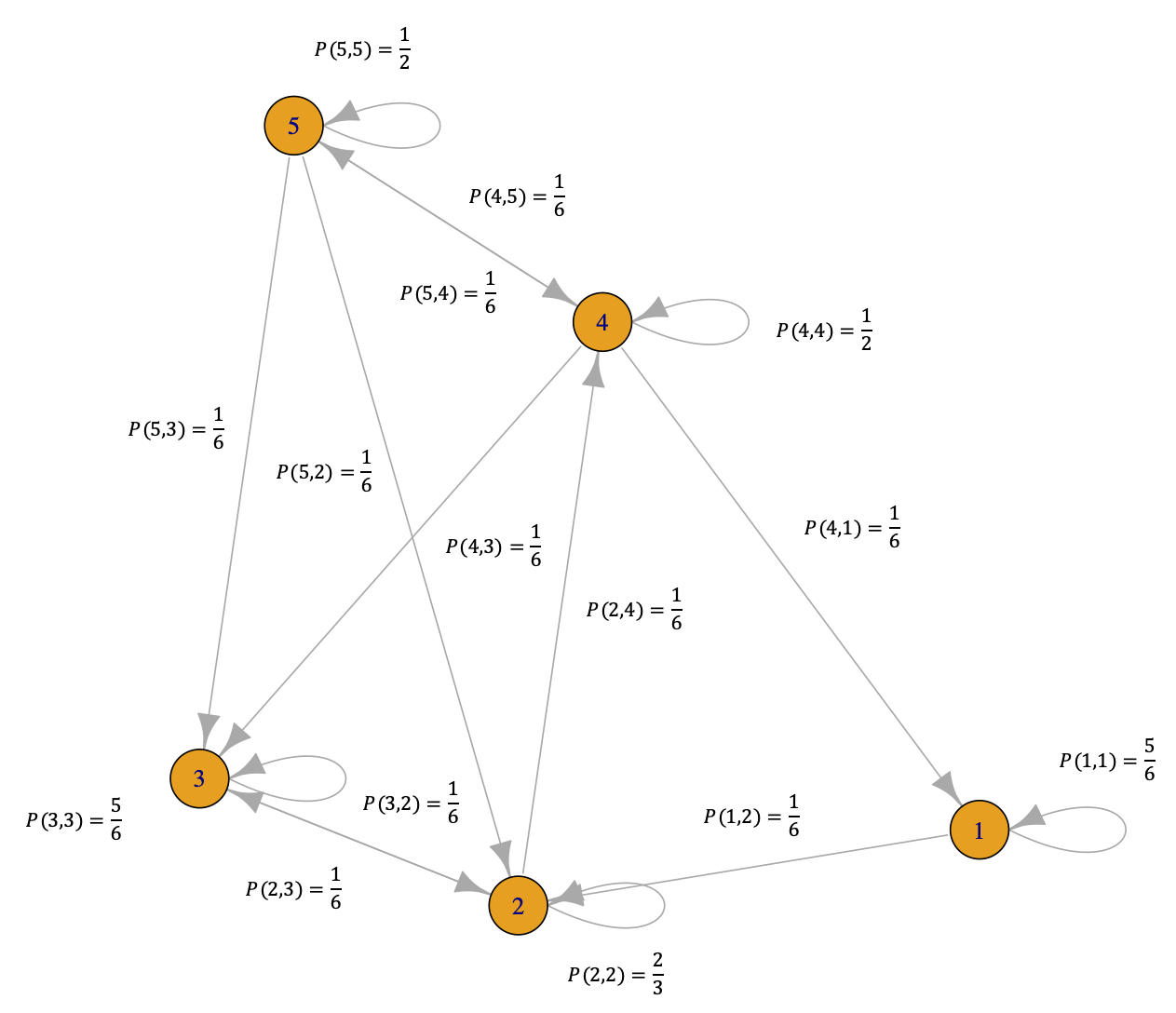
This paper studies the performance of the spectral method in the estimation and uncertainty quantification of the unobserved preference scores of compared entities in a very general and more realistic setup in which the comparison graph consists of hyper-edges of possible heterogeneous sizes and the number of comparisons can be as low as one for a given hyper-edge. Such a setting is pervasive in real applications, circumventing the need to specify the graph randomness and the restrictive homogeneous sampling assumption imposed in the commonly-used Bradley-Terry-Luce (BTL) or Plackett-Luce (PL) models. Furthermore, in the scenarios when the BTL or PL models are appropriate, we unravel the relationship between the spectral estimator and the Maximum Likelihood Estimator (MLE). We discover that a two-step spectral method, where we apply the optimal weighting estimated from the equal weighting vanilla spectral method, can achieve the same asymptotic efficiency as the MLE. Given the asymptotic distributions of the estimated preference scores, we also introduce a comprehensive framework to carry out both one-sample and two-sample ranking inferences, applicable to both fixed and random graph settings. It is noteworthy that it is the first time effective two-sample rank testing methods are proposed. Finally, we substantiate our findings via comprehensive numerical simulations and subsequently apply our developed methodologies to perform statistical inferences on statistics journals and movie rankings.
翻译:暂无翻译