A General approach to Ammann bars for aperiodic tilings

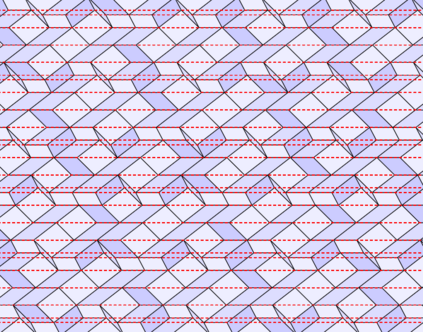
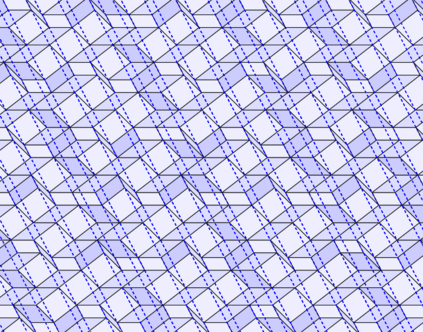
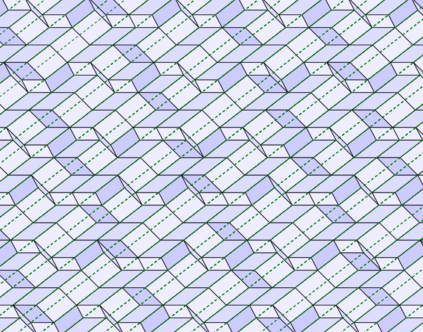
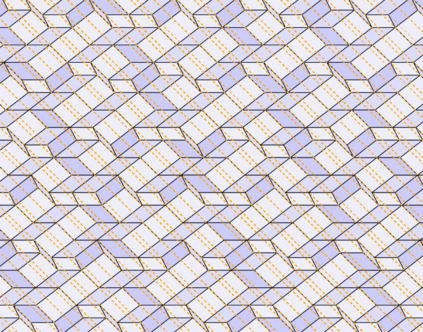
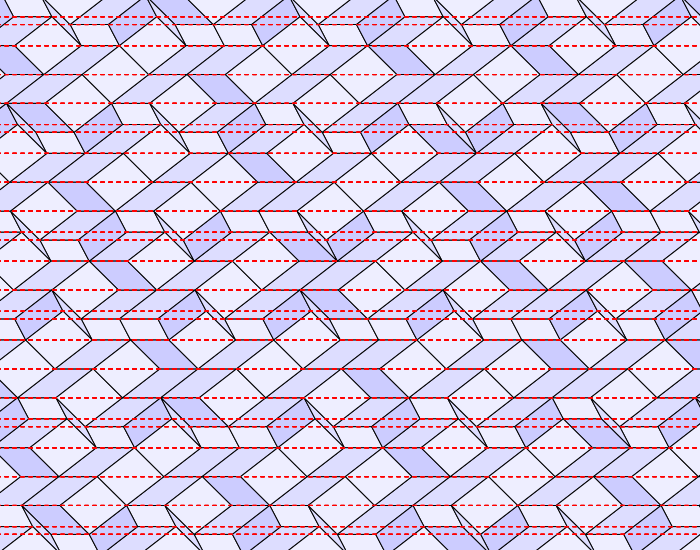
Ammann bars are formed by segments (decorations) on the tiles of a tiling such that forming straight lines with them while tiling forces non-periodicity. Only a few cases are known, starting with Robert Ammann's observations on Penrose tiles, but there is no general explanation or construction. In this article we propose a general method for cut and project tilings based on the notion of \emph{subperiods} and we illustrate it with an aperiodic set of 36 decorated prototiles related to what we called \emph{Cyrenaic tilings}.