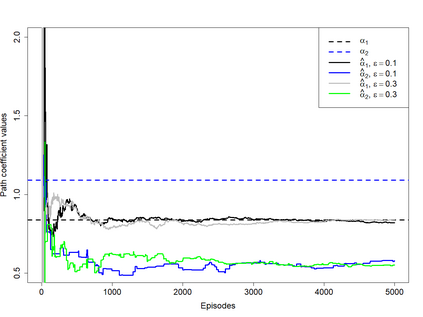
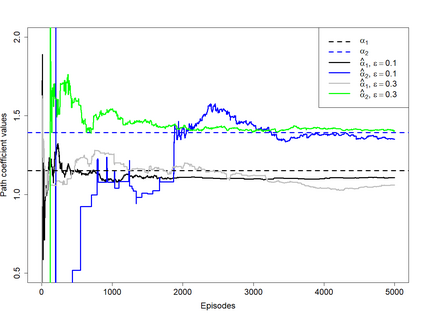
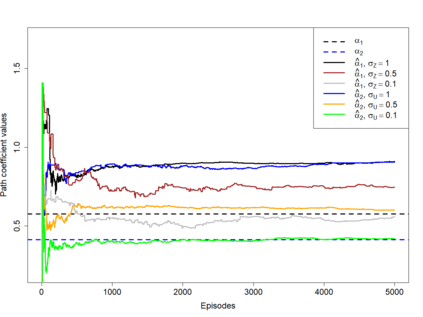
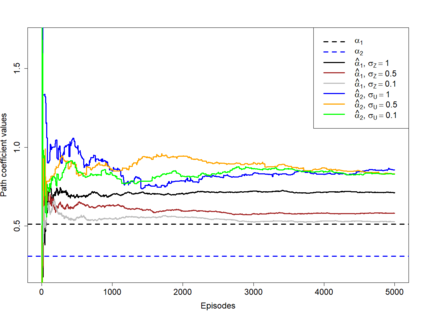
We extend path analysis by showing that, for a singly-connected path diagram, the partial covariance of two random variables factorizes over the nodes and edges in the path between the variables. This result allows us to determine the contribution of each node and edge to the partial covariance. It also allows us to show that Simpson's paradox cannot occur in singly-connected path diagrams.
翻译:我们扩展路径分析, 显示对于单一连接路径图来说, 两个随机变量的局部共变系数 会在两个变量之间的节点和边缘上产生。 这个结果让我们可以确定每个节点和边缘对部分共变的贡献。 它也让我们能够显示辛普森的悖论不能出现在单连接路径图中 。
相关内容
专知会员服务
78+阅读 · 2022年3月15日
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
0+阅读 · 2022年8月15日
Arxiv
0+阅读 · 2022年8月15日