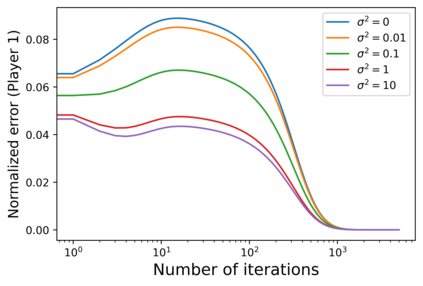
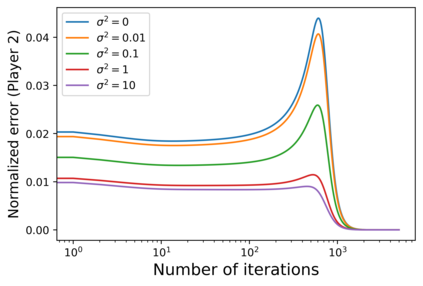
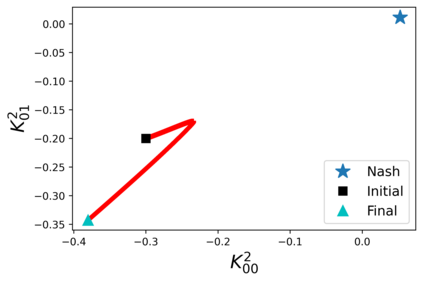
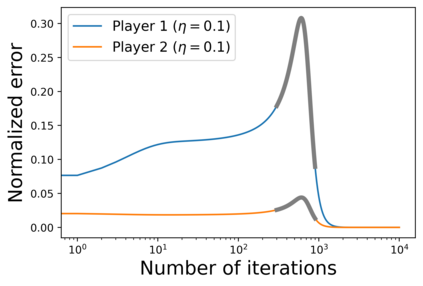
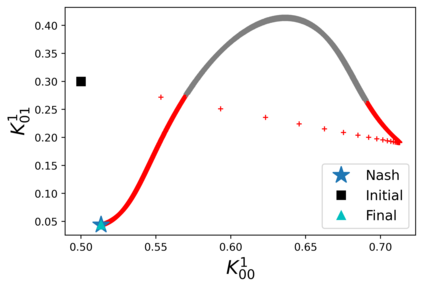
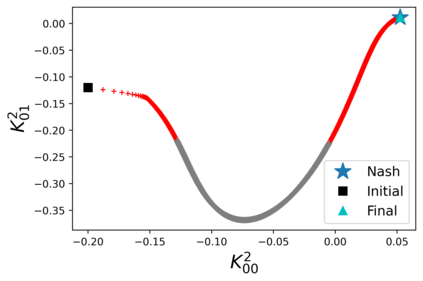
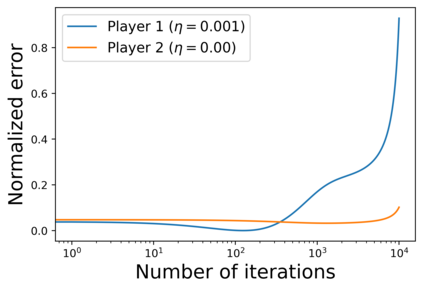
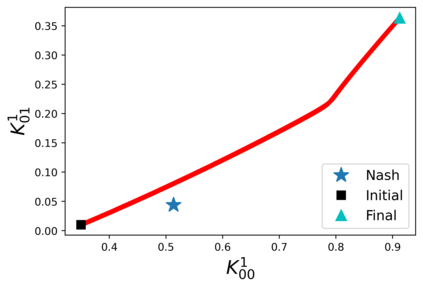
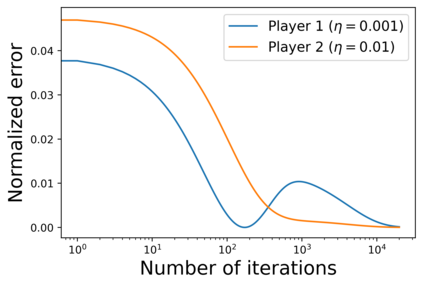
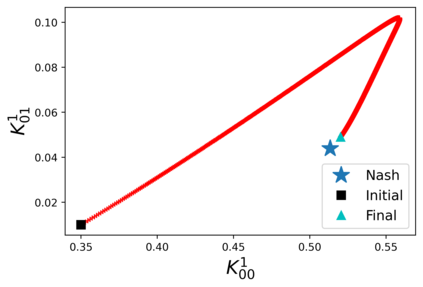
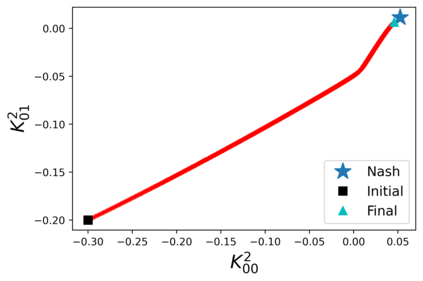
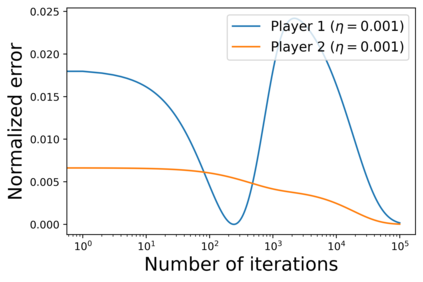
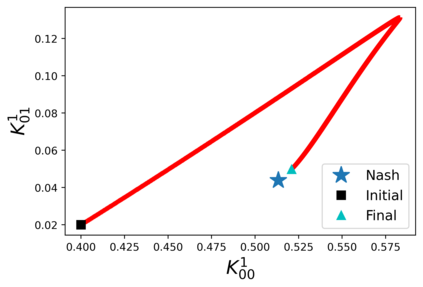
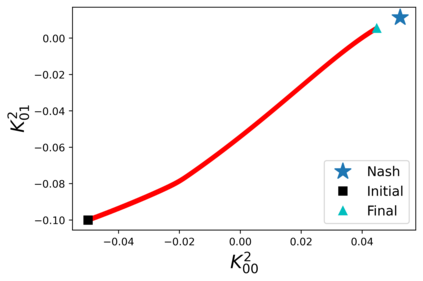
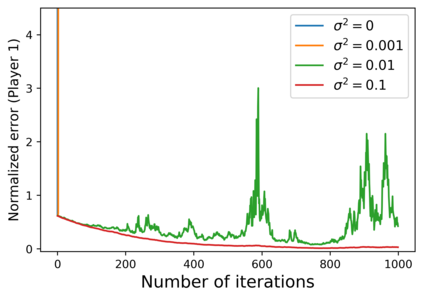
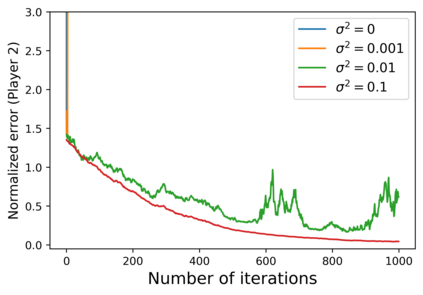
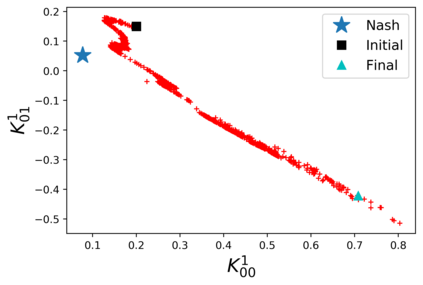
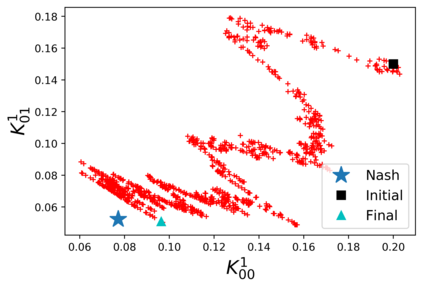
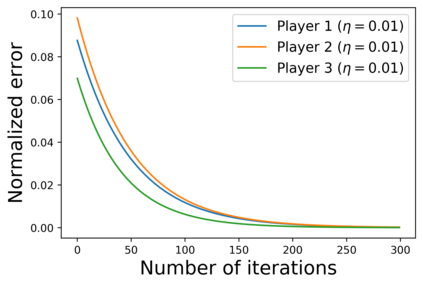
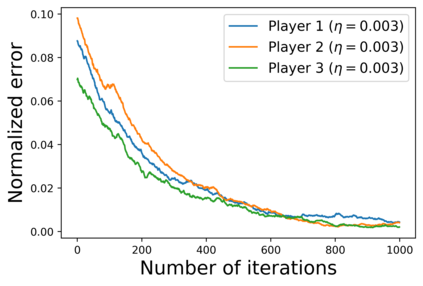
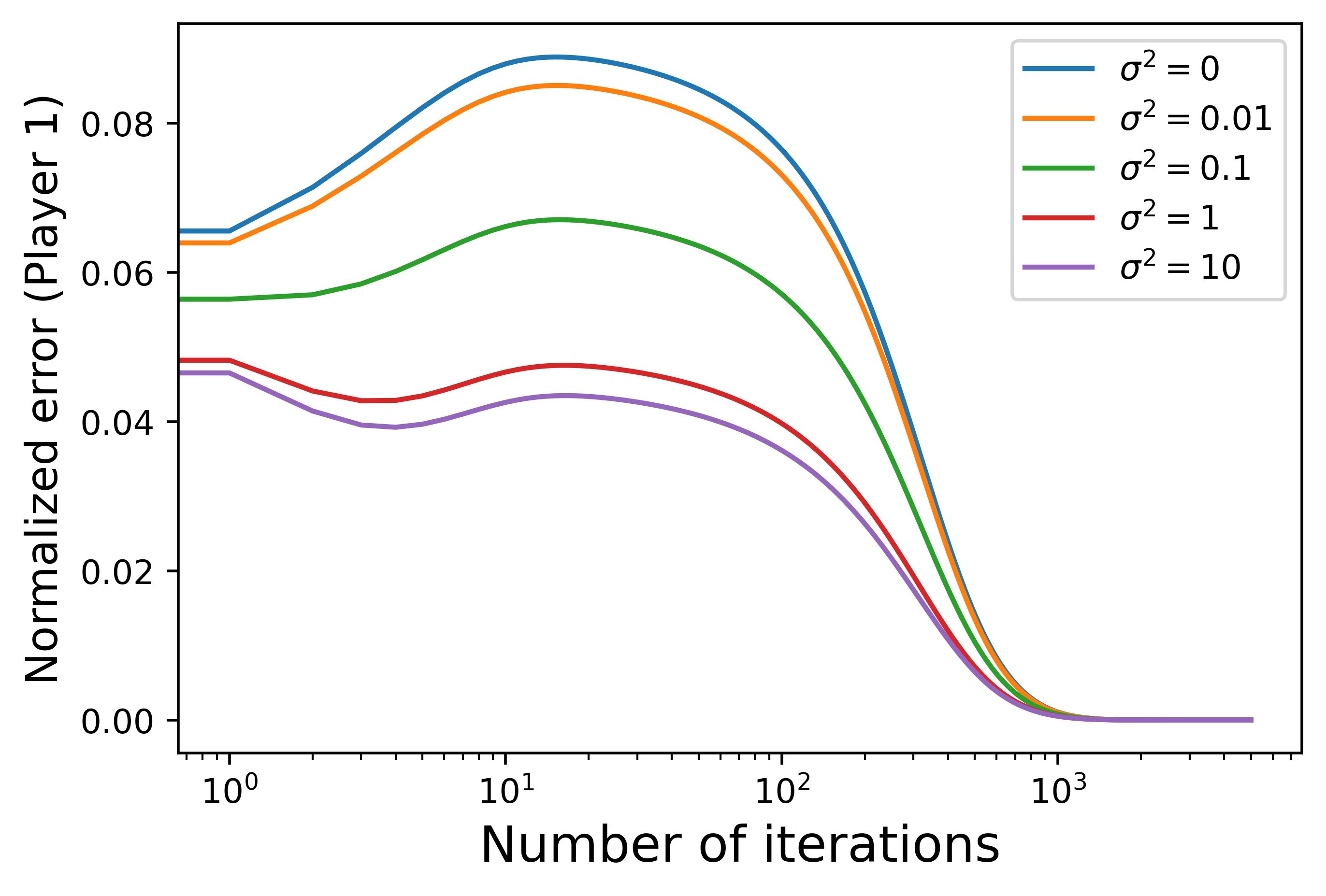
We consider a general-sum N-player linear-quadratic game with stochastic dynamics over a finite horizon and prove the global convergence of the natural policy gradient method to the Nash equilibrium. In order to prove the convergence of the method, we require a certain amount of noise in the system. We give a condition, essentially a lower bound on the covariance of the noise in terms of the model parameters, in order to guarantee convergence. We illustrate our results with numerical experiments to show that even in situations where the policy gradient method may not converge in the deterministic setting, the addition of noise leads to convergence.
翻译:我们认为N球员线性赤道游戏是一个总和,在一定的地平线上具有随机动态,并证明自然政策梯度方法与纳什平衡的全球趋同。为了证明该方法的趋同,我们需要在系统中有一定的噪音。我们给出了一个条件,基本上在模型参数方面对噪音的共变程度有一个较低的约束,以保证趋同。我们用数字实验来说明我们的结果,以表明即使在政策梯度方法可能无法在确定性环境下趋同的情况下,增加噪音也会导致趋同。