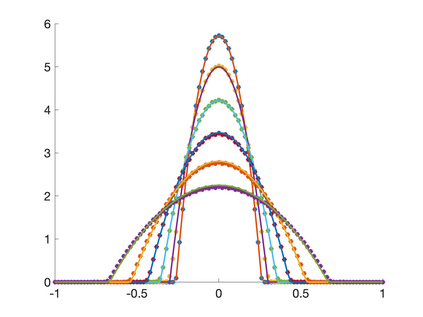
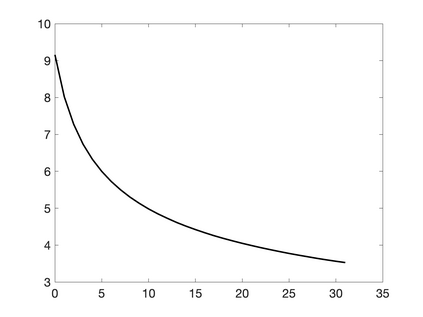
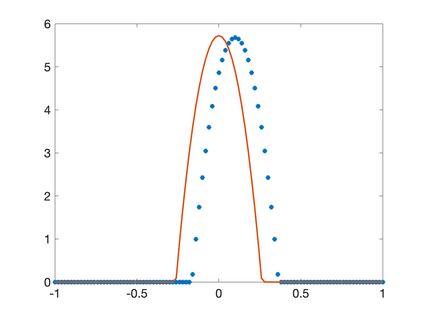
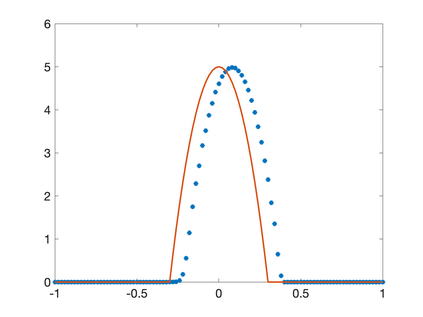
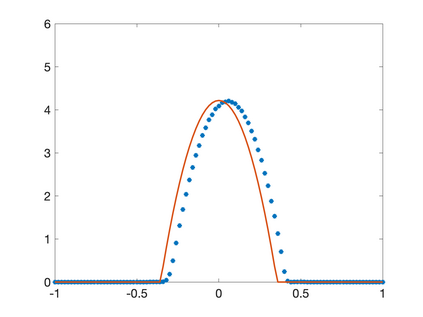
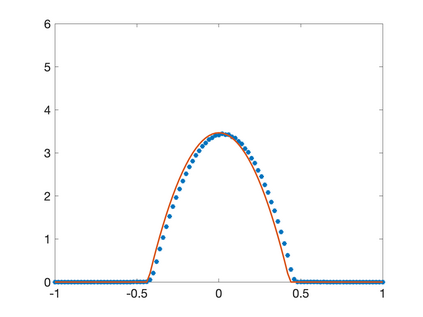
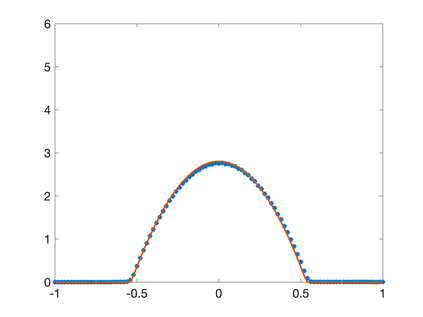
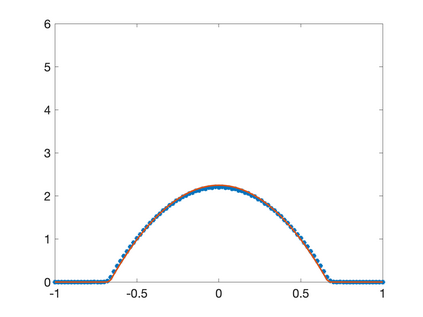
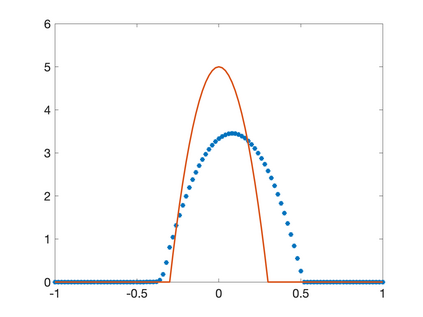
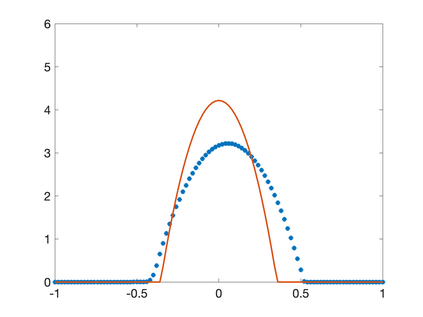
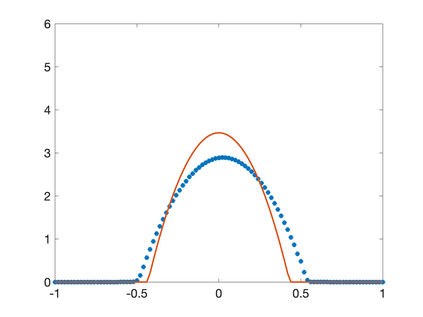
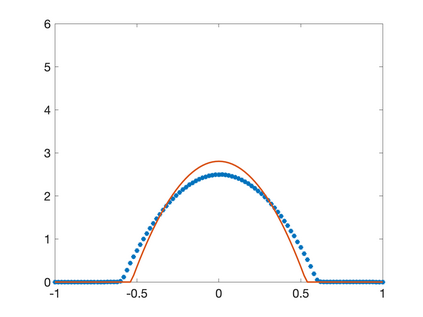
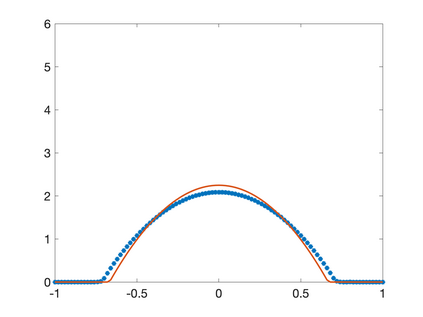
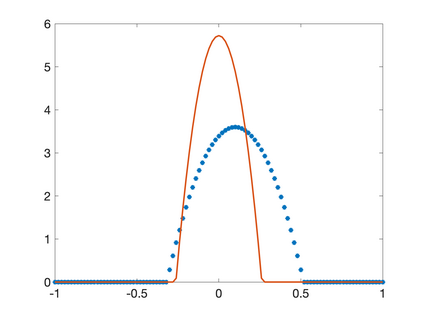
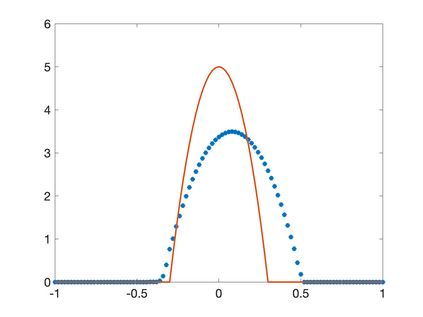
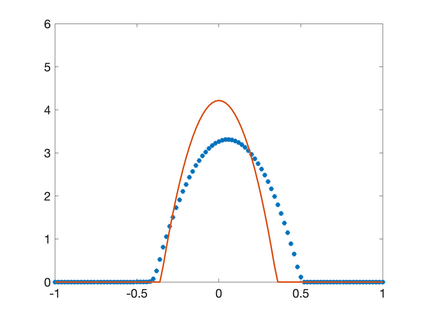
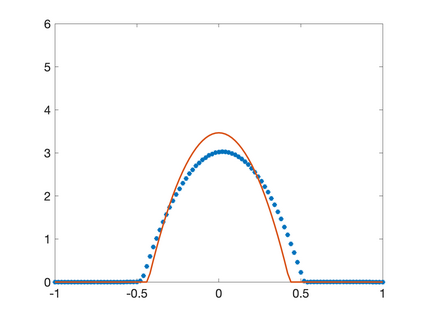
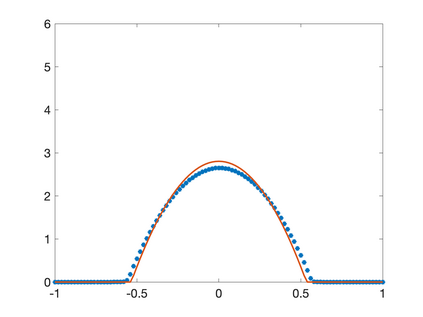
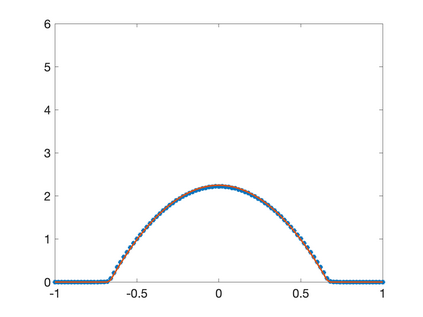
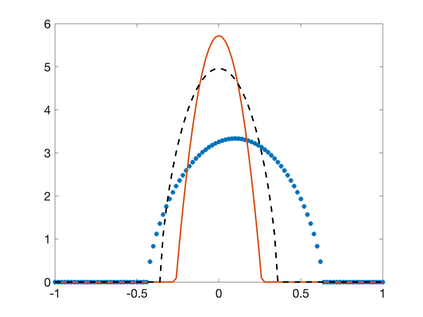
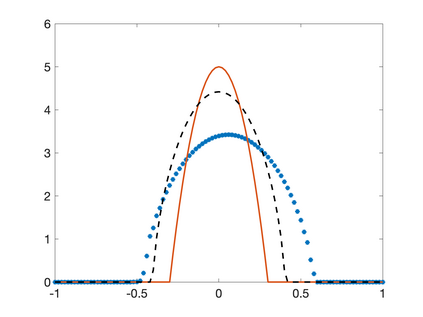
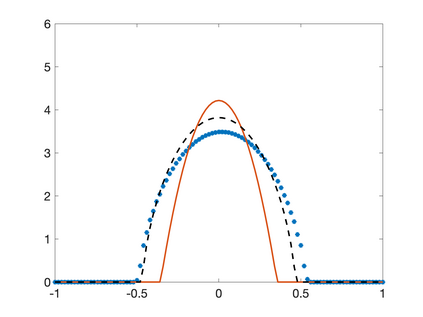
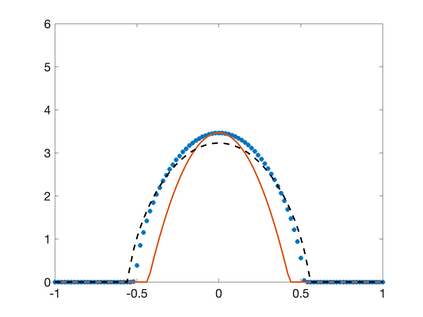
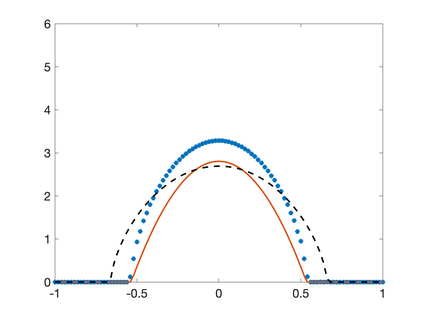
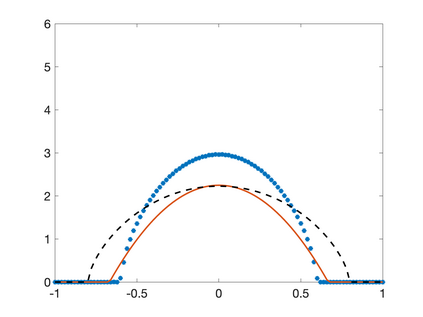
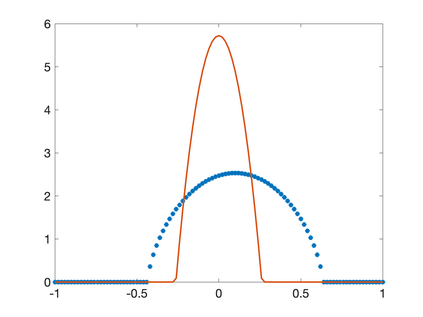
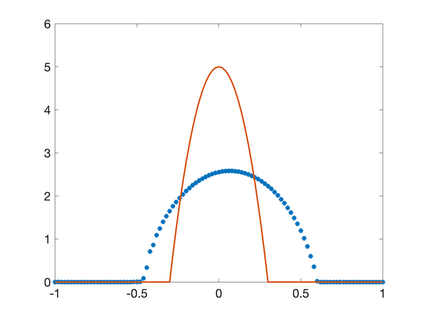
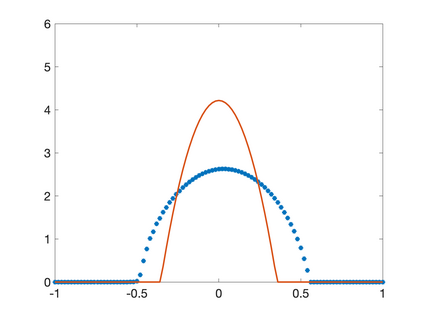
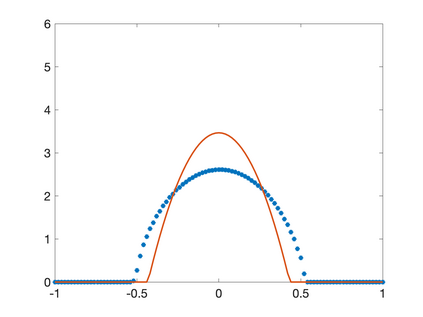
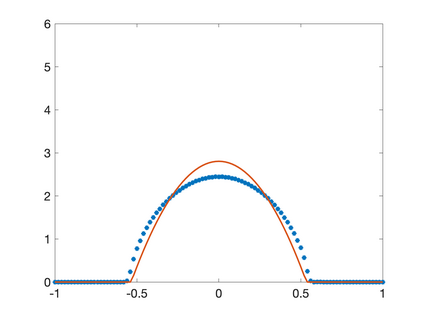
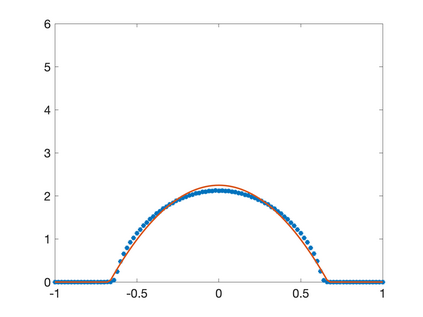
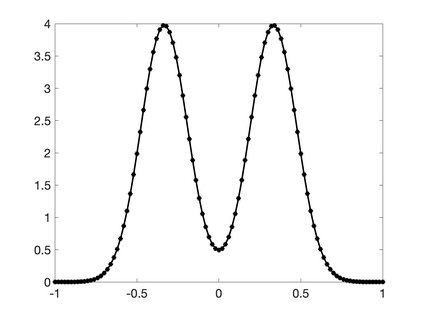
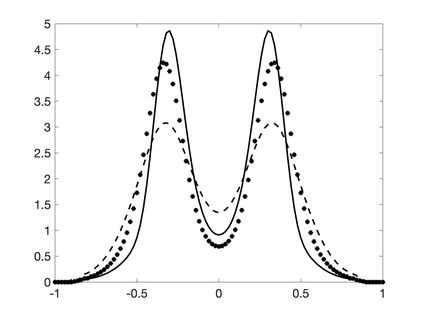
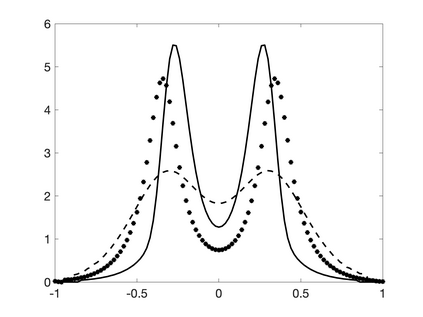
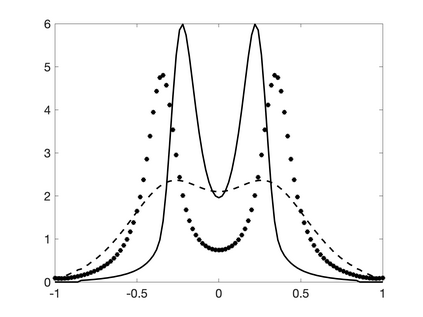
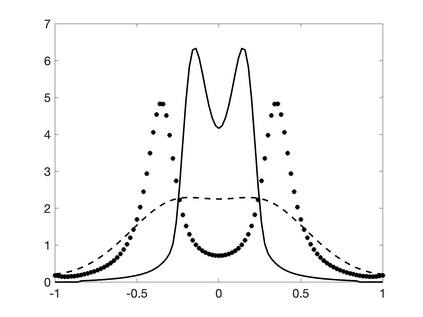
We present a framework enabling variational data assimilation for gradient flows in general metric spaces, based on the minimizing movement (or Jordan-Kinderlehrer-Otto) approximation scheme. After discussing stability properties in the most general case, we specialise to the space of probability measures endowed with the Wasserstein distance. This setting covers many non-linear partial differential equations (PDEs), such as the porous medium equation or general drift-diffusion-aggregation equations, which can be treated by our methods independent of their respective properties (such as finite speed of propagation or blow-up). We then focus on the numerical implementation of our approach using an primal-dual algorithm. The strength of our approach lies in the fact that by simply changing the driving functional, a wide range of PDEs can be treated without the need to adopt the numerical scheme. We conclude by presenting detailed numerical examples.
翻译:我们根据尽量减少运动(或约旦-Kinderle Heir-Ottto)近似法,提出了一个框架,使一般计量空间梯度流的变异数据同化。在讨论最一般情况下的稳定特性之后,我们专门研究瓦塞斯坦距离的概率测量空间。这一设置涵盖许多非线性部分方程式,如多孔介质方程式或一般漂移-扩散-聚合方程式,这些方程式可以不受各自的特性(例如有限的传播速度或爆炸速度)处理。然后我们侧重于我们方法的量化实施,使用原始的双算法。我们的方法的优点在于,通过简单地改变驱动功能,可以处理广泛的PDE方程式,而不必采用数字法。我们最后提出详细的数字示例。