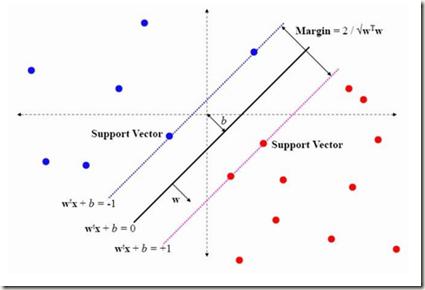
We address the issue of binary classification in Banach spaces in presence of uncertainty. We show that a number of results from classical support vector machines theory can be appropriately generalised to their robust counterpart in Banach spaces. These include the Representer Theorem, strong duality for the associated Optimization problem as well as their geometric interpretation. Furthermore, we propose a game theoretic interpretation by expressing a Nash equilibrium problem formulation for the more general problem of finding the closest points in two closed convex sets when the underlying space is reflexive and smooth.
翻译:我们在不确定的情况下处理Banach空间二元分类问题,我们指出,传统支持矢量机器理论的一些结果可以适当地推广到Banach空间的强力对应方,包括代表理论、相关最佳化问题的强烈双重性及其几何解释。 此外,我们提出一种游戏理论解释,表达纳什平衡问题提法,以解决在基础空间具有反射性和平稳性时在两套封闭式软盘中找到最接近点这一更为普遍的问题。