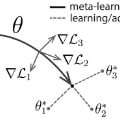
The main objective of this research paper is to investigate the local convergence characteristics of Model-agnostic Meta-learning (MAML) when applied to linear system quadratic optimal control (LQR). MAML and its variations have become popular techniques for quickly adapting to new tasks by leveraging previous learning knowledge in areas like regression, classification, and reinforcement learning. However, its theoretical guarantees remain unknown due to non-convexity and its structure, making it even more challenging to ensure stability in the dynamic system setting. This study focuses on exploring MAML in the LQR setting, providing its local convergence guarantees while maintaining the stability of the dynamical system. The paper also presents simple numerical results to demonstrate the convergence properties of MAML in LQR tasks.
翻译:暂无翻译