A non-parametric approach to detect patterns in binary sequences

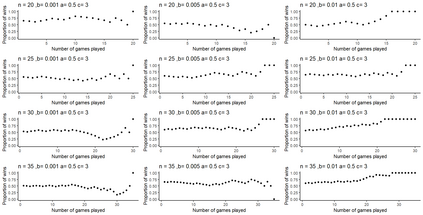
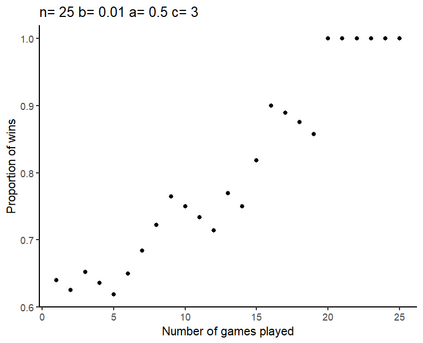
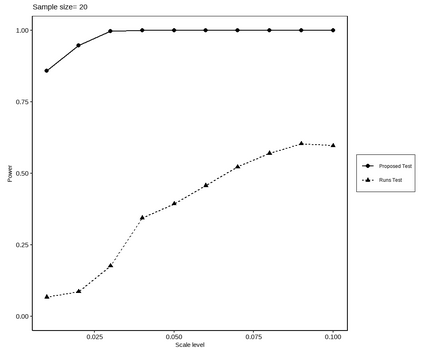
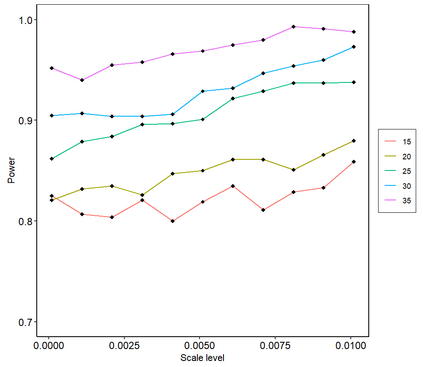
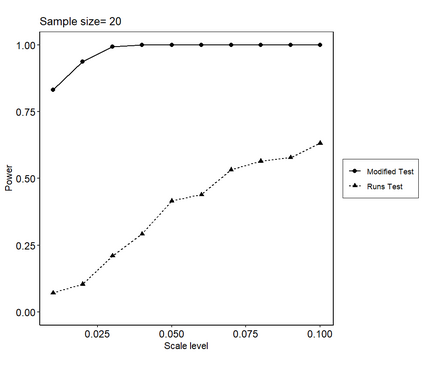
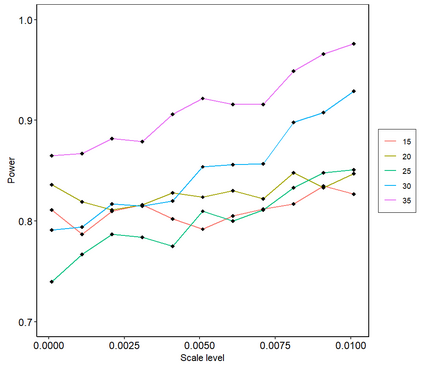
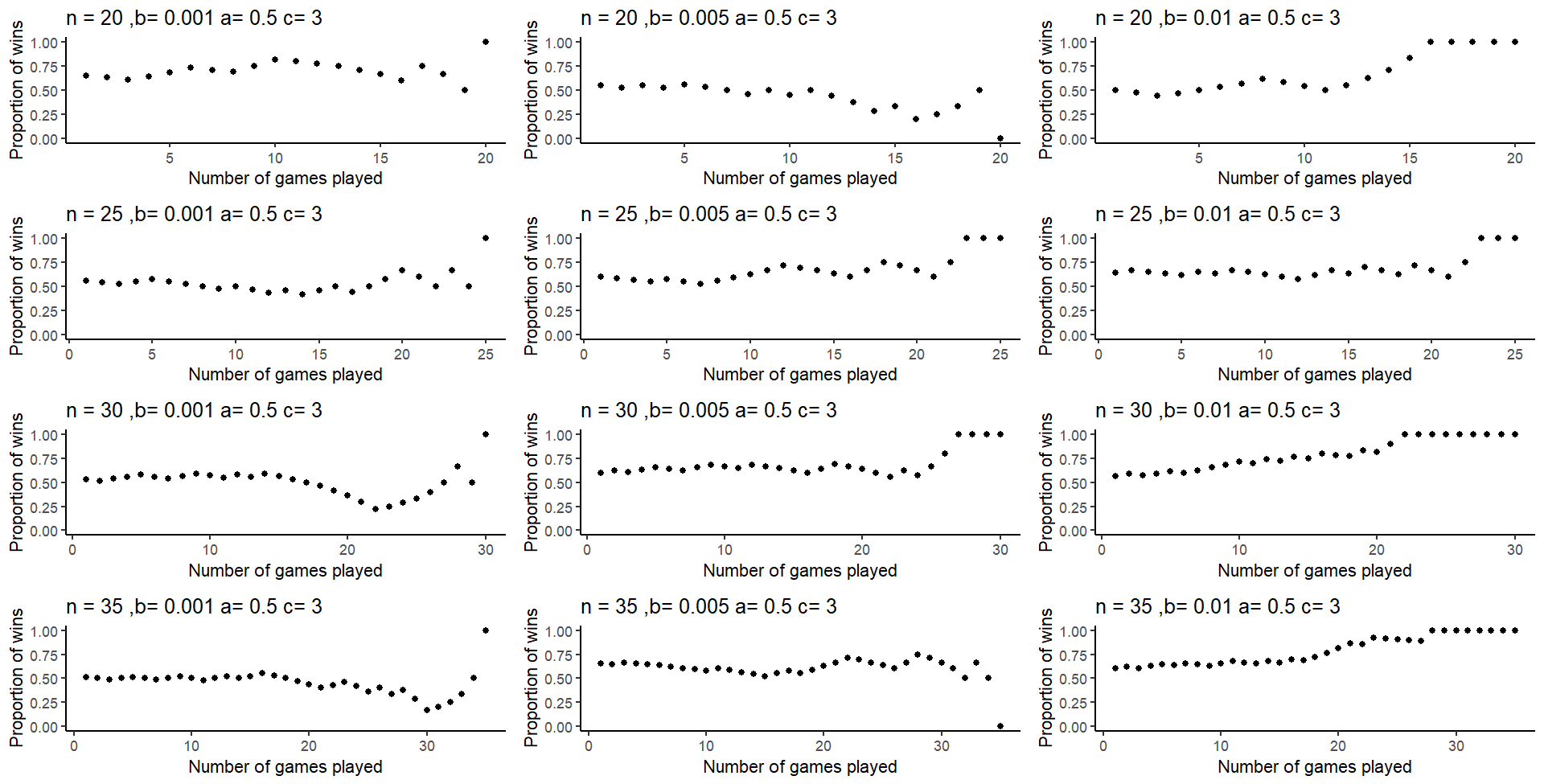
To determine any pattern in an ordered binary sequence of wins and losses of a player over a period of time, the Runs Test may show results contradictory to the intuition visualised by scatter plots of win proportions over time. We design a test suitable for this purpose by computing the gaps between two consecutive wins and then using exact binomial tests and non-parametric tests like Kendall's Tau and Siegel-Tukey's test for scale problem for determination of heteroscedastic patterns and direction of the occurrence of wins. Further modifications suggested by Jan Vegelius(1982) have been applied in the Siegel Tukey test to adjust for tied ranks.