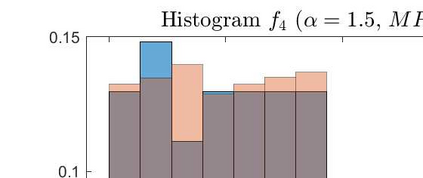
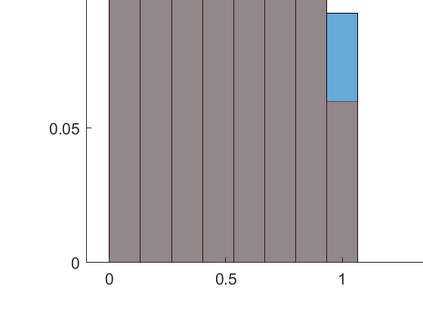
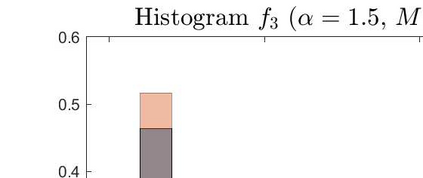
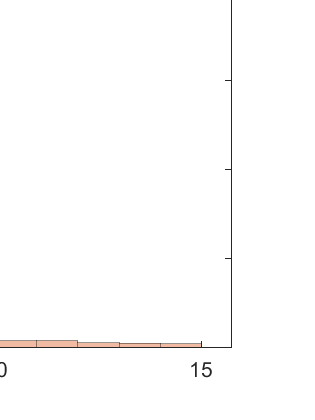
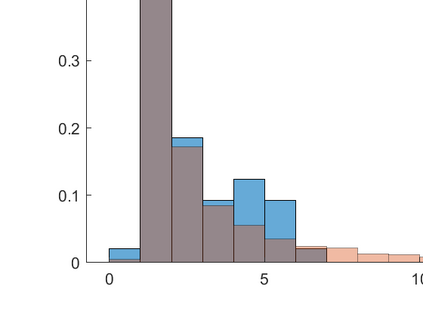
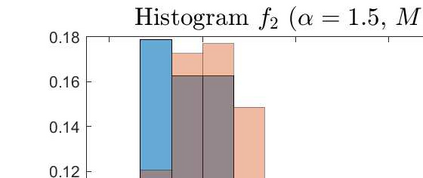
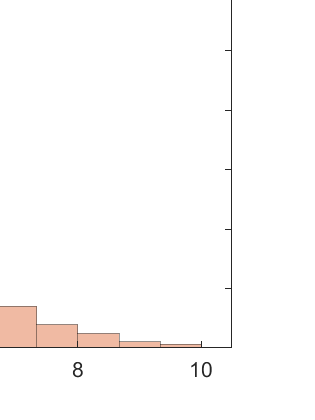
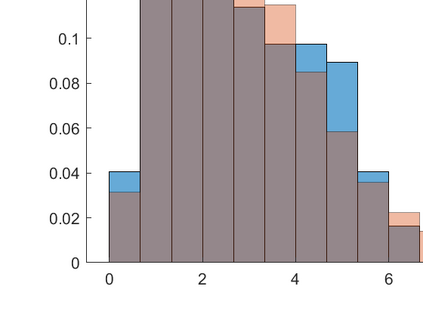
We consider stationary real harmonizable symmetric $\alpha$-stable processes $X=\left\{X(t):t\in\mathbb{R}\right\}$ with a finite control measure. Assuming the control measure is symmetric and absolutely continuous with respect to the Lebesgue measure on the real line, we refer to its density function as the spectral density of $X$. Standard methods for statistical inference on stable processes cannot be applied as harmonizable stable processes are non-ergodic. A stationary real harmonizable symmetric $\alpha$-stable process $X$ admits a LePage series representation and is conditionally Gaussian which allows us to derive the non-ergodic limit of sample functions on $X$. In particular, we give an explicit expression for the non-ergodic limits of the empirical characteristic function of $X$ and the lag process $\left\{X(t+h)-X(t):t\in\mathbb{R}\right\}$ with $h>0$, respectively. The process admits an equivalent representation as a series of sinusoidal waves with random frequencies whose probability density function is in fact the (normalized) spectral density of $X$. Based on strongly consistent frequency estimation using the periodogram we present a strongly consistent estimator of the spectral density. The periodogram's computation is fast and efficient, and our method is not affected by the non-ergodicity of $X$. Most notably no prior knowledge on parameters of the process such as its index of stability $\alpha$ is needed.
翻译:我们认为固定的、真实的、可以对称的对称 $ ALpha$- sable 进程 $X : t\ in\ mathb{R ⁇ right}$, 带有有限的控制度。 假设控制措施对实行的Lebesgue 测量值是对称的和绝对的连续的, 我们将其密度函数称为光谱密度。 稳定流程的统计推断标准方法无法应用, 因为可对称的稳定流程是非对称的。 一个固定的、真实的、可以对称的对称 $+X : $x : 一个固定的对称 $\\ alpha$_ 美元- sable 美元- sable 美元 的对称 levelPage 系列代表了LePage 系列的表示, 允许我们以$X 为单位的样本功能的对非对调值限制。 特别是, 美元的经验特征功能是 美元xxx 和直径直径的直径的直径直径的直径直径的直径直径直径直径比值 。